Functions could be considered one of the most important topics in high school mathematics due to their importance and relevance to a number of other mathematical topics and their foundational role in college-level mathematics and other related areas in the sciences (e.g., Cooney, Beckmann, & Lloyd, 2010; Dubinsky & Harel, 1992; Leinhardt, Zaslavsky, & Stein, 1990). Further, they are a critical base for mathematical understanding of science, technology, engineering, and mathematics (STEM) disciplines and are often regarded as the unifying element of much of secondary mathematics.
Students begin studying informal function concepts as early as third grade, then they become a dominant feature throughout most high school courses. In the Common Core State Standards for Mathematics (CCSSM) the study of functions is given its own domain, separate from Algebra, in grades 9–12 (National Governors Association Center for Best Practice & Council of Chief State School Officers, 2010).
Putting functions front and center in high school mathematics is accompanied by many conceptual obstacles that have been well documented in the literature (e.g., Even, 1990; Tall, McGowen, & DeMarois, 2000). Research has revealed common misconceptions with respect to the definition of function (Vinner & Dreyfus, 1989), use of function notation (Oehrtman, Carlson, & Thompson, 2008), and connections between function representations (e.g., Brenner, 1998; Clement, 2001; Dreher & Kuntze, 2015; Stylianou, 2011). Some of the same misconceptions have been identified in studies involving preservice and practicing secondary math teachers (Bannister, 2014; Chesler, 2012; Even, 1990, 1993; Kabael, 2011; Wilson, 1994). Due to the similarities among misconceptions held by K-16 students as well as teachers, preservice teachers need opportunities to examine their own knowledge of function and consider how to engage students in tasks that develop robust understandings of function.
Foundational to developing deep and nuanced understandings of functions as they are positioned throughout secondary mathematics is understanding the concept of function itself. This need is highlighted in the recently published National Council of Teachers of Mathematics (NCTM) book, Developing Essential Understandings of Functions, Grades 9-12 (Cooney et al., 2010), in which the authors identified five “big ideas” in regard to function – the first of which is the function concept. Related to this big idea, the authors identified essential understandings that high school teachers must have and explicitly address when teaching. The following are the essential understandings presented for the function concept:
- Functions are single-valued mappings from one set – the domain of the function – to another (its range).
- Functions apply to a wide range of situations. They do not have to be described by any specific expression.
- The domain and range of functions do not have to be numbers. (p. 8)
The authors suggested that attending to the development of the essential understandings is imperative: “The importance of understanding functions and the challenge of understanding them make them essential for teachers of mathematics in grades 9-12 to understand extremely well themselves” (Cooney et al., 2010, p. 1).
In response to the call for opportunities for preservice teachers (PSTs) to deepen their knowledge of function, we designed and studied the implementation of an applet-based learning intervention focused on disrupting PSTs’ current understanding of the function concept. The purpose of this study is to examine the ways in which an applet designed to challenge PSTs’ understanding of function does so, and determine whether or not engaging with the applet results in shifts in their sense making related to the functions and nonfunctions represented in the applet.
Background
Over the last 50 years an extensive body of research has focused on understanding of functions. The consensus in this body of work is that many students possess a weak understanding of the concept of function, and research has documented several misconceptions that appear to be quite common.
Students’ Understandings of the Function Concept
Much of the research on students’ understandings of function has occurred in the context of college algebra, precalculus, or calculus classes. These studies have identified common understandings that students develop related to the concept of function. One common student understanding is that functions are defined by an algebraic formula (Breidenbach, Dubinsky, Hawks, & Nichols, 1992; Carlson, 1998; Clement, 2001; Sierpinska, 1992). This understanding is not surprising since functions are typically introduced as specific function types, such as linear and quadratic functions, in the middle school and high school curriculum (Cooney et al., 2010). Thompson (1994b) found that, not only do students view functions as algebraic formulas, some view functions as two expressions separated by an equal sign. While an equation view of function is not inherently wrong, it is narrow and can lead to difficulties for students as they work with functions in different contexts and with different representations (Cooney et al., 2010).
Along with an algebraic view of functions, research has shown that students often rely on the vertical line test to identify a function from a nonfunction (Breidenbach et al., 1992; Fernandez, 2005). This view can lead to conceptual difficulties in determining functions from nonfunctions, including the tendency to apply rules to determining functions from nonfunctions (Breidenbach et al., 1992; Fernandez, 2005). Students whose view of function is algebraic and uses procedural techniques to identify functions and nonfunctions struggle to comprehend a general mapping of input values to a set of output values (Carlson, 1998; Thompson 1994a).
When students consider particular function families, studies have shown that many exhibit difficulties identifying constant functions as functions (Bakar & Tall, 1991; Carlson, 1998; Rasmussen, 2000). This difficulty appears to stem from the fact that constant functions do not vary (Oehrtman et al., 2008).
In a study of undergraduates enrolled in a college algebra course, Carlson (1998) found that only 7% of students who earned a grade of A in the course (A-students) could produce a correct example of a function whose output values are the same regardless of input value. Carlson also posed this question to second semester calculus undergraduates, and 25% of A-students produced the example y = x.
Underlying the students’ problematic understandings about function is their definition of function. Yet, particular attention to the definition itself has not been widely researched (exceptions include Breidenbach et al., 1992, and Vinner & Dreyfus, 1989). In many studies student descriptions of functions are not tied directly to their definition of function. The consistency of problematic understandings of function found across studies speaks to the need for pedagogical practices to specifically disrupt and correct these understandings.
Teachers’ Understandings of the Function Concept
When considering the development of student understanding of function, teachers’ understandings are important as well. The majority of the research has focused on in-service teachers’ or PSTs’ descriptions of function, and similar themes to students’ understandings of functions have emerged (Even, 1990, 1993; Kaebel, 2011; Wilson, 1994). For example, similar to students, practicing teachers and PSTs tend to view functions algebraically and discuss properties of graphs (e.g., vertical line test) in their descriptions (Even, 1990, 1993; Wilson, 1994). One exception is a study by Vinner and Dreyfus (1989) who examined junior high teachers’ definitions of functions prior to a professional development. They found that 25 of 36 teachers provided a definition that pointed to a correspondence between two sets of elements.
More recent studies have reported similar findings, adding to the evidence that teachers hold some of the same misconceptions as K-16 students with respect to the definition of function and connecting representations (Bannister, 2014; Chesler, 2012). In particular, Chesler (2012) noted that many secondary mathathematics PSTs lack “flexibility and expertise in interpreting and using mathematical definitions” (p. 38) as they examined the equivalence of various definitions of function.
Teachers’ understanding of function has been shown to impact the pedagogical choices they make during instruction of the concept. For example, in her study of PSTs, Even (1993) found that PSTs could not justify the need for univalence (one-to-one) and did not know why it was important to distinguish between functions and nonfunctions. Because of this lack of content knowledge, the PSTs were limited in their pedagogical approaches, resulting in teaching students to procedurally identify functions using the vertical line test. Moreover, Bannister (2014) suggested that PSTs who are adept at translating between algebraic and graphical representations of functions may be better prepared to understand diverse student conceptions when they encounter them during instruction. Studies such as these point to the need to develop opportunities for teachers to not only examine their own understanding of function, but also analyze student thinking about functions in more productive ways.
Theoretical Framework
In considering PSTs’ learning related to function, we adopted a theoretical lens of transformation theory (Mezirow, 2009). Transformation theory is consistent with constructivist assumptions, specifically that meaning resides within each person and is constructed through experiences (Confrey, 1990). Mezirow (2009) described four forms of learning that lie at the heart of the theory: elaborating existing meaning schemes, learning new meaning schemes, transforming meaning schemes, and transforming meaning perspectives (p. 22). According to Peters (2014) meaning perspectives are the broad predispositions a person has toward a concept based on their prior experiences and culture. In contrast, meaning schemes, which are situated within meaning perspectives, are the specific expectations, knowledge, beliefs, attitudes or feelings that are used to interpret experiences (Cranton, 2006; Peters, 2014).
In the context of this study we were interested in a PSTs’ meaning schemes related to the function concept. Furthermore, given that they have previous knowledge of function, we are very specifically interested in provoking an elaboration or transformation of their existing meaning schemes. For example, a PST might elaborate on her meaning scheme for function by reconsidering her prior conception of function as a graph that passes the vertical line test and adopt a broader view of function that includes representations beyond merely graphs. Another might transform his incorrect understanding of function by rejecting his prior conception of a function being any equation and replacing it with a conception of a function being a mapping between two sets in which that mapping has specific properties.
Learning by changing meaning schemes (through elaboration or transformation) often begins with a stimulus, a disorienting dilemma, which requires students to question their current understandings that have been formed from experiences (Mezirow, 2009). This type of learning experience was of particular interest – both in our design of stimuli for it and the ways that meaning schemes are changed as a result. Given the evidence that PSTs often have a view of function that is limited to algebraic expressions and their associated graphs (e.g., Carlson 1998; Even, 1990) and that such understandings typically result in a vertical-line-test-related definition of function (e.g., Carlson, 1998), we aimed to design experiences that would problematize these understandings, thereby creating a stimulus for change.
One strategy that has been suggested for mitigating common misunderstandings related to function is the use of a function machine as a cognitive root. The idea of a cognitive root was introduced by Tall et al. (2000) as an “anchoring concept which the learner finds easy to comprehend, yet forms a basis on which a theory may be built” as he was developing a cognitive approach to calculus (p. 497). As an example of a cognitive root for function concepts, Tall et al. suggested the use of a function machine (sometimes referred to as a function box). The machine metaphor Tall and colleagues described is typically a “guess my rule” activity. In such activities, the inputs and associated outputs are provided, and students are challenged to determine what happened in the function machine (i.e., determine the function rule). While students are presented with a machine to embody the function concept, the rules used by the machine are algebraic in nature. In their studies using such machines proved quite promising, yet some students still struggled with connecting representations and determining what is and is not a function (McGowen, DeMarois, & Tall, 2000).
Given the promise of a machine metaphor as a cognitive root for function coupled with our desire to present a dilemma with which PSTs would need to grapple with given their current understandings, we set out to design a machine-based experience using representations that were unfamiliar for PSTs as a stimulus for examining their meaning schemes of function.
Applet Design
Unlike typical function machines (i.e., a number is put in, inside the machine the number is transformed by a function rule, and then a number is spit out), the applet we designed to trigger a disorienting dilemma in PSTs’ understanding of function contained no numerical or algebraic expressions. Instead it was built on the metaphor of a vending machine. Our intention was to avoid misconceptions that occur when students rely on an algebraic, and often procedural, view of functions (i.e., inconsistent use of the vertical line test).
Additionally, we hoped to emphasize the essential understandings identified by Cooney et al. (2010), in particular that functions apply to a wide range of situations and their domain and range do not have to be numbers. The Vending Machine applet (https://ggbm.at/rCtUxApF) is a GeoGebra file that contains five soda vending machines each with buttons for Red Cola, Diet Blue, Silver Mist, and Green Dew. The instructions direct users to explore the five machines and determine which are functions (Figure 1; McCulloch, Lee, & Hollebrands, 2015). When the user presses a button (input), one or more cans appear in the bottom of the machine (output). To remove the can(s) from the machine, the user clicks a reset button.
The functionality of each machine was designed to address misconceptions from the literature on distinguishing functions and nonfunctions. Machine A is the identity function; each button produces a can of the corresponding color. Machine B is the same as A, except when Silver Mist is selected, it produces two silver cans. This machine requires students to wrestle with the notion of what represents an element in the range. For Machine C, every button results in a single green can, the purpose of which is to present PSTs with a constant function to consider (i.e., the same number of cans of the same color for each button). For each button on Machine D, a single can is produced, but the color is different from the color of the button pressed. This machine was designed to problematize their occasional use of the term unique when thinking about outputs.
Finally, Machine E is similar to D, except the Silver Mist button randomly produces a can of a different color each time it is pressed. The purpose of Machine E is to provide a context in which testing the buttons on the machine once is not sufficient for determining whether or not the object is a function. The idea of testing the buttons more than once is foundational, and something that should be expected on all machines, as functions are predictable – meaning that if users know the function rule they can predict the output for any input.

Methods
The purpose of this study was to examine the effectiveness of the Vending Machine applet as a disorienting dilemma for PSTs as they consider the function concept. We specifically addressed the following research questions:
- In what ways does the vending machine applet provoke disorienting dilemmas related to PSTs understanding of function?
- In what ways do such dilemmas interact with the ways that PSTs make sense of functions and nonfunctions represented in the applet?
To address these questions, this study employed an embedded multiple case study intervention design (Yin, 2009). The cases were defined as individual students’ work on the various machines as a collective. We were interested in the different decisions students made as they engaged with the applet, paying particular attention to disorienting dilemmas that may have occurred for each case. Within the case, we aimed to understand any dilemmas that occurred and if and how they were resolved. Between the cases, we intended to illuminate the various ways in which the applet served to disrupt students’ current understandings to develop a more robust meaning of function.
Participants
The participants in this study were PSTs enrolled in a senior level content-focused methods course. Nine (referred to using pseudonyms) of the 10 PSTs enrolled in the course were present and completed the study activities. Of the nine, seven were undergraduate secondary mathematics education majors (three of the seven were also dual mathematics majors), and the other two were enrolled in the Master of Arts in Teaching (initial licensure) program. All nine were in their final semester of coursework prior to student teaching.
Data Collection
Data for this study included all student work related to the implementation of the Vending Machine task. We asked PSTs individually to write a definition of a function, including examples and nonexamples. Next, a whole class discussion was facilitated using their definitions through which the class agreed upon the following definition: “A function is a mathematical relationship such that each input has exactly one output.” Then, they were asked to engage in the Vending Machine applet task as a homework assignment. They were to explore the machines to determine which were functions and which were nonfunctions. Simultaneously, they completed a worksheet to provide written documentation of their thinking.
Following the task, PSTs completed a written reflection in which they were asked to revise the agreed-upon definition of function based on their experience with the task, to reflect on the different representations of functions presented in the task, and to discuss aspects of function highlighted by different machines with which they engaged (including possible uses with students).
The data sources for this implementation included written artifacts and video recorded screen captures. We collected students’ written initial definitions, their written responses to the Vending Machine task, and their written reflections (including revised definitions if they chose to do so). In addition, each PST captured a screencast of their work during their interaction with the Vending Machine applet as they followed a think-aloud protocol while working on the task. PSTs uploaded their screencasts to a shared, secure online folder and submitted their written work during the following class session.
Data Analysis
To begin our analysis process, all video recorded data were transcribed including both verbatim transcription of verbal utterances and descriptions of student actions within the applets. Transcriptions were organized in a table that noted machine, transcript of verbal utterances, and description of coordinated actions (see Figure 2). Next, we determined for each machine which students correctly identified it as a function or nonfunction.

Each machine in the applet was designed to provoke a disorienting dilemma related to the PSTs’ current understandings of function. Within the data related to each machine, we aimed to make sense of the PSTs changes in function conceptions (i.e., transformations of their meaning schemes). To identify possible disorienting dilemmas, we used a modified version of Powell, Francisco, and Maher’s (2003) method for video analysis. We viewed the screencasts to identify critical moments in which PSTs expressed surprise, confusion, or doubt about if a machine was a function or not.
For example, as one student engaged with Machine E, he stated, “Silver is blue, now silver is silver…this is really, really confusing. Silver is changing from blue, green, silver and red.” We identified this critical event as a disorienting dilemma, since the student expressed confusion about what was happening when he pressed the Silver Mist button. We then examined the transcript to make sense of the PSTs’ explanations and actions, how they resolved any dilemmas that occurred, and if they made any changes to their definition of function.
Next, we created a storyline for each PST, tying together the dilemmas they faced (if any), and the ways they reacted to them (both actions they took within the applet and what they said). Three cases, representative of the PST data as a whole, were chosen to help us understand how the applet did or did not create a stimulus for PSTs to examine (and possibly transform) their meaning schemes. We created detailed descriptions of the three selected cases in order to create a complete picture of how each PST engaged with the various machines and the conceptions of function they were drawing upon to make their decisions.
From the three cases, we completed a with-in case analysis by answering the research question for each case. Then, we completed a cross-case comparison (Merriam, 1998) to facilitate further clarification about which machines caused disorienting dilemmas and how the PSTs resolved the dilemmas they encountered.
Findings
Our findings are organized similar to our analysis process. We present findings regarding PSTs’ identification of function or nonfunction for each machine and whether or not a disorienting dilemma was experienced. Next, we present three cases, selected as representative of the various ways participants made sense of the disorienting dilemmas they encountered when interacting with the function machine applet.
Identification of Machines as Functions or Nonfunctions
Of the nine PSTs completing the function machine task, five of them provided correct responses for all five machines. The other four PSTs’ errors were related to Machine B and/or Machine E (see Table 1). Their interpretations and characterizations of the independent and dependent variables (referred to as “inputs” and “outputs” by most PSTs) were central to their determination of whether or not each machine was a function. All but three PSTs experienced a disorienting dilemma. Those who did not, also identified both Machine B and Machine E incorrectly.
Table 1
PSTs’ Worksheet Responses: Function or Nonfunction
| Machine | Jamie | Sam | Morgan | Kennedy | Blake | Quinn | Drew | River | Taylor |
| A | F | F | F | F | F | F | F | F | F |
| B | F | N | F | N | N | N | F | F | F |
| C | F | F | F | F | F | F | F | F | F |
| D | F | F | F | F | F | F | F | F | F |
| E | N | N | N | F | F | F | N | N | N |
| Experienced a disorienting dilemma | |||||||||
| – | Y | Y | Y | N | N | N | Y | Y | Y |
Examples of Vending Machine Dilemmas and Sense Making
Each of the three cases are presented here, providing insight into the ways the participants engaged with the vending machine applet, reacted to the disorienting dilemmas they faced, and as a result changed their meaning schemes for function (if they did). The first, the case of River, is representative of those PSTs who experienced dilemmas with each of the machines that were designed to provoke them. The second case, Jamie, is representative of those PSTs who experienced dilemmas with some, but not all, of the machines that were designed to provoke them. Finally, the case of Blake, is representative of a PST who did not experience any of the disorienting dilemmas for which we designed. For each of the cases a detailed description of the PST’s interaction with the applet is provided along with findings related to the PST’s sense making regarding the identification of individual machines as functions or nonfunctions.
River. River immediately began testing Machine A by pressing each button once, then pressing the reset button and repeating his button test. He tested each machine this way, by pressing every button, resetting the machine, and then pressing each button again. River then tested the input buttons in a different order to see if he got the same results.
River encountered his first dilemma when he presses the Silver Mist button on Machine B. He said, “Here we have something special, apparently. The colors remain the same, but here we have a little surprise. With Silver Mist we collect two items.” At this point he went back to press each button two more times, in different orders, to see which buttons were “fine” and which were “problematic.” He determined that only Silver Mist was problematic.
In trying to describe what he was seeing River stated, “It assigns to one input, two outputs.” As River continued to test the buttons he said, “We come to expect for every input, one drink. However, for the Silver Mist it is assigned two Silver Mists…and this kind of breaks the law of a function – every input should have one output, so this is not a function.” However, as soon as he stated that Machine B is not a function, he started to again consider the conditions under which it could be a function:
Except for one thing. If we assign for every input not only one object, but also a number…so one input would be one element and the output would be what we call a vector. So not only a color for the drink, but also a quantity. So, Red Cola would give (Red Cola, 1); and Diet Blue would give (Diet Blue, 1); Green Dew would give (Green Dew, 1); and Silver Mist would give (Silver Mist, 2)…and we could say that the quantity allowed are 1 or 2. So in that case, it would be a function. It would be a function that assigns to one element, a vector. [ARE YOU USING ELLIPSES (…) APA STYLE – THAT IS, TO INDICATE OMITTED SECTIONS OF QUOTATION?]
The two possibilities are expressed in his written response as well, as is shown in Figure 3.

On Machine C, like the previous machines, River pressed all four buttons before hitting the reset button. For this machine (the one in which all buttons return a single can of Green Mist) he appeared to get a can of Green Mist for the first button pressed and then nothing for each of the subsequent buttons. He said, “Red Cola returns a Green Mist; Diet Blue doesn’t give anything; Silver Mist doesn’t give anything; and Green Dew doesn’t give anything…apparently.” We interpreted his use of the word apparently at the end to indicate that he questioned this result.
Next, River pressed Red Cola again and got a Green Dew, then Diet Blue again. It appeared that there was no additional can (because its output was also a Green Dew). He stated, “For Diet Blue it appears that there is no image and this is problematic.” However, at this point he pressed the reset button and tried the Silver Mist button again and saw that it did indeed have an image, a Green Dew can. He pressed reset again, and then tried the Green Dew button once more. When he saw the output, we could hear excitement in his voice as he stated, “Green Dew also has an image, and it is Green Dew.” He then tested each button two more times. Eventually stating,
All of the inputs are thrown on one single output, Green Dew. So, it is a function! Although we might have the feeling that it was not at the beginning, but it is. Every input has exactly one output, and this output is a Green Dew.
The final dilemma that River faced was when he encountered Machine E. When testing the buttons on Machine E he went back to pressing all four soda buttons before pressing the reset button. In doing so, on the first try Red Cola produced Red Cola, Diet Blue produced Diet Blue, Silver Mist produced Green Dew and Green Dew looked like it did not produce anything. At this point he stated, “Here we have a problem. Green Dew doesn’t have anything.” However, as he was making that statement he pressed the reset button followed immediately by pressing the Green Dew button again and saw that this time it produced Green Dew. “Oh, this time it has something…how come?” He then began testing the buttons repeatedly and noticed that the Silver Mist button was not producing the same output each time,
It looks like it’s completely random. We have a problem…it’s not predictable what kind of image I’m supposed to have…so this is not a function…why isn’t it a function? Because a function is supposed to be an input set that we have, an output set. That’s something that we have too…and every input is supposed to have one image, or one output. But the way I throw each input on the output has to be unique. It’s not supposed to change all the time. I mean I have to be able to repeat… um…the assignment of each input to each output, it cannot change all the time…So machine E is not a function because we have no idea what to expect.
Similar to his interaction with Machines B and C, River began making sense of this dilemma by imagining situations in which the Machine would represent a function. When he could not, he worked to detail his reasoning for declaring it is not a function. All three machines that we designed to be dilemmas were indeed dilemmas for River, and they were in exactly the ways we anticipated. River worked through the dilemmas by not only testing the machines further, but also trying to make sense of what it would take for them to be functions rather than to just assume they were not.
Jamie. Jamie interacted Machine A by clicking each button once and without a problem identified it as a function. He continued to engage with Machine B in the same manner, however, after clicking the Silver Mist button and seeing two silver cans appearing, he said he thought it was a function but decided not to make a decision about Machine B yet. Instead, he continued on, trying each button on Machines C and D once. He declared C and D both functions without any hesitation. For Machine E, Jamie continued the same approach, but a green can appeared from the Silver Mist button and the Green Dew button. This result caused him to temporarily pause, but he ultimately identified Machine E as a function by comparing it to Machine C. Jamie explained,
[Machine E] seems like a function. It kind of works under the same principle as what C did, where everything in C gave a Green Dew. Even though that is not the case in Machine E, Red Cola and Diet Blue spit out two separate outputs but Silver Mist and Green Dew spit out the same. Just those two relate back to Machine C. So I would say it is a function.
Following this statement Jamie’s dilemma occurred: “This kind of makes me want to say that Machine B isn’t a function just because it seems like one of these wouldn’t be a function.”
Jamie decided to try each machine again, this time with a different approach. He clicked each button only once, but this time he did not click the reset in between each choice. As a result, this time when he tried Machine E’s Silver Mist button an additional can did not appear. He clicked reset and clicked the Silver Mist button five times in a row and realized that all four colored cans appeared.
I just went through and found that if I click Silver Mist I will get all of them. For Machine E if I click Silver Mist, I will get all the different cans. Which means Machine E isn’t representative of a function because, because I can get different outputs from Silver Mist. This would be an awful vending machine.
This dilemma of a button producing more than one color can caused Jamie to retry all the machines for a third time. This time he clicked each button on each machine several times to test for cans of different colors cans appearing from one button. When he tried Machine B’s Silver Mist he said, “Silver Mist only gives me two Silver Mists.” He declared that Machine B wa still a function and that all the machines are functions except Machine E. Then Jamie further clarified his understanding of Machine C:
Machine C is kind of like a – you can think of it as a y = 3. No matter what you put into it you are always going to get one thing out of it. And in Machine C’s case that is Green Dew.
For Jamie the appearance of different colored cans from the same button on Machine E was a disorienting dilemma. It caused him to not only stop and think about what was happening with that particular machine, but to also go back and retest the previous machines. Furthermore, this single dilemma not only allowed him to make sense of the machine on which it occurred, but also to make sense of Machine B – a Machine he had previously decided to reserve judgement on. Jamie’s experience with the Vending Machine applet provides an example of how a single disorienting dilemma can provoke reflection and ultimately a change in understanding.
Blake. Blake began with Machine A, pressing each button and resetting after each. She determined that Machine A is a function “because for every button you press, which is your input, you get an output, which is a soda.” Blake continued on to Machine B, proceeding the same way and paused after she pressed Silver Mist. “You get two. So when you put in an input, you get two outputs. In a function, you’re not supposed to get two outputs. So Machine B is not a function.” She was clearly focused on the number of outputs, regardless of what they were, to determine if the Machine is a function or not.
For Machine C, Blake maintained her pattern of pressing a soda color and resetting between each one. Again, her focus was on the number of cans. She stated,
Red, you still get one output. It doesn’t matter what it is, as long as you get one output. It could vary. A function could be sent to the same output, I believe? So, yes it’s a function. For every input, you get an output. Even though you get the same output, you still get an output, and an output means one and only one output that you’re getting.
Here, she appeared to be operating on the definition of function as “for every input you get an output.”
As Blake explored Machine D, she noted that you get one soda for each button (one Silver Mist, one Diet Blue, etc.). She again justified that this machine is a function, “because for every input you get an output.” Finally, she pressed each button for Machine E, noting that she got a Green Dew for both Silver Mist and Green Dew. She said,
I still think it’s a function. Um, it could be like absolute value or something to where like the Green Dew is a positive or something. I don’t know. But you can get two of the same inputs for a function. So, I mean, you can get two of the same outputs for any function. So I think it’s still a function because you’re still getting one output for each input.
In her written work, she emphasized the word “one” once she got to Machine D and E (Figure 4).

Blake never revisited a machine after exploring others, nor did she ever press a button more than two or three times. She determined if the machines were functions based solely on the number of outputs and not if the machines were predictable. This approach is algebraic in nature, and the way she engaged with the applet did not provoke a disorienting dilemma to change her understanding of function.
Discussion
With the crucial role function plays in high school and college mathematics, PSTs must have a deep understanding of functions themselves to be able to support the development of the function concept with their future students. To address this need, created and testd an applet based on a vending machine metaphor, designed to provoke disorienting dilemmas related to PSTs’ current understanding of function. The goal was to promote careful reflection and, ideally, an elaboration or transformation of meaning schemes related to the definition of function.
The vending machines were devised to address misconceptions from the literature on distinguishing between functions and nonfunctions (e.g., Breidenbach et al., 1992; Carlson, 1998, Even, 1990, 1993; Thompson, 1994b; Wilson, 1994). Specifically, our study investigated the ways in which this vending machine applet provoked disorienting dilemmas related to understanding of function and the ways in which such dilemmas interact with the ways that PSTs made sense of the functions and nonfunctions represented in the applet.
Based on our research questions and the evidence of the cases presented here, the applet clearly did not provoke disorienting dilemmas for all PSTs. Like the case of Blake, all of the PSTs who did not experience any dilemma (this includes Quinn and Drew) also did not identify Machines B and E as function or nonfunction correctly. However, all of the other PSTs experienced at least one disorienting dilemma as they engaged with the applet and, as a result, examined their meaning schemes related to the definition of function.
Furthermore, for most of these PSTs their meaning schemes related to the definition of function clearly deepened as a result. For example, elaborations or transformations in meaning schemes for function were evident in both River’s and Jamie’s engagement with the applet, as can be seen in their revisiting and sense making of Machine B after engaging with Machine E.
As this was our first use of the Vending Machine applet as a cognitive root for function with PSTs, affordances and limitations of its design highlighted in the results must be noted. While Machines B, C, and E were all designed with the intent of provoking disorienting dilemmas addressing common research based misconceptions, the results show that most PSTs in this study did not find Machine C to be problematic, but did recognize B and E as problematic. All of the PSTs either misidentified these machines or experienced dilemmas that resulted in examining their meaning schemes. (Note: Morgan identified Machine B as a nonfunction, but provided a sound explanation for this choice by explicitly identifying a restricted domain and range for which the statement would be true.)
The context of a vending machine appears to have been an affordance of the design of this applet. The use of nonalgebraic objects put the PSTs in an unfamiliar mathematical context, yet the fact that they could imagine using such machines appears to have been a powerful metaphor. For example, consider the ways that River and Jamie used the context in their meaning schemes in phrases like,
Machine C is kind of like a – you can think of it as a y = 3. No matter what you put into it, you are always going to get one thing out of it. And in Machine C’s case that is Green Dew.
The Vending Machine applet was designed to address misconceptions specifically identified in the literature related to Cooney et. al’s (2010) essential understandings of the function concept:
- Functions are single-valued mappings from one set – the domain of the function – to another-its range.
- Functions apply to a wide range of situations. They do not have to be described by any specific expression.
- The domain and range of functions do not have to be numbers. (p. 8)
As is evident by all three cases presented, PSTs were able to demonstrate an understanding of both 1b and 1c, in this case the nonalgebraic and nonnumeric context of vending machines. However, some PSTs, like Blake, were unable to demonstrate essential understanding 1a. These PSTs focused on the number of outputs did not experience a dilemma and continued to use procedural techniques to comprehend the set of input and output values.
PSTs who demonstrated the essential understanding 1a, like River and Jamie, used mapping thinking to consider the number of elements produced by an input and considered the predictability of the machines. These PSTs did not come to their conclusions about Machines B and E quickly or easily. Rather, the machines did appear to provide them with a disorienting dilemma that resulted in more careful consideration – and eventual revision of – their definition of function (e.g., “Oh! Oh, that’s weird!!!…Okay, okay, okay…” Jamie said as he pressed each button on Machine E and noticed that the Silver Mist can produced each kind of cola.). As such, this version of the Vending Machine applet used as a cognitive root for function shows promise as a way to move some PSTs from using procedural techniques to identify functions and nonfunctions that, in the past, have caused difficulties related to comprehending general mappings of input values to a set of output values (Carlson, 1998; Thompson, 1994a).
While using the vending machine context was clearly an affordance of the design of the applet, there were other aspects of the design that need further consideration. For example, the need to press the reset button to remove the output was first seen as a limitation, as PSTs often forgot to do so, ending up with multiple outputs showing at once or new outputs being hidden behind old outputs. The former possibly suggested incorrect output sets, and the later gave the impression that an input did not have an output. However, this feature was also an affordance in that it offered insight to PSTs’ thinking and often provoked a disorienting dilemma.
Another limitation that was highlighted in the case of Jamie was the number of machines that were included in the applet. Not enough examples of nonfunctions or functions with more than one element as an output were provided. In more than one instance PSTs got to the final machine and assumed it was a nonfunction simply because “at least one of these must be”. Furthermore, the limited number of machines might have contributed to the lack of a disorienting dilemma experience for Blake, Quinn, and Drew. Additional machines designed to provoke similar dilemmas in different ways might result in more PSTs experiencing them.
Finally, agreeing upon a class definition of function prior to engaging with the task might have changed the way that PSTs interacted with the applet. It was not clear if all PSTs were actually in agreement with the adopted definition. If one did not understand the agreed upon definition, it would be difficult to apply it to an analysis of the machines.
Given that we are suggesting a model that is slightly different from the traditional machine model as a cognitive root for function, it is worthwhile to consider the affordances and drawbacks of not only the design, but the use of a vending machine as a model for the function concept. The Vending Machine applet was designed utilizing no numeric or algebraic representations and in such a way that it aligned with the understandings of function, Cooney et al. (2010) defined as “essential understandings” of the function concept. In doing so, the applet can be used to develop conceptual understandings of what it means to be a function prior to focusing on procedures typically associated with algebraic representations, like the vertical line test.
The findings in this study support the notion that these are affordances of this model for function. On the other hand, this model has limitations. While it provides a strong foundation for function, it is not a model upon which we can continue to build an understanding of different characteristics of function, function families, and other related concepts. At some point the model falls apart and standard representations are necessary.
Revision to the Applet
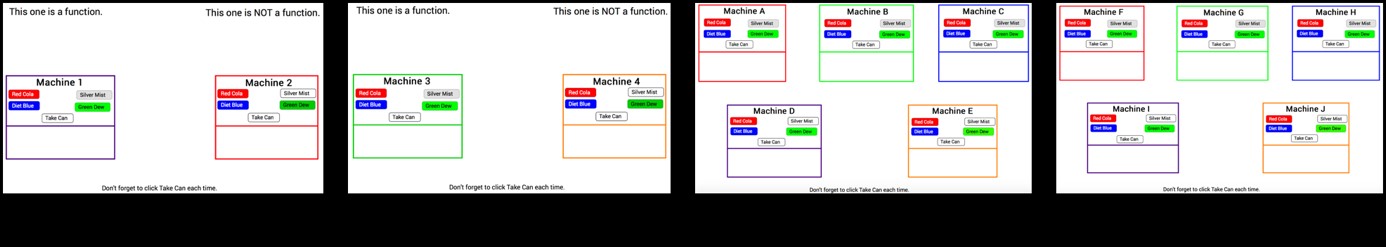
From this initial research on the PSTs’ engagement with the applet and the affordances and limitations identified, the applet has been revised. The new version (2.0) addresses PSTs’ interpretations of Machine B and Machine E and the limitations noted. It consists of four pages (Figure 5). The first two pages contain two vending machines. On each page one machine is labeled as a function and the other is labeled as not a function. The two nonfunction machines each have at least one button that produces a random can when pressed.
The new applet also provides the opportunity for PSTs to make a conjecture, after page 2, on why Machines 1 and 3 are functions and Machines 2 and 4 are nonfunctions. They then test their conjecture on the pages 3 and 4, that each contain five machines. The five original machines are included, along with five new machines. These new machines provide additional opportunities to examine machines that are not one-to-one, produce random pairs of cans as an output, and have random outputs for all four inputs.
Conclusion
From a broad perspective, the results of this study show the promise of framing PSTs’ learning using transformation theory. If goals within our mathematics education courses include PSTs thinking deeply about student thinking, their own mathematical thinking also needs to be pushed. However, given the extensive experiences they have had with high school mathematics, tasks within methods and content courses for PSTs need to be designed that take their existing knowledge into consideration and provide mechanisms for reflecting on and deepening that knowledge. The PSTs’ interactions with the Vending Machine applet suggests that providing tasks that promote disorienting dilemmas is a powerful way to promote changes in meaning schemes. This design is useful for framing PSTs’ mathematical learning environments.
Furthermore, focusing specifically on PSTs’ understanding of function, the results of this study suggest that the Vending Machine applet might be a powerful tool (cognitive root) for disrupting PSTs’ algebraic view of function and, thus, resulting in an elaboration or transformation of meaning schemes related to the concept of function. However, to determine whether or not these findings are generalizable, the implementation of the applet needs to be studied on a larger scale and in a variety of instructional contexts. For example, in this study the PSTs worked with the applet independently. Working with a peer may prompt dilemmas differently. Working with a peer may prompt different or deeper sense making as a result of those dilemmas.
Attending to different instructional contexts will help us maximize the benefits of the tool. In addition, to better understand how PSTs’ understanding of function changes as a result of experiences with the applet, rather than agreeing upon a shared definition of function prior to using the applet as was done in this study, a pretask measure of PSTs’ individual understanding of function may be included, so that changes can be identified and mapped to particular experiences with the applet.
Through further study and revision, this work may result in a solid cognitive root that disorients PSTs’ algebraic view of function, remedies existing misconceptions, and provide a foundation on which robust conceptual understanding of function can be built.
Acknowledgement
The work in this project was partially supported by the National Science Foundation Preparing to Teach Mathematics with Technology project, funded by the National Science Foundation with grants to NC State (DUE 1123001). The opinions, findings, and conclusions or recommendations in this paper are our own, and do not necessarily reflect the views of the National Science Foundation.
References
Bakar, M., & Tall, D. (1991). Students’ mental prototypes for functions and graphs. In F. Furinghetti (Ed.), Proceedings of the 15th Conference of the International Group for the Psychology of Mathematics Education, Vol. 1 (pp. 104–111). Retrieved from https://files.eric.ed.gov/fulltext/ED413162.pdf
Bannister, V. R. P. (2014). Flexible conceptions of perspectives and representations: An examination of pre-service mathematics teachers’ knowledge. International Journal of Education in Mathematics, Science and Technology, 2(3), 223-233.
Breidenbach, D., Dubinsky, E., Hawks, J., & Nichols, D. (1992). Development of the process conception of function. Educational Studies in Mathematics, 23, 247–285.
Brenner, M. E. (1998). Meaning and money. Educational Studies in Mathematics, 36, 123-155.
Carlson, M. P. (1998). A cross-sectional investigation of the development of the function concept. Research in Collegiate Mathematics Education, III, Issues in Mathematics Education, 7(1), 115-162.
Chesler, J. (2012). Pre-service secondary mathematics teachers making sense of definitions of functions. Mathematics Teacher Education and Development, 14(1), 27-40.
Clement, L. (2001). What do students really know about functions? Mathematics Teacher, 94(9), 745-748.
Confrey, J. (1990). What constructivism implies for teaching. In R. B. Davis, C. A. Maher, & N. Noddings (Eds.), Journal for Research in Mathematics Education Monograph No. 4: Constructivist views on the teaching and learning of mathematics (pp. 107-122). Reston, VA: National Council of Teachers of Mathematics.
Cooney, T. J., Beckman, S., & Lloyd, G. M. (2010). Developing essential understanding of functions for teaching mathematics in grades 9-12. Reston, VA: National Council of Teachers of Mathematics.
Cranton, P. (2006). Understanding and promoting transformative learning: A guide for educators of adults. San Francisco, CA: Jossey-Bass.
Dubinsky, E., & Harel, G. (1992). The nature of the process conception of function. In E. Dubinsky & G. Harel (Eds.), The concept of function: aspects of epistemology and pedagogy (pp. 85–106). Washington, DC: Mathematical Association of America.
Dreher, A., & Kuntze, S. (2015). Teachers’ professional knowledge and noticing: The case of multiple representations in the mathematics classroom. Educational Studies in Mathematics, 88(1), 89-114.
Even, R. (1990). Subject matter knowledge for teaching and the case of functions. Educational Studies in Mathematics, 21(6), 521-544.
Even, R. (1993). Subject-matter knowledge and pedagogical content knowledge: Prospective secondary teachers and the function concept. Journal for Research in Mathematics Education, 24(2), 94-116.
Fernandez, E. (2005). Understanding functions without using the vertical line test. Mathematics Teacher, 99(2), 96-100.
Kabael, T. U. (2011). Generalizing single variable functions to two-variable functions, function machine and APOS. Educational Sciences: Theory and Practice, 11(1), 484-499.
Leinhardt, G., Zaslavsky, O., & Stein, M. K. (1990). Functions, graphs and graphing: Tasks, learning and teaching. Review of Educational Research, 60(1), 11-64.
McCulloch, A.W., Lee, H.S., & Hollebrands, K. (2015). Preparing to teach mathematics with technology: An integrated approach to algebra (1st ed.). Retrieved from http://ptmt.fi.ncsu.edu
McGowen, M., DeMarois, P., & Tall, D. (2000). Using the function machine as a cognitive root. In M. L. Fernandez (Ed.), Proceedings of the 22nd annual meeting of the North American Chapter of the International Group for the Psychology of Mathematics Education (pp. 247-254). Indianapolis, IN: Hoosier Association of Mathematics Teacher Educators
Mezirow, J. (2009). Transformative learning theory. In J. Merizow & E. W. Taylor (Eds.), Transformative learning in practice: Insights from community, workplace, and higher education (pp. 18-31). San Francisco, CA: Jossey-Bass.
Merriam, S. B. (1998). Qualitative research and case study applications in education. San Francisco, CA: Jossey-Bass Publishers.
National Governors Association Center for Best Practice & Council of Chief State School Officers. (2010). Common core state standards for mathematics. Washington DC: Author.
Oehrtman, M. C., Carlson, M. P., & Thompson, P. W. (2008). Foundational reasoning abilities that promote coherence in students’ understandings of function. In M. P. Carlson & C. Rasmussen (Eds.), Making the connection: Research and practice in undergraduate mathematics (pp. 27-42). Washington, DC: Mathematical Association of America.
Peters, S. A. (2014). Developing understanding of statistical variation: Secondary statistics teachers’ perceptions and recollections of learning factors. Journal of Mathematics Teacher Education, 17(6), 539-582.
Powell, A. B., Francisco, J. M., & Maher, C. A. (2003). An analytical model for studying the development of learners’ mathematical ideas and reasoning using videotape data. Journal of Mathematical Behavior, 22, 405-435.
Rasmussen, C. L. (2000). New directions in differential equations: A framework for interpreting students’ understandings and difficulties. Journal of Mathematical Behavior, 20, 55-87.
Sierpinska, A. (1992). On the understanding the notion of function, In G. Harel & E. Dubinsky (Eds.), The concept of function: Aspects of epistemology and pedagogy (MAA Notes, 25). Washington, DC: Mathematical Association of America.
Stylianou, D. A. (2011). An examination of middle school students’ representation practices in mathematical problem solving through the lens of expert work: Towards an organizing scheme. Educational Studies in Mathematics, 76(3), 265-280.
Tall, D., McGowen, M., & DeMarois, P. (2000). The function machine as a cognitive root for the function concept. In M. L. Fernandez (Ed.), Proceedings of the 22nd annual meeting of the North American chapter of the International Group for the Psychology of Mathematics Education, (Vol. 1, pp. 255-261), Tucson, AZ.
Thompson, P. W. (1994a). Images of rate and operational understanding of the fundamental theorem of calculus. Educational Studies in Mathematics, 26, 229– 274.
Thompson, P. W. (1994b). Students, functions, and the undergraduate curriculum. In A. H. Schoenfeld & J. J. Kaput (Eds.), CBMS Issues in Mathematics Education: Research in Collegiate Mathematics Education, 4, 21–44.
Vinner, S., & Dreyfus, T. (1989). Images and definitions for the concept of function. Journal for Research in Mathematics Education, 20(4), 356-366.
Wilson, M. R. (1994). One preservice secondary teachers’ understanding of function: The impact of course integrating mathematical content and pedagogy. Journal for Research in Mathematics Education, 25(4), 346-370.
Yin, R. K. (2009). Case study research: Design and methods (4th ed.). Thousand Oaks, CA: Sage Publications, Inc.
![]()