How can mathematics teacher educators engage preservice teachers (PSTs) in the work of teaching before they are ready to practice in real classrooms? This question has been foundational in our design of LessonSketch (www.lessonsketch.org), a Web-based research and development platform for the study of teaching. LessonSketch and, in particular, its storyboarding tool Depict, show a way in which online learning can be a part of practice-based teacher development.
The Internet, particularly Web 2.0 with its capacity to enable users to create rich media files, has brought an important infrastructure to the work of professional education. The Internet not only enables the creation of communities whose members might be geographically displaced but also enables their communication to happen through much more than written text, including user-created video and computer graphics.
Although much current online education still relies on the transaction of written texts and the mere consumption of video by learners (Lonn & Teasley, 2009; Zhang, Zhou, Briggs, & Nunamaker, 2006), we see a not-too-distant future when professional educators and trainers will take better advantage of this technological infrastructure to support professional training focused on the study of professional practice—specifically, by creating online learning experiences where trainees create and transact professional artifacts.
In this paper, we demonstrate what this future can look like in two ways: By describing the features of LessonSketch that capitalize on the technological infrastructure to create resources for professional educators to work with their clients and by describing the actual work we have done with those resources in the professional preparation of PSTs in the context of a secondary mathematics methods class.
A View on Learning Applied to the Learning of Teaching
An important foundation of the technology we have designed comes from what Papert and Harel (1991) called the “constructionist” theory of learning, whereby learners learn by engaging in the construction of public artifacts. Constructionism is one of many approaches to active learning, but it distinguishes itself in that it puts a premium on students’ activity as producing material goods that others can interact with: Those artifacts can be artwork, physical models, poems, software, or videos—a wide variety of artifacts that have the common characteristic that they persist as public assets even after the experience of making them has ended.
Brousseau’s (1997) notion of the milieu—the counterpart to the learner in a learning situation—helps explain the role that artifact construction can play in learning. In Brousseau’s theory, the milieu is that part of the environment that receives the actions of the learner and that, by reacting to those actions (resisting or triggering consequences), informs the learner’s actions, or differently said, it provides the learner with feedback. We use feedback in its original sense of a reaction that informs an action. We do not usually include the summative evaluations that instructors may provide (e.g., “good work”) for the transactional value of an artifact created. We would include the more formative responses that either peers or instructors provide when they enter into the world of the artifact (e.g., “You have only five students in your class?”).
For Brousseau (1997) a milieu could be material, social, or technological, but the notion of constructionism points, in particular, to material and technological milieus that the action of the learner can alter significantly. In this kind of milieu the constructive actions of the learner might summon laws of nature that may have been muted before, and they may, in turn, become sources of feedback for the learner. Consider as an example the case of wood blocks. If a child merely uses them to cover the floor, he or she may not need to contend with gravity and, thus, will not have to cope with the consequences of misjudging a block’s center of mass. As soon as the child erects vertical structures where some blocks are partially supported, gravity becomes an important phenomenon to contend with, and some understanding of center of mass becomes a way of coping with the consequences of gravity. In other words, the actions of the learner choosing to build a vertical structure summon the laws of gravity, which were muted in a flat structure, and the laws of gravity now participate in providing feedback to the child as to how to build vertically with the wooden blocks.
Before arguing the importance of this constructionist perspective for teacher education, a consideration of the role of practice in learning to teach is in order. Teaching is a practice in addition to a realm of knowledge. For the prospective professional, the learning of teaching requires more than learning academic knowledge about teaching; it requires learning to do the work of teaching. Appropriately, Lampert (2010) advocated for the learning of teaching in, from, and for practice, meaning that practice provides the context and means for learning, in addition to providing the subject matter to be studied and the goals to be met. Engagement in the work of teaching allows such learning in practice. Thus, the learning of teaching in practice seems to call for active pedagogies of practice, for example, the identification of high-leverage practices (Ball, Sleep, Boerst, & Bass, 2009) or the rehearsal of particular routines and strategies (Lampert et al., 2013).
The verb practice names a particular way of understanding. Teacher educators may be familiar with Ryle’s (1945) distinction between knowing that and knowing how, whereby regarding a practice, Ryle argued that knowing how is a concept “logically prior to the concept of knowledge-that” (p. 4, 5).
In his elaborations on Heidegger’s Being and Time, Dreyfus (1980) posed a slightly different distinction between the knowledge of the practitioner and that of the observer of a practice. Dreyfus (1980) spoke of “practical holism” and set it in contrast with the “theoretical holism” (more common among academics) in a particularly important aspect: While theoretical holism permits its practitioner to voluntarily confront, accept, and bring into consideration observations about the world and organize them in ways that satisfy the intellect, practical holism requires the practitioner to cope with demands that quite often impose and call attention to themselves regardless of how willingly or skillfully the practitioner can take care of them.
That distinction applies no less to teaching (where unlike theorists, practitioners cannot really choose what they have to deal with) as it applies to the learning of yoga poses (distinguishing the theoretical knowledge of the geometry of the poses from the practical knowledge involved in the reproduction of the poses using one’s own body) or the learning of a musical instrument (distinguishing the theoretical knowledge of where to position the left hand on the board of a string instrument from the practical knowledge of how to achieve those positions with one’s own arm, hand, and fingers).
In actual practicing, one comes to grips with demands that might had been unknown to be part of the work (e.g., the existence and compulsion of particular muscles) but that have a practical bearing on the feedback being sought (e.g., the quality of the sound produced or the image of the pose achieved). Practice thus brings up things to cope with (e.g., muscle pain). By coping with those demands, one will not necessarily advance a lot in understanding what those demands really are, but one might learn to manage them so as to produce practical outcomes that are pleasing or effective. Like yoga or music, the learning of teaching in practice is useful insofar as it creates opportunities to cope with those demands.
In this paper we argue that constructionism has a role in such learning in practice: Engagement in the construction of artifacts can support prospective practitioners’ learning of the work of teaching. It can do so, in particular, by engaging them in interactions with a milieu in which they also get to cope with the demands of practice. We elaborate this point by describing specifically the artifacts that we have had prospective teachers create in the LessonSketch platform, the kind of feedback that they can expect on their actions, and how it can assist their learning to teach.
Pedagogies of Practice: The Role of Approximations
One way in which professional education (including teacher preparation) has been able to focus on learning practice has been by implementing what Grossman et al. (2009) called “pedagogies of practice,” which include what they call “representations,” “decompositions,” and “approximations” of practice. Approximations of practice are “opportunities to engage in practices that are more or less proximal to the practices of a profession” (p. 2056), which “may fall along a continuum, from less complete and authentic to more complete and authentic” (Grossman et al., 2009, p. 2078).
Although student teaching is a canonical example of an approximation of practice in teacher preparation, it is not the only one. Unit and lesson planning, rehearsals, role plays, and simulations are also considered approximations of practice. They can vary in their authenticity but still be considered approximations of practice inasmuch as they include particular aspects of practice that the professional candidate is expected to do (rather than to see or read about). As Grossman et al. (2009) noted, “By definition, approximations of practice are not the real thing. They differ with regard to the level of completeness and congruence with which they approximate practice” (p. 2078).
In the case of early professional preparation, be that with prospective teachers or prospective physicians, practice with actual clients may arguably be too risky, but engaging in other approximations of practice may give the opportunity to learn in practice. We are interested here in the role that technological mediation and, particularly, online interactions can play in creating such approximations.
In regard to how they provide feedback, two ideal types of technologically mediated approximations of practice for learning teaching can be distinguished. The first of them is inspired in computer games where the user plays a well-defined role, aims for specified goals, and receives specific feedback from the system. A low-tech version of such approximation is the simulated patient used in the professional preparation of physicians (Lane, Slavin, & Ziv, 2001). The second is inspired in authoring applications, where users pursue their own goals by employing their choice of tools from many available and where the traces of their activity are the sources of feedback. A low-tech version of such approximation is the authoring of lesson plays, explained by Zazkis, Sinclair, and Liljedahl (2013).
The two types are mostly distinguished here by the specificity with which the milieu in the approximation responds to the moves by the learner. In the first case the feedback is external to the learner, to some extent preprogrammed, and executed through a mechanism that the learner does not really see. In the second case, the feedback can come from the learners themselves when they switch from a constructing mode to a reading and interpreting mode. For simplicity we will call the former simulators and the latter microworlds. Each of them has its own advantages, and our distinction is meant only to highlight their respective advantages rather than to discount any of them.
At the one extreme, simulators provide controlled conditions of a certain domain that can help the apprentice learn to solve a limited set of problems in that domain by adjusting input variables of a simulator and observing and examining output variables of the same simulator (Ören, 2009). A simulated student that embodies a particular personality can involve the PST in specific practical work and knowledge use needed for handling the particular needs and goals of some kinds of students. Some online teacher education applications are like this, particularly SimSchool (Gibson, 2007; Zibit & Gibson, 2005): Users learn to associate teaching moves to particular students by adapting to the reactions of students to earlier moves, while these reactions might result from theories and models inscribed in the software by the designer. In SimSchool’s case, one of these is a theory of personality (McCrae & Costa, 1996) and another one is an interpersonal theory (Kiesler, 1983).
Christensen, Knezek, Tyler-Wood, and Gibson (2011) have indicated that SimSchool has been useful in helping PSTs improve their instructional self-efficacy (e.g., confidence in their competence to make positive learning happen, even in adverse learning conditions) and, in some circumstances, improve their basic teaching skills (e.g., stating learning objectives clearly or selecting learning objectives that are aligned with students’ needs). The TeachLive program (Dieker, Rodríguez, Lignugaris, Hynes, & Hughes, 2013; Hayes, Straub, Dieker, Hughes, & Hynes, 2014) is another such example, similar to SimSchool in professional learning objectives, though different in its computing and graphics realization.
At the other extreme, authoring environments, microworlds, and virtual worlds such as LOGO (Papert, 1980, 1993), Minecraft (Short, 2012), or Second Life (Boulos, Hetherington, & Wheeler, 2007) provide the learner with self-regulated learning opportunities, as Rieber (1996, p. 47) suggested that “learners are expected to self regulate their own learning in a microworld. Self-regulated learning is when a person takes responsibility for his or her learning and, as a result, takes appropriate action to ensure that learning takes place.”
According to Rieber (1996), two major characteristics that make microworlds distinct from simulations are (a) the learner is presented with a simple case of a domain at first and then has the chance to explore increasingly complex ideas or models over time, and (b) the environment must match the cognitive and affective state of the learners so that they know what to do immediately within the environment, with little or no technical support.
While simulations can be useful in mathematics teacher education (Chieu & Herbst, 2011), microworlds where PSTs create artifacts (what Grossman et al., 2009, called representations of teaching) can also be useful tools to approximate teaching. Related to constructionism, these environments can help PSTs learn practice by virtually playing or living in a microworld (Rieber, 1996). In particular, a microworld that enables PSTs to create representations of teaching can allow them to prototype and visualize lessons, making concrete what they might envision doing in a lesson. They can also possibly critique it by comparing it to images they might hold of realistic or successful lessons. The LessonSketch platform prototypes what such a microworld could look like.
LessonSketch and Its Tools for Approximating Practice
The LessonSketch platform (see Herbst, Aaron, & Chieu, 2013) was designed to support the use of rich media in the study of teaching by researchers and practitioners. It contains media resources and authoring and communication tools that teacher educators and preservice teachers can use to construct, visualize, annotate, share, and discuss representations of teaching.
In particular, the Depict tool (Herbst & Chieu, 2011) allows users to create classroom scenarios in the form of storyboards by dragging and dropping and manipulating classroom graphics (such as teacher, students, and furniture) to create sequences of still frames, to which they can add speech bubbles. Users can represent a lesson using such a storyboard; they can then do many things with it, including viewing it or annotating it and sharing it with others.
In designing Depict, Herbst and Chieu (2011) strove to combine, metaphorically speaking, affordances of two types of authoring environments. On the one hand is the authoring environment of word processing software, which can be used flexibly to create lesson plans and the lesson plays used by Zazkis et al. (2013) and Crespo, Oslund, and Parks (2011), perhaps in connection with special forms or tables.
The word processing software enables a transparent representation process (the user does not need to spend a lot of time or effort learning a special language to manage the authoring tool) at the expense of relatively low face validity of the representation created (the scenarios produced do not really look like teaching—they look like words on a page). Still, this authoring environment can be very useful. In his role as teacher educator, the first author has been involving PSTs in the creation of classroom dialogs since 2004, using for that purpose word processing software (Ghousseini, 2008; Ghousseini & Herbst, 2014).
On the other hand are programming environments like LOGO (Papert, 1980), Scratch (Maloney, Resnick, Rusk, Silverman, & Eastmond, 2010) or Adobe Flash, where there are distinct mechanisms for creation and feedback (in LOGO this is apparent in the two panels, one panel where the learner programs in code to construct the artifact and the other panel where the learner observes the consequent behavior of the turtle, which provides feedback on the construction code; see Olive, 1991; Papert, 1980, 1993). We were cognizant of the usefulness of Adobe Flash as an authoring environment to create classroom scenarios, since we had been using it along with dedicated graphics to produce animations that we would later utilize as prompts in conversations among experienced teachers about practice (Herbst, Nachlieli, & Chazan, 2011). We had been employing and directing graphic artists to use the Adobe Flash environment; we realized that we could not expect PSTs to use that authoring environment in the same way that a graphic artist would.
Yet, considering the extent to which we knew practitioners could engross themselves with such scenarios, we wondered whether an authoring environment could be created for teachers to produce their own scenarios, requiring a minimum of specialized knowledge of the software but enabling them to produce artifacts with good face validity. In particular, we were interested in an authoring environment that would enable users to better represent the nonverbal aspects of classroom interaction, including its multivocality (many possible interacting people) and multimodality (many modalities of communication, including facial expression, gesture, inscriptions, and so on; see Herbst, Chazan, Chen, Chieu, & Weiss, 2011). The drag-and-drop and layering schemes that have become commonplace in the user interface of presentation software (e.g., Microsoft’s Powerpoint) were useful starters for such design.
Herbst and Chieu (2011) created Depict as a step toward such an authoring environment that would allow visual, collaborative, and relatively fast authoring of representations of teaching by teachers—lessons that could be recorded, communicated, annotated, discussed, and improved. For PSTs the creation of such representations could be a way of approximating practice, while for in-service practitioners it could be a way of creating the kind of shared artifacts that constitute a basis of professional knowledge (Morris & Hiebert, 2011).
A question we contended with in that design work was how to think about the graphics that the software would manage in order to enable the representation of classroom scenarios. We took the perspective that the graphics were a semiotic system, the basis of a language of representation. In such consideration it was useful to think of the contrast between the semiotic systems managed in writing and in videorecording.
In written cases, lesson scripts, or lesson plays, written language turns all classroom events into abstract symbols (written words and sentences). Some of that language use resembles closely the events represented. Lines of the script may transcribe much of what transpires in spoken text (e.g., much of the ideational meanings transacted), but written language is limited in its capacity to represent other aspects of lessons—the unspoken, in particular. So many classroom events are nonverbal and even occur simultaneously (Doyle, 1986) that a text describing them is not likely to represent them in a way that engrosses the reader.
At the opposite extreme are videotaped enactments. These videos can capture much of the nonverbal complexity of classroom events (but see Hall, 2000) but probably too much. Videotaped enactments can be shared online and commented on. Their improvement, however—for example replacing segments from an enactment with new footage—could easily create semiotic discontinuities in the way the video represents the individuality of events, participants, and settings. Say, a PST wanted to represent a classroom scenario by videotaping a skit enacted with the collaboration of some of his friends and, after recording it, realized that one brief segment had not recorded well. The addition of new footage to supply for that lack would run into difficulties that might be beside the point of the representation (e.g., the need to find exactly the same crew and setting for an enactment at a different time vs. the need to splice video clips that use different actors to represent something that putatively was a single event). The visual language of day-to-day human interaction seems to include too many semiotic variables that the PST would need to manage in order to use video enactments for the kind of approximation of practice we wanted to enable.
The use of a symbol system whose visual component consisted of a nondescript cast of cartoon characters provided a way out of the problem of what symbol system to use to represent practice. Depict relies on the dragging and dropping of graphics that add a visual component to the language component. Depict calls for users’ knowledge of simple graphics management (as called for by presentation software packages), such as dragging, layering, and zooming as part of its user interface language. Depict also manipulates a dedicated set of classroom graphic assets that we describe as a cast of characters (see two examples in Figures 1a and 1b).

 Figure 1a. ThExpians B Figure 1b. ThExpians C
Figure 1a. ThExpians B Figure 1b. ThExpians C
It matters less what those characters look like than the fact that they are designed to enable some individual differences but not others. The current version of Depict manipulates a cast of characters called ThExpians B (see Figure 1a) where most physical individual differences among people are muted (characters have the same eyes, head, height, skin color, and weight but can be distinguished by their shirt color, physical location, physical orientation, and facial expression). Other character sets could be used with Depict to phase in other sources of individual differences (see Figure 1b for a character set that phases in semiotic resources to represent skin color, age, and occupation).
Our decision to use simple cartoon characters as a graphic language for classroom interaction aimed at enabling rapid prototyping of scenarios that could be easily revised. The bulk of the work of the user is not in designing who the individuals are but in designing what individuals do. Users can, therefore, not only script what classroom participants would say but also represent what they would do, including what they would write on the board, the facial expressions they might make, and so forth.
The low demands of graphical representation not only make the users’ work simpler but it also make it easier for users to engage in a make-believe game, whereby the scenario they author can represent what they and their students would do. We have observed teachers’ capacity to project their own settings onto these kind of nondescript graphics (Chazan & Herbst, 2012) and are also aware that more detailed graphic realizations can run into response problems (e.g., the uncanny valley; see Mori, 1970). Further, since users can not only create but they can also share, annotate, discuss, and edit those representations, the combination of depiction and visualization creates a feedback loop that can support learning in similar ways as the turtle graphics provide feedback to the programming in the LOGO microworld.
Microworlds such as LOGO are an apt metaphor to conceptualize what Depict provides with its creation component and its view component. The creation component consists of tabs, graphics, and commands that PSTs can use to create representations of classroom scenarios. The view component is a simple storyboard player used to display what a scenario looks like through navigation components (thumbnail images, arrows) and a canvas. In a typical procedure of creating a classroom scenario, PSTs drag and drop a classroom background that contains a teacher desk, a whiteboard, posters, windows, and so on. Then, they drag and drop furniture (e.g., student desks), supplies on the desks or floor (e.g., pencils, books, and computers), a teacher character, and a number of student characters.
For each character, they can manipulate its position on the canvas, its view (front, back, side), and its shirt color and add facial expressions (e.g., frustrated, happy, smiling, talking) and hands. They can also insert dialogs in the form of speech bubbles for the teacher and the students (with different shapes to indicate some prosodic differences) and props such as books, paper, pencil, manipulatives, calculators, and so forth. To add content to the white board, PSTs can use a drawing tool (Inscribe, also available in LessonSketch), upload image files from their own computers, or use web links on the Internet.
To facilitate the PSTs’ work, Depict provides a number of programmed subprocedures or templates that allow them to create instances of classroom settings quickly (e.g., a front, back, or side view of a classroom with a teacher character and a number of student desks and characters already placed in standard locations). PSTs can also use the copy, cut, paste, duplicate, and delete functions to repeat or remove some subprocedures they used before (e.g., duplicate a character or a slide they created earlier). Other useful functions include zooming in and out, layering the graphics, changing the order of frames, saving the depiction file to their personal resources, and rendering the depiction in a storyboard format that can be shared with and viewed by other users easily.
Most of the creation commands are in the drag-and-drop form within a graphical user interface, thus enhancing the WYSIWYG (what you see is what you get) character of the software. Like in the LOGO environment, where the user can look at the graphical representation to get feedback on their production, the PST can look at the Depict canvas to get immediate feedback on events of the scenario being created.
For example, a frame in which too few students were visible could tell PSTs that they might need to add more students if they want the scenario to look like one that happened in a real classroom. Also, a frame in which an eager expression is added to a student character might suggest the need to include a speech bubble for the student to say something to explain him or herself. A frame in which a task requires work in groups but where the class is seen sitting in rows could hint to the user the need to reorganize student desks and characters.
PSTs can also receive feedback on their depictions from peers and from the teacher educator. Advanced users of LessonSketch (such as teacher educators) may create shared folders and invite their PSTs to put their depictions still being created in that folder so they can be created in collaboration with other users. When they have a scenario to share more widely, they can render the depiction and add it to the collection of “My Stories” where they define who can access those files. Those individuals are then able to use Annotate, a media annotation tool also available on LessonSketch, to comment on the depictions they have access to, frame by frame.
PSTs can also discuss those depictions with each other or with the teacher educator in a forum using Discuss, an advanced communication tool in LessonSketch that allows users to create a media-based forum. During the discussion, they can view existing depictions and create alternative versions of those existing depictions that can then be attached back to the discussion forum. This process can continue on and result in an agreed-upon classroom scenario and even in a set of related but different classroom scenarios. After that, they can publish their work to the collection of “Contributed Stories,” where all LessonSketch users could see a depiction, could annotate it individually or in small groups, or discuss it collectively in a story-based Forum.
The feedback by peers, the teacher educator, and other LessonSketch users is not immediate, like the feedback users get when they view their own depictions. Instead, it complements that one by bringing other people’s perspectives, and it is useful because it can help PSTs see what they might not have seen when they looked at the graphical representation by themselves. In summary, there is reason to believe this form of creation, evaluation, and collaboration around the approximation of practice could help PSTs learn about different aspects of professional practice, which is illustrated in the following section.
A Practice-Based Secondary Mathematics Methods Course
Web-based software like Depict can play a role in practice-based teacher education. Teacher education is now in a state of transition, shifting from a focus on theory and reflection toward a practice-based approach, which focuses on learning to do the work of teaching (Ball & Forzani, 2009; Grossman, Hammerness, & McDonald, 2009). In practice-based teacher education, PSTs learn a core set of teaching practices extracted from the teaching profession; to facilitate their learning of those practices, the practices are decomposed into constituent elements (Grossman et al., 2009). Preservice teachers study those practices through inspecting representations of instruction, such as records of students’ work, teachers’ lesson plans, or video records of classroom instruction.
With the assistance of teacher educators, PSTs decompose those practices into constituent elements. They may draw on rubrics or grading tools that account for the strategies, routines, and techniques involved in a practice (Boerst, Sleep, Ball, & Bass, 2011; Ghousseini, 2008; Herbst, 2011a, 2011b, 2011c, 2014). More importantly, though, in practice-based teacher education PSTs study those practices through varied and multiple opportunities to approximate teaching. In other words, a “practice-focused curriculum” includes not only a focus on knowledge and “the actual tasks and activities involved in the work” of teaching but “emphasize[s] repeated opportunities for novices to practice carrying out the interactive work of teaching and not just to talk about that work” (Ball & Forzani, 2009, p. 503). Enactment through rehearsal is one way in which PSTs get involved in approximating practice (Lampert et al., 2013).
Starting in the fall 2004, the first author started changing his secondary mathematics teaching methods course into a practice-based approach. At the time the change was predicated on the need to increase the value that PSTs perceived in a secondary methods class that appeared to students as more centered on what the field of mathematics education aspired for mathematics classroom work to be (e.g., the National Council of Teachers of Mathematics, 2000, Principles and Standards) than on the actual work that PSTs needed to be able to do. Much of the language now used to describe pedagogies of practice (e.g., approximations) was not yet in print, yet the ideas on how one could center the teaching of methods on the learning of practice were already under development (and arguably had been explored in the past, e.g., by Cooney, Davis, & Henderson, 1975).
The revised course included studying large instructional practices, like teaching through problems or promoting and managing classroom discourse, and smaller instructional practices, like concluding a lesson or explaining a procedure. The thought was that the values from mathematics education reform could be embedded in the study of those practices and that the practices had more face validity for PSTs in that they provided a way for them to relate to classroom instruction as something they would do (not something they would refrain from doing; see Chazan & Ball, 1999; Smith, 1996).
Among the strategies used to teach those instructional practices were demonstrations or video records of the enactment of those practices, decompositions of each practice that the first author had developed for use in the course (called rubrics at the time; see Herbst, 2011a, 2011b, 2011c; 2014, for current versions of some of those), and the approximation of those practices through the creation (mostly by script writing) and enactment of scenarios where the practices were rehearsed. In fall 2011 the course started to incorporate an online component through phasing in the use of Depict.
The fall 2011 secondary mathematics methods course focused on five practices: setting norms for mathematical work, explaining procedures, explaining concepts, promoting and managing students’ discourse, and assigning and reviewing students’ (home)work. Each of those core practices was decomposed into strategies that a novice might use to enact the practice. In the case of explaining a concept, those strategies included making connections to students’ prior knowledge and using multiple representations with deliberate connections, among others (Herbst, 2011a; see also Leinhardt & Steele, 2005).
In general, each instructional practice was covered for about 2 weeks in approximately the same manner. Prior to formally introducing the practice, PSTs were given articles from journals such as the NCTM’s Mathematics Teaching in the Middle Grades to assist the introduction of the practice by the teacher educator. During the first week studying the practice, the readings would be used to elicit the PSTs’ ideas about the practice. The class would also look at a representation of the practice, for example, a video or a demonstration, where the practice could be identified and named. Then the teacher educator would engage PSTs in decomposing the practice they had read about by asking them to discuss what it would take to enact this practice and make purposeful connections between what emerged from this discussion and the content of the written decomposition of practice.
The class would then use that decomposition of practice to discuss another representation of that practice. In some cases the representation of practice was a video of a teaching episode, and in others it was a live demonstration by the teacher educator. For example, when introducing setting mathematical norms, the teacher educator modeled the components of the practice while the PSTs behaved as students who acted out certain behaviors (both desirable and undesirable) that had been given to them on note cards at the beginning of class. When the course covered managing mathematical discourse, the teacher educator modeled the various moves in the decomposition of practice by leading a discussion on the mathematical work the PSTs had done to find the circle tangent to a line at a given point.
After an instructional practice had been introduced and represented, PSTs completed homework assignments that engaged them in approximating the practice. Just as a decomposition of practice would assist in inspecting the components of a practice, the problems chosen as approximations of practice would help PSTs practice particular strategies as well as put together the whole practice. An example of one such homework problem is the exercise shown in Figure 2, which was used to practice the setting and enforcing of norms for mathematical work.
| 2. In Ms. Taylor’s algebra I class, after some time solving equations students are given the problem, Solve 3(x+5) – 7 (5+x) = 7 (x+5) – 33 When asked to solve the problem on the board, Milton wrote the following and then returned to his seat. – 4x – 20 = 7x – 2 a. How could Ms. Taylor address the class apropos of Milton’s work so that she can help students develop a more flexible understanding of the norm that in algebra you should “show your work”? Script what she should say and practice saying it out loud. b. As you can tell from the choice of problem, Ms. Taylor must have been thinking about using this problem to teach students about the value of doing an algebraic substitution (e.g., let z = x +5) before solving. How could she prompt the class to think about the substitution so that students realize this would be a good thing for Milton to do or to have done? Script what she should say and practice saying it out loud.
|
| Figure 2. A homework problem on norms. |
When class reconvened the following week, time was allocated to rehearse selected problems from the previous week’s homework assignment. In small groups of four PSTs and one teacher educator or course assistant, PSTs would take turns enacting what they had scripted and receiving feedback from the teacher educators and their peers.
Two of the practices in the course (setting norms and explaining procedures) were studied in this way, while for the other three the software Depict was used in two ways—to create illustrations of the various strategies in which a practice was decomposed and to present and solve homework problems. As before, PSTs would rehearse what they had depicted in small groups of peers and an instructor.
It might be worthwhile to explain here what we think makes scripting a lesson, that is, creating dialog and other content for a lesson, into an approximation of practice, a part of practice-based teacher education. Approximations of practice are supposed to be opportunities to do the teaching rather than to write about it. To what extent would scripting the lines and actions for the teacher or the students be an approximation of teaching? At best, it is a tedious form of lesson planning, and at worst, it is an exercise in fancy. The work of coming up with precisely what to say and what to do, how long to speak and how to address the class, what examples to give, what tasks to assign, what to respond to and how to respond are aspects of practice that can be approximated in the context of lesson plays and scripts.
They do not cover all aspects of teaching practice. For example they do not eliminate the need for rehearsals, where PSTs can practice prosody, affect, tempo, and posture. They do not eliminate the need for clinical simulations, where PSTs can test the accuracy of their anticipations of student responses or the effectiveness of their follow-up questions. None of them eliminate the need for actual practice in front of actual students. If scripting is looked at only in that way, we are seeing the proverbial glass half empty. A way of seeing the proverbial glass half full is to notice how this activity can turn the homework for methods, an activity that might otherwise have only academic purposes (e.g., writing reading responses), into one that can combine academic and practical purposes (e.g., reading about students’ conceptions on a particular idea in order to anticipate how students might respond to a task on that idea).
Furthermore, in the context of a practice-based methods class where practices are decomposed into strategies and techniques, scripting scenarios is an activity that can bring those pieces together so as to connect them to the actual doing of practice and creating opportunities for PSTs to cope with additional, perhaps still tacit demands of practice. By the fall 2011 the first author had several years of experience using the writing of lesson scripts, not only to see its positive role approximating practice, but also its limitations. For example, when PSTs scripted lessons they involved few students and scripted mostly verbal actions, which would poorly prepare them to handle the multivocality and multimodality of real classrooms.
With the design of Depict students could use the images as feedback to realize that a whole class was available for action and that they could use multiple communication modalities. The dissertation study by Chen (2012), done under the direction of the first author, showed that Depict could do that; moreover, it showed that depicting a lesson was a better approximation of practice than talking through a lesson plan.
A Study that Encouraged the Use of Depict to Approximate Practice
Chen (2012) explored the differences in how preservice secondary mathematics teachers depicted a lesson on slope they had planned and how they described it in an interview where they were asked to talk through their plan. Chen recruited PSTs in their junior year and assigned them in pairs to one of two conditions: To talk through a lesson they had planned or to depict the lesson that they had planned. Chen found that the PSTs who used Depict attended to more instructional details than they had in their written lesson plans and as compared to those who were interviewed about their lesson plans. Once PSTs realized it was not sufficient to copy and paste from their lesson plans into Depict, they immersed themselves in the teachers role and unpacked the lesson (p. 219).
When using Depict, the PSTs in Chen’s (2012) study better specified the teacher’s work by attending to what the teacher could say in speech bubbles to explain tasks and present problems to students. In specifying the directions and explanations the teacher could give, the PSTs paid close attention to the language they used and the scaffolding students might need to understand the mathematical ideas at play. The PSTs who depicted their lesson anticipated a more active role for the teacher than those who talked about their lesson plan. These PSTs also attended to where in the room the teacher would stand and to what the teacher inscribed on the board to accompany instruction. For example, in Chen’s study two preservice teachers discussed needing to label the axes and units on a coordinate graph (p. 227), a detail they had not considered in their written plan.
When depicting the lesson, the PSTs that Chen (2012) observed realized they had to unpack the mathematical tasks they were assigning to students. They examined and specified the numbers in the examples they had intended to provide and attended to the mathematical appropriateness of the numbers. They also became more aware of temporal aspects of instruction, as they considered connections and transitions between mathematical tasks that had not been considered in their plan and were less present among those who talked through a lesson.
In addition to attending to the teacher’s work, preservice teachers depicting their lessons attended to students as individuals in the lesson. The PSTs who depicted a lesson gave students names and some personality traits and had students raise their hands to answer questions. Though neither the interview nor the Depict pairs of students had anticipated much student participation in their written lesson plans, those who depicted the lesson involved students significantly more often as individuals and as a class than those who talked about their lesson. When PSTs talked about their lessons, they always conceived of students as a class, acting as a group, rather than considering individual students who might say or do different things.
Chen’s (2012) work suggested a hypothesis that needs to be further tested: that the semiotic resources in Depict may help PSTs unpack and attend to important instructional details better than lesson planning or talking through a lesson plan. Since the evidence from Chen’s study was available in fall 2011, so we explored whether Depict could improve the homework in the methods class.
Approximating Practice Using Depict
We incorporated Depict as an online component of a face-to-face class during the second half of the semester. Instead of using the learning management system we normally use for course repository and assignment submissions, we asked students to sign up for a LessonSketch account and assigned them LessonSketch Experiences as homework. (Experience is the generic name used in LessonSketch for designed, self-contained sets of webpages including text and media. They can be course modules, assignments, assessments, research questionnaires, etc. Advanced users can create an agenda for an experience using LessonSketch‘s Plan tool and then create an Experience by putting together an agenda, participants, dates, and other parameters. See Herbst et al., 2013).
The homework experiences would ask PSTs to depict particular aspects of a practice they were studying and to attach their depictions to their answers to each Experience. When they came to class they would also spend time rehearsing what they had depicted.
Overview of the Homework Assignments
The homework assignments for three practices (explaining a concept, promoting and managing students’ discourse, and assigning and reviewing students’ work), taught in the fall 2011 secondary mathematics methods class, were done using Depict. Each practice was covered in two homework assignments, and each homework assignment contained several problems requiring PSTs to depict some aspect of the practice in a particular mathematical context. Homework assignments 6 and 7 provided practice on explaining concepts, 8 and 9 provided practice on promoting and managing students’ discourse, and 10 and 11 provided practice on assigning and reviewing students’ work.
With the exception of homework assignment 10, the homework assignments were all similarly structured. When participants opened the LessonSketch Experience they saw a welcome screen that described the assignment. For example, for Homework 9 they saw the screen in Figure 3.
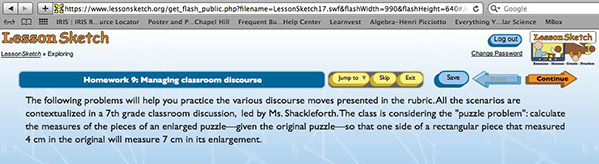 Figure 3. Introduction to Homework 9.
Figure 3. Introduction to Homework 9.
After the introduction, the PSTs were presented with three to four problems. Each problem was presented on a separate screen and started with a short (one- or two-sentence) description of a classroom context and, usually, an accompanying depiction (see Figure 4).
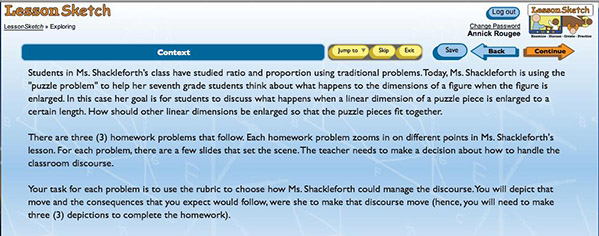 Figure 4. Context provided for a homework assignment.
Figure 4. Context provided for a homework assignment.
After reading about and viewing this classroom context, PSTs were given a short task around a particular aspect of the practice being studied (such as ranking possible discourse moves, writing what types of conceptual errors students might make, or writing what concerns they might have with the student work presented in the classroom scenario). These tasks were opportunities to brainstorm about the aspect of the instruction they would then be asked to depict. For example, in Homework 11 on reviewing students’ work, they were initially shown a student’s solution to a problem and asked whether they had any concerns with the work done, as shown in Figure 5.
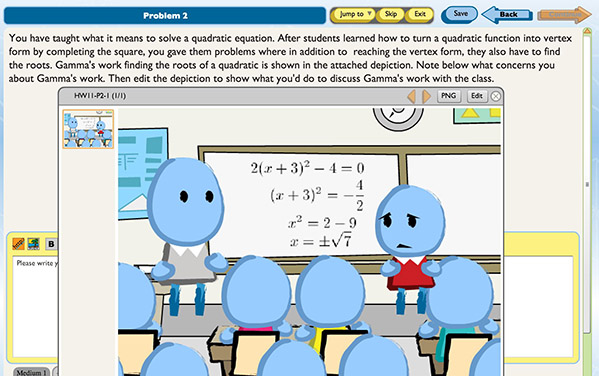 Figure 5. Work done by a student in an algebra class.
Figure 5. Work done by a student in an algebra class.
After PSTs had written about the concerns they might have with the student work presented in the depiction, they were asked to edit this scenario, depicting what they would do to discuss the student’s work with the class (see Figure 5). PSTs were to press the edit button, which would take them to an editable version of the depiction (see Figure 6), where they would be able to add frames to show what they would say and do apropos of the student’s work.
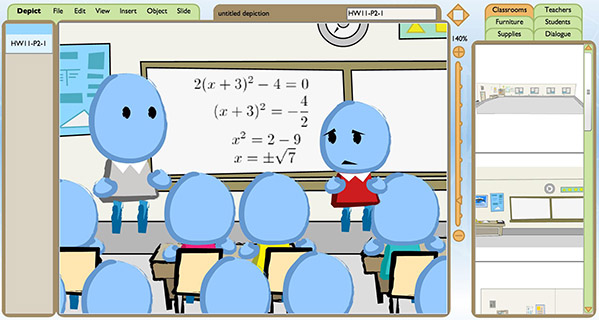 Figure 6. An editable Depict screen.
Figure 6. An editable Depict screen.
In general, each homework problem included the presentation of some classroom scenario (often including a depiction), and a short task to write about some aspect of instruction PSTs would later be asked to depict. They were then asked to edit the provided depiction to illustrate what they would say or do to address the aspect of instruction they were working on. When they edited the depiction, they would save it in their Resources folder inside their LessonSketch account, render it as a published depiction, and attach it to the homework assignment. When they were done with their homework assignment, the instructor could use the Annotate tool to make comments on the various questions they answered or on the frames of the depictions they had attached (Figure 7).
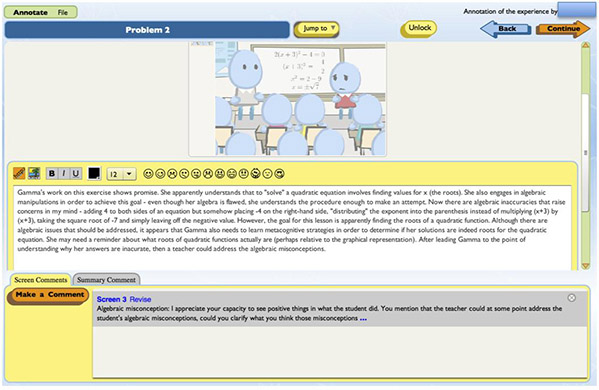 Figure 7. Using Annotate to give feedback on homework.
Figure 7. Using Annotate to give feedback on homework.
Figure 7 shows an example of an Annotate file where the top of the screen includes the text response of a PST to the question asked, and the panel in the bottom includes a comment by the teacher educator. An instructor can annotate each completed assignment, then share the annotation files with the PSTs using a shared folder in LessonSketch.
The Content of the Assignments
As noted, homework assignments would give PSTs the opportunity to practice various strategies related to instructional practices they were learning to do. The assignments done on LessonSketch were labeled Explaining Concepts, Promoting and Managing Students’ Discourse, and Assigning and Reviewing Students’ Work.
Homework assignments 8 and 9 were about promoting and managing classroom discourse. They were both situated in the context of a seventh-grade class. PSTs could see a five-frame depiction where the teacher shows a geometric puzzle on the board and indicates they would want to create an enlarged copy to donate to a homeless family shelter (see also Ghousseini, 2008). The teacher gives one of the dimensions of one piece of the enlarged puzzle (inspired in Brousseau’s, 1997, puzzle problem) and tells students that a rectangle piece that was 4 x 10 units long in the original will be enlarged so that the side that was 4 units long will be 7 units long. Students are asked to determine what other measures will be.
The PSTs could see that students in the depicted class have different ideas. One student volunteers an answer. PSTs were asked to consider and rank four potential discourse moves, from top choice to fourth choice that the teacher would have available at that moment in the scenario. Next PSTs were asked to edit the depiction, showing how they would make the discourse move they ranked as the top choice and possible consequences of this move.
Each of the problems in homework assignments 8 and 9 had the same flavor: The PSTs could see a segment of the puzzle lesson, which ends at a moment when the teacher needs to make a discourse management move. They were asked to consider various possible moves and to depict the one they would make and its possible consequences. Through these two assignments, PSTs had the chance to practice a range of discursive moves, which are described in Herbst (2011b), including revoicing, pressing for explanation, orienting, and others that they had been studying in class.
Homework assignments 10 and 11 were about selecting and reviewing students’ work, particularly students’ homework[a] . Homework 10 was situated in the context of a class where PSTs have taught linear functions and must construct a homework assignment. PSTs were provided with a list of 29 problems involving linear functions and asked to select a subset of 9 to 12 of these problems for homework, providing a justification for the selection. In the second part of Homework 10, PSTs were asked to narrow this 9-12 problem subset further to three problems that they considered essential to review in class and to depict how the teacher might announce and review those problems with the class. The third part of Homework 10 provided students’ work on some of the problems in the original bank of 29 problems. PSTs were asked to “identify three records of student work and depict how you would use those responses in future instruction.”
Homework 11 had the same structure as other assignments: The PSTs were situated in the context of an algebra class where they have assigned some problems on quadratic functions. Each of the three problems presented the PSTs with the scenario of a student putting up his or her work on the board; the work ranges in strategies used and errors exhibited (see Figure 6). The PSTs were asked to consider and depict how they would review the student’s work in public. Thus, the problems in homework assignments 10 and 11 gave PSTs the opportunity to practice strategies they had studied in the context of assigning and reviewing students’ work (Herbst, 2014), including balancing conceptual understanding and procedural fluency, expecting students to justify solutions to problems, and focusing on the mathematics at stake in each problem.
A Look in Detail to the Homework on Explaining a Concept
In class PSTs had received and discussed a decomposition of practice (Herbst, 2011a), which identified eight strategies for explaining a concept: problematizing the concept, connecting to prior knowledge, representing the concept, exemplifying the concept, identifying core components of the concept, identifying key errors with the concept, establishing the range and boundaries of the concept, and holding students accountable. The decomposition builds on Gaea Leinhardt’s work on instructional explanations (Leinhardt & Steele, 2005). The PSTs encountered exercises on Explaining a Concept in homework assignments 6 and 7, which were also the first assignments in which they would use Depict. (They had used other functionalities of LessonSketch previously.)
Homework 6 began with Problem 0, which provided an opportunity for PSTs to explore the features and capabilities of Depict. They were given requirements about the visual content of the depiction they would author (e.g., it had to include one frame with a student answering a question or making a comment, and it had to show use of the whiteboard), but PSTs were free to choose the mathematical content. [b]
In Problem 1, PSTs were ushered into Mr. Vince Gogh’s Algebra I class in which the concept of function is being introduced. The provided depiction contains two frames. In the first, Mr. Gogh is shown at the board telling the class, “OK, class, today we’re going to be talking about functions. In seventh grade you learned about linear functions. Now we are going to learn, in general, what a function is.” In the second, Mr. Gogh is pointing to and reading the definition of function on the board (“A function is a relation that assigns exactly one value in the range to each value in the domain.”). The PSTs were then asked to write about how Mr. Gogh might unpack this definition and describe what might be “important to say to help students understand what the definition means.”
Next, PSTs were provided with a five-frame depiction where frames 1 and 2 are identical to those given to set the context. Frames 3, 4 and 5 include some scaffolds for their work editing the depiction (a space on the board with a note asking them to insert something there and blank speech bubbles assigned to the teacher suggesting they type in text). This problem provides an opportunity to practice, in particular, how to identify core components of the concept and give examples.
In problem 2, PSTs were ushered into Ms. Clark’s Algebra II class, where students are learning about the properties of logarithms. The PSTs were given a two-frame depiction in which Ms. Clark introduces the properties of the logarithm of a product and the logarithm of a quotient (which she writes on the board) and tells her students that she wants to show them “errors that I often see people make when using logarithms and operations.” PSTs were asked to list common conceptual errors students might make with logarithms, and then they were asked to edit the depiction to show how they might point out these common errors to students.
Problem 3 took place in Mr. Little’s class where he has just defined the exponential function “y = ax” and “now wants to make clear to his students what needs to be true for the exponential function to be well-defined.” PSTs were given a three-frame depiction, in which Mr. Little is at the board reviewing the definition he has given of an exponential function (also on the board) and preparing to discuss when this function is well defined (e.g., a > 0).
After PSTs had viewed this depiction and written about which key points they would make clear to students so they would understand where the exponential function is well defined, they were asked to edit the depiction. Problem 2 was an opportunity to practice identifying key errors, and Problem 3 was an opportunity to practice establishing the range and boundaries of a concept. Other strategies were practiced in Homework 7.
In Homework 7, Problem 1 started by asking PSTs to write about how they would problematize the concept of inverse variation for their Algebra II class—what problem they think could be solved with inverse variation that they could present to their students before they learn about inverse variation. Then they were given a four-frame depiction to edit. The depiction begins with a frame in which the teacher is talking about direct variation and a general formula (y = ax) is on the board. The depiction then continues with a general formula for inverse variation on the white board:
y = ![]() .
.
PSTs were asked to edit the depiction and complete what the teacher should say and do to problematize the concept.
In Problem 2, PSTs were first asked to write about what prior knowledge geometry students might have that could be used to anchor an understanding of the concept of surface area of a pyramid. The PSTs were asked to edit a four-frame depiction to illustrate what they would do and say to the class to connect the concept of surface area of a pyramid to the students’ prior knowledge. The first frame in the provided depiction has the teacher introducing the day’s topic.
On the whiteboard, “Today’s Class: Surface Area of Pyramids” is written next to a two-dimensional representation of a regular, right, square pyramid. In the second frame, the teacher tells students they will be coming up with the formula for the surface area of a pyramid based on what they already know about surface area. In this frame, PSTs were cued to continue the teacher’s talk. The third frame contains a view of the class with two students having empty speech bubbles (to encourage PSTs to script speech for the students). The fourth frame had the beginning of the teacher talk (“So to calculate the surface area we would have to …”) and a prompt on the board for the PSTs to “write an expression for the surface area of a pyramid.”
Problem 3 in Homework 7 started by having PSTs write about two representations they could use to explain the concept of solving a system of linear equations to their Algebra I class. They had to edit a five-frame depiction to illustrate what they would say and do to explain what it means to solve a system of equations. The frames provided cues to include the linear functions and two representations on the board and add teacher talk in the speech bubbles. The fourth frame in the provided depiction was a view of the class with no speech bubbles included (though they could be added).
Homework 7 also included an extra credit problem that asked PSTs to attach a depiction they had created in class on how they would explain the meaning of the following:
![]() .
.
No further directions or classroom context were given in the extra credit problem. To illustrate what students do with those problems we take a special look at the answers to Problem 2 in Homework 7.
What PSTs Did to Connect a Concept to Students’ Prior Knowledge
In looking at the PSTs’ depictions, we paid attention to particular practical issues of teaching that the exercise enabled PSTs to experience or cope with. The exercise was predicated on the need to practice connecting with prior knowledge.
Different Ways of Connecting to Prior Knowledge
We observed a spectrum of putative ways of connecting to prior knowledge. These ranged from an extreme of starting with general and vague questions that tried to elicit prior knowledge to another extreme of giving more information then asking students for a specific piece of prior knowledge. For example Dudley (all names of individual PSTs are pseudonyms) had his cartoon teacher ask students for the definition of surface area and what surface area meant when dealing with shapes other than the pyramid. Other PSTs, such as Klo, had their cartoon teacher name a specific 3D object (e.g., the cube) and ask students what they remembered about finding the surface area of that object.
Seamus had his cartoon teacher remind the students how they had found the surface area of a cube and then asked them how they thought that applied to a pyramid. A fourth group of PSTs had their cartoon teacher ask the students what 2D shapes they could recognize in the pyramid. A fifth group had their cartoon teacher actually tell his students what shapes made up the faces of a pyramid and prompt students to recall the area formulas for those shapes.
As is evident from the range of responses, all PSTs thought they were connecting to prior knowledge. Yet, the ways in which they did so varied, with some making connections for the students and others trying to elicit the connections from students starting first with little support. Those who had started more broadly, such as Dudley, showed how the cartoon teacher could eventually elicit the various area formulas that students needed in order to understand what is involved in the surface area of the pyramid. In those scenarios he included more back-and-forth discussion with students (including some teacher revoicing).
While, by themselves, the depictions did not necessarily produce learning of how to connect to prior knowledge, they were the basis on which to produce such learning when, upon return to class the next week, PSTs rehearsed their scenarios with their peers. Similar conditions for learning could be created if PSTs were asked to contribute their depictions to a forum where others in the group could comment on them. Teacher educators could easily take this approach in LessonSketch, making the online work more interactive than we did in the fall 2011 course.
Attending to Students’ Thinking
Beyond revealing how PSTs understood the work of the particular practice they were learning (in this case, connecting to prior knowledge as part of explaining a concept), the depictions also showed how PSTs were coping with other elements of the practice of teaching. One of those is the existence of many students who could have varied ideas (correct and incorrect) of the mathematics at play. In considering how the PSTs elicited connections with prior knowledge, we could look at whether the PSTs anticipated that students might make erroneous connections and come up with ways of handling those errors. Only one of the 12 PST depictions for this problem included incorrect student responses. In all the others, students gave complete, correct answers, or the student answers built on each other to provide all the necessary information (e.g., one student would give the formula for the area of a rectangle and, in the same frame, another student would give the formula for the area of a triangle).
The incorrect responses only occurred in Dudley’s depiction: When the teacher asked for the shapes needed to build a pyramid, several students named 3D objects (cone and rectangular prism, one student said that obviously they needed a pyramid,) as opposed to 2D shapes. Dudley made his cartoon teacher patiently ask the students another question. What shapes did they see in the diagram of a pyramid, and when one student mentioned a square and some triangles, Dudley’s teacher asked the class whether they saw the same things.
The depictions also showed the extent to which PSTs involved many students and whether they were behaving similarly to students in actual classrooms. For example, two of the PSTs involved more than one student and had some of them engaged in behaviors or talk that made the scenario more complex. Over the totality of depictions made by PSTs through the course, the third author observed that about 30% of the talk PSTs scripted was attributed to students and that every PST created at least one depiction where students contributed at least one error.
This result was in stark contrast to the text-based representations (in response to homework assignments 1-5), where PSTs scripted almost exclusively teacher talk. Thus, it seems that, as a milieu for learning, Depict does provide the opportunity for PSTs to cope with the multivocality of students (the extent to which there are many students and they can say different things).
When left to their own devices, PSTs can choose to tone down the influence of such multivocality (for example, by making students complete each others’ sentences and together say what the cartoon teacher was hoping for). We conjecture that if depictions were created in groups or contributed to a forum, both of which can be done in LessonSketch, PSTs could easily call each other out for making scenarios unrealistic or edit each other’s scenarios to represent diverse student perspectives, thus making the experience a better environment in which to cope with multivocality.
The Mathematical Demands of Teaching
While the mathematics might be considered an element of explicit academic knowledge that PSTs study in mathematics courses, research has shown that such academic mathematics does not exhaust the mathematical demands of the work of teaching—PSTs need to learn mathematical knowledge for teaching (Ball, Lubienski, & Mewborn, 2001). Possibly, some of that mathematical knowledge for teaching is learned on the job; yet, approximations of practice such as depicting lessons may be environments in which PSTs get to cope with some of the mathematical demands of the work of teaching.
In looking at the surface area problem we could see how PSTs might come to terms with the need to handle two different concepts of height associated with the pyramid—the height of the pyramid itself, as the distance from the apex to the base, and the height of each triangle that made up the lateral surface of the pyramid as the distance from the apex to the side of the base contained in each of the triangles that formed the lateral surface. The latter is called slant height (see http://mathworld.wolfram.com/SlantHeight.html). One could call knowledge of the slant height an element of common content knowledge, though decisions of when to introduce it and how to explain it bring with them other domains of mathematical knowledge for teaching (e.g., which representations to use to show to students that a slant height is usually longer that the height or that slant heights can be of different lengths are elements of what Ball, Thames, & Phelps, 2008, called “knowledge of content and teaching”).
Of the 12 depictions PSTs submitted in response to this problem, six made no mention of the expression slant height or of the difference between it and the height of the pyramid. Some of them reduced the problem of explaining the surface area of the pyramid to one of calculating the areas of the base and the lateral faces as plane shapes. Although they explained to students the idea of surface area, they did not prepare students to read a formula that might include the slant height.
Three depictions used slant height in the formula for the surface area of the pyramid but never explained where in the figure it came from and how it is different from the height of the pyramid. In two of those the cartoon teacher called it slant height, and one mentioned that it is the height of the triangles. One PST’s depiction used slant height in the formula and labeled it in the figure but did not explain what it was. Klo labeled the slant height in the figure and implicitly addressed it. In her depiction, the cartoon teacher asked the students if “all four triangles have the same area?” to which a student responded,
I think so…because the base is the same for each triangle, because the base for each triangle is a side of the square…and the height of each triangle is the same, because if it weren’t then the pyramid would be lopsided.
Only Kate’s depiction addressed the difference between height and slant height explicitly: She had her cartoon teacher draw the slant height in the figure and represent it in the formula with a letter l and talk about it. In that depiction, a student asked, “Are all the triangles the same? They look different in the drawing.” Another student responded, “Of course they are the same. The bottom is one side of a square, and the tops are all at the same height.” In the following frame Kate’s cartoon teacher responds by labeling l (the slant height) and h on the diagram and explains that “although l > h, where h is the height from the ground to the top of the pyramid, we use l for the height of our triangles. l is also called slant height” (see Figure 8).
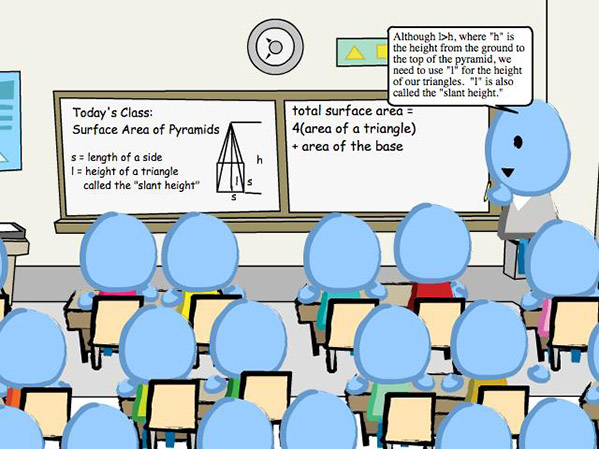 Figure 8. Kate’s depiction with the teacher speaking about the slant height.
Figure 8. Kate’s depiction with the teacher speaking about the slant height.
All three PSTs who included a diagram with slant height placed it in a location that would be problematic (the front face of the pyramid in perspective; see Figure 8) and might still confuse students about the difference between the height and slant height. A better option would, perhaps, be to draw the slant height on the face to the left or to the right, and contrast it with the height drawn inside the pyramid with a 90 degree angle marking to show it is perpendicular to the base (see how Wolfram’s Mathworld’s page shows it in http://mathworld.wolfram.com/SlantHeight.html).
Our point is that the need to explain the concept in detail created a context in which PSTs could be confronted with the need for mathematical knowledge for teaching, specifically when and how to represent slant height. Although the way the assignments were structured (completed individually) did not by itself create sufficient conditions for PSTs to learn that piece of mathematical knowledge for teaching, we believe that a slight revision, where students either cocreated a depiction or commented on and edited each other’s depiction could serve to bring those issues to consideration. PSTs might also be asked to cocreate or share their depiction with more experienced teachers, such as their mentors in a field placement, to engage in unpacking and learning the piece of the mathematical knowledge for teaching relevant to the mathematics at stake in a depiction.
The three issues discussed here apropos of Problem 2 in Homework 7—figuring out how to connect to prior knowledge, coping with the multivocality of students, and coping with the mathematical demands of instruction—are examples of how the work of creating a depiction, which is a case of constructing an artifact of teaching, can be part of an environment where instructional practice is learned in, from, and for practice. To the extent that depicting can enable such coping with elements of practice, it seems that it could be an appropriate approximation of practice. Clearly, lots of room for improvement exists in the design of how PSTs interact with Depict. If depictions are created individually and turned in to the instructor, they may have some value as opportunity to learn. If the depictions, however, are cocreated, annotated, or discussed and then revised, all of which can be done online before the next class session, this work could lead to even better development of knowledge of practice.
Conclusion
In this paper we have illustrated how a web-based storyboarding software can be used to provide opportunities for preservice mathematics teachers to learn teaching in practice. Specifically, we have argued and illustrated how the construction of representations of classroom scenarios using Depict, a storyboarding software, an application of the constructionist approach to learning proposed by Papert and Harel (1991), can have value for preservice teachers. We have illustrated what particular aspects of teaching could be learned by looking in some detail to responses to one problem selected from a homework assignment: Those aspects considered here—how to connect to prior knowledge, coping with the diversity of students’ thinking, and coping with the mathematical demands of instruction—are but a few examples of the opportunity to learn from approximating practice using this medium. We have also noted that the opportunity to learn from such approximation of practice can be increased if the storyboarding is complemented by other online activities, such as cocreating, sharing, annotating, and discussing that can make the feedback component more robust.
Notes
[a] The problems for homework assignments 10 and 11 were developed by the first author with the assistance of the third author as well as Justin Dimmel and Rachel Snider, who were part of the instructional team. Members of the team also collaborated in creating the materials needed to set up all the assignments in the LessonSketch platform.
[b] The problems on explaining concepts were developed by the first author in collaboration with Gloriana González and Adam Poetzel from the University of Illinois at Urbana-Champaign.
References
Ball, D. L., & Forzani, F. M. (2009). The work of teaching and the challenge for teacher education. Journal of Teacher Education, 60(5), 497-511.
Ball, D. L., Lubienski, S. T., & Mewborn, D. S. (2001). Research on teaching mathematics: The unsolved problem of teachers’ mathematical knowledge. In V. Richardson (Ed.), Handbook of research on teaching (4th ed., pp. 433-456). New York, NY: Macmillan.
Ball, D. L., Sleep, L., Boerst, T. A., & Bass, H. (2009). Combining the development of practice and the practice of development in teacher education. The Elementary School Journal, 109(5), 458-474.
Ball, D. L., Thames, M. H., & Phelps, G. (2008). Content knowledge for teaching: What makes it special? Journal of Teacher Education, 59(5), 389-407.
Boerst, T. A., Sleep, L., Ball, D. L., & Bass, H. (2011). Preparing teachers to lead mathematics discussions. Teachers College Record, 113(12), 2844-2877.
Boulos, M. N. K., Hetherington, L., & Wheeler, S. (2007). Second Life: An overview of the potential of 3-D virtual worlds in medical and health education. Health Information & Libraries Journal, 24(4), 233-245.
Brousseau, G., (1997). Theory of didactical situations in mathematics: Didactique des mathématiques, 1970-1990 (N. Balacheff, M. Cooper, R. Sutherland, & V. Warfield, Eds. and Trans.). Dordrecht, The Netherlands: Kluwer.
Chazan, D., & Ball, D. (1999). Beyond being told not to tell. For the learning of mathematics, 19(2), 2-10.
Chazan, D., & Herbst, P. (2012). Animations of classroom interaction: Expanding the boundaries of video records of practice. Teachers College Record, 114(3), 1-34.
Chen, C. (2012). Learning to teach from anticipating lessons through comic-based approximations of practice (Unpublished doctoral dissertation). The University of Michigan, Ann Arbor.
Chieu, V. M., & Herbst, P. (2011). Designing an intelligent teaching simulator for learning to teach by practicing. ZDM-The International Journal of Mathematics Education, 43(1), 105-117.
Christensen, R., Knezek, G., Tyler-Wood, T., & Gibson, D. (2011). simSchool: An online dynamic simulator for enhancing teacher preparation. International Journal of Learning Technology, 6(2), 201-220.
Cooney, T. J., Davis, E. J., & Henderson, K. B. (1975). Dynamics of teaching secondary school mathematics. Boston, MA: Houghton Mifflin.
Crespo, S., Oslund, J. A., & Parks, A. N. (2011). Imagining mathematics teaching practice: prospective teachers generate representations of a class discussion. ZDM- The International Journal of Mathematics Education, 43(1), 119-131.
Dieker, L. A., Rodriguez, J. A., Lignugaris, B., Hynes, M. C., & Hughes, C. E. (2014). The potential of simulated environments in teacher education current and future possibilities. Teacher Education and Special Education, 37(1), 21-33.
Doyle, W. (1986). Classroom organization and management. In M. Wittrock (Ed.), Handbook of research on teaching, (3rd. ed., pp. 392-431). New York, NY: Macmillan.
Dreyfus, H. L. (1980). Holism and hermeneutics. The Review of Metaphysics, 34(1), 3-23.
Ghousseini, H. N. (2008). Learning with routines: Preservice teachers learning to lead classroom mathematics discussions (Unpublished doctoral dissertation). The University of Michigan, Ann Arbor.
Ghousseini, H. N. & Herbst, P. (2014). Pedagogies of practice and opportunities to learn about classroom mathematics discussions. Manuscript submitted for publication.
Gibson, D. (2007, June). SimSchool – A complex systems framework for modelling teaching and learning. Paper presented to the National Educational Computing Conference, Atlanta, GA.
Grossman, P., Compton, C., Igra, D., Ronfeldt, M., Shahan, E., & Williamson, P. (2009). Teaching practice: A cross-professional perspective. Teachers College Record, 111(9), 2055-2100.
Grossman, P., Hammerness. K., & McDonald, M. (2009) Redefining teaching, Re-imagining teacher education. Teachers and Teaching: Theory and Practice 15, 273-289.
Hall, R. (2000). Video recording as theory. In A. Kelly & R. Lesh (Eds.), Handbook of research design in mathematics and science education (pp. 647-664). Mahwah, NJ: Erlbaum.
Hayes, A. T., Straub, C. L., Dieker, L. A., Hughes, C. E., & Hynes, M. C. (2013). Ludic learning: Exploration of TLE TeachLivE™ and effective teacher training. International Journal of Gaming and Computer-Mediated Simulations, 5(2), 20-33.
Herbst, P. (2011a). Explaining concepts and propositions (Rubric). Retrieved from the Deep Blue database at the University of Michigan: http://hdl.handle.net/2027.42/84658
Herbst, P. (2011b). Promoting and managing students’ discourse (Rubric). Retrieved from the Deep Blue database at the University of Michigan: http://hdl.handle.net/2027.42/84368
Herbst, P. (2011c). Setting mathematical norms. Retrieved from the Deep Blue database at the University of Michigan: http://hdl.handle.net/2027.42/101119
Herbst, P. (2014). Assigning and reviewing students’ work: A decomposition of practice. Retrieved from Deep Blue database at the University of Michigan: http://hdl.handle.net/2027.42/102572
Herbst, P., Aaron, W., & Chieu, V. M. (2013). LessonSketch: An environment for teachers to examine mathematical practice and learn about its standards. In D. Polly (Ed.), Common Core Mathematics Standards and implementing digital technologies (pp. 281-294). Hershey, PA: IGI Global.
Herbst, P., Chazan, D., Chen, C., Chieu, V.M., & Weiss, M. (2011). Using comics-based representations of teaching, and technology, to bring practice to teacher education courses. ZDM—The International Journal of Mathematics Education, 43(1), 91–103.
Herbst, P., & Chieu, V. M. (2011). Depict: A tool to represent classroom scenarios (Technical report). Retrieved from the Deep Blue database at the University of Michigan: http://hdl.handle.net/2027.42/87949
Herbst, P., Nachlieli, T., & Chazan, D. (2011). Studying the practical rationality of mathematics teaching: What goes into “installing” a theorem in geometry? Cognition and Instruction, 29(2), 1–38.
Kiesler, D. J. (1983). The 1982 interpersonal circle: A taxonomy for complementarity in human transactions. Psychological Review, 90, 185-214.
Lampert, M. (2010). Learning teaching in, from, and for practice: What do we mean? Journal of Teacher Education, 61(1-2), 21-34.
Lampert, M., Franke, M. L., Kazemi, E., Ghousseini, H., Turrou, A. C., Beasley, H., … & Crowe, K. (2013). Keeping it complex: Using rehearsals to support novice teacher learning of ambitious teaching. Journal of Teacher Education, 64(3), 226-243.
Lane, J. L., Slavin, S., & Ziv, A. (2001). Simulation in medical education: A review. Simulation & Gaming, 32(3), 297-314.
Leinhardt, G., & Steele, M. (2005). Seeing the complexity of standing to the side: Instructional dialogues. Cognition and Instruction, 23(1), 87-163
Lonn, S., & Teasley, S. D. (2009). Saving time or innovating practice: Investigating perceptions and uses of Learning management systems. Computers and Education, 53(3), 686-694.
Maloney, J., Resnick, M., Rusk, N., Silverman, B., & Eastmond, E. (2010). The Scratch programming language and environment. ACM Transactions on Computing Education, 10(4), 16.
McCrae, R., & Costa, P. (1996). Toward a new generation of personality theories: Theoretical contexts for the five-factor model. In J. S. Wiggins (Ed.), The five-factor model of personality: Theoretical perspectives (pp. 51-87). New York, NY: Guilford.
Mori, M. (1970). The uncanny valley. Energy, 7(4), 33-35.
Morris, A. K., & Hiebert, J. (2011). Creating shared instructional products: An alternative approach to improving teaching. Educational Researcher, 40(1), 5-14.
National Council of Teachers of Mathematics. (2000). Principles and standards for school mathematics. Reston, VA: National Council of Teachers of Mathematics.
Olive, J. (1991). Logo programming and geometric understanding: An in-depth study. Journal for Research in Mathematics Education, 22(2), 90-111.
Ören, T. I. (2009). Modeling and simulation: A comprehensive and integrative view. In L. Yilmaz & T. I. Ören (eds.), Agent-directed simulation and systems engineering (pp. 3-36). Berlin, Germany: Wiley Series in Systems Engineering and Management.
Papert, S. (1980). Mindstorms: Children, computers, and powerful ideas. New York, NY: Basic Books.
Papert, S. (1993). The children’s machine: Rethinking school in the age of the computer. New York, NY: Basic Books.
Papert, S., & Harel, I. (1991). Constructionism. Norwood, NJ: Ablex.
Rieber, L. P. (1996). Seriously considering play: Designing interactive learning environments based on the blending of microworlds, simulations, and games. Educational Technology Research & Development, 44(2), 43-58.
Ryle, G. (1945). Knowing how and knowing that: The presidential address. Proceedings of the Aristotelian Society (Vol. 46, pp. 1-16). New York, NY: Wiley
Short, D. (2012). Teaching scientific concepts using a virtual world—Minecraft. Teaching Science, 58(3), 55.
Smith III, J. P. (1996). Efficacy and teaching mathematics by telling: A challenge for reform. Journal for Research in Mathematics Education, 27(4), 387-402.
Zazkis, R., Sinclair, N., & Liljedahl, P. (2013). Lesson play in mathematics education: A tool for research and professional development. New York, NY: Springer.
Zhang, D., Zhou, L., Briggs, R. O., & Nunamaker Jr., J. F. (2006). Instructional video in e-learning: Assessing the impact of interactive video on learning effectiveness. Information & Management, 43(1), 15-27.
Zibit, M., & Gibson, D. (2005). simSchool: The game of teaching. Journal of Online Education, 11(6).
Author Notes
The work reported here has been done with the support of National Science Foundation grants and DRL- 0918425 to P. Herbst and D. Chazan. All opinions are those of the authors and do not necessarily represent the views of the Foundation. The design and development of the secondary methods course approach described here benefitted from conversations with Hala Ghousseini, Gloriana González, and Lawrence Clark. The authors acknowledge valuable comments to earlier drafts by Daniel Chazan and Vilma Mesa.
All graphics assets from screenshots of the LessonSketch platform are ©The Regents of the University of Michigan, used with permission.
Patricio Herbst
University of Michigan
610 East University Ave. #4119
Ann Arbor, MI 48109-1259
Email: [email protected]
Vu-Minh Chieu
University of Michigan
Email: [email protected]
Annick Rougee
University of Michigan
Email: [email protected]
![]()