Among the wide variety of skills necessary for secondary mathematics teachers to be successful in the classroom, a deep understanding of mathematical content is perhaps preeminent. In its report The Mathematical Education of Teachers, the Conference Board of the Mathematical Sciences (2001) recommended that, in addition to completing the equivalent of an undergraduate mathematics major, prospective high school teachers be required to complete a capstone class “connecting their college mathematics courses with high school mathematics” (p. 8).
At the same time, the ability to use technology effectively to enhance mathematics teaching is becoming increasingly important in secondary school classrooms. Technology is playing an ever more prominent role in education and especially mathematics education. The National Council of Teachers of Mathematics (NCTM, 2000) included technology as one of the six principles of school mathematics (along with equity, curriculum, teaching, learning, and assessment): “Technology is essential in teaching and learning mathematics; it influences the mathematics that is taught and enhances students’ learning” (p. 24).
At Utah State University (USU), we offer a course that combines the goals of a capstone mathematics experience and those of a technology course for future teachers. Through experiences with the course, we have identified several considerations for a program designed to prepare future mathematics teachers to teach effectively with technology:
- Technology skills should be taught as preservice teachers investigate mathematical principles.
- The nature and availability of technological tools changes rapidly and this dynamism should be reflected in course content.
- Upperclass preservice teachers have a great deal of mathematical and pedagogical sophistication, and this expertise can be used to motivate and direct their learning about technology, pedagogy, and mathematics.
Each year about 40 students graduate from the mathematics education program at USU. In addition to the general mathematics and education course work required of them, mathematics education students complete a mathematics and technology course offered through the mathematics department. In this course, entitled Capstone Mathematics, Statistics, and Technology for Teachers, students learn to use technology as they examine secondary mathematics topics from an advanced standpoint. Students are introduced to technology through instructor-led activities in which they investigate mathematical content. They also lead the class in authentic mathematics learning activities focusing on employing technology to increase understanding of secondary mathematics topics.
Current Strategies for Teaching Mathematics With Technology
An NCTM (2008) statement on the role of technology in mathematics education asserted that “with guidance from effective mathematics teachers, students at different levels can use [technology] to support and extend mathematical reasoning and sense making, gain access to mathematical content and problem-solving contexts, and enhance computational fluency” (para. 1). Technology can be effective in enhancing student learning, and many educators are using innovative strategies and tools to exploit technology in mathematics instruction.
Software available for teaching mathematics can be grouped into five categories (Kurz, Middleton, & Yanik, 2005):
- Review and practice software – tools used to reinforce familiar material (e.g., multiplication concentration). (Editor’s note: Website URLs can be found in the Resources section at the end of this paper.)
- General software – resources that are applicable across a variety of mathematical topics (e.g., GeoGebra).
- Specific software – applications that target a particular mathematical topic (e.g., an applet that illustrates the law of averages).
- Environmental software – tools that provide a contextual setting and often emphasize real-world application (e.g., Lemonade Stand).
- Communication software – resources for sharing and displaying information (such as blogs and wikis).
A wealth of software resources in each of these categories is easily accessible and often freely available on the Internet.
In addition to software resources and standard tools such as graphing calculators, educators are exploiting a variety of technological tools in mathematics instruction, including cell phones (see Davis, 2010; Valk, Rashid & Elder, 2010), GPS devices (see Nord, Jabon, & Nord, 1997), and mobile data collection units (see Boast, 2000). The iPod touch has been adopted in some classrooms as a means of getting technology into the hands of all students (Auchincloss & McIntyre, 2008; Banister, 2010). Moreover, numerous sites on the Internet are devoted to giving teachers access to materials that will promote appropriate use of software and technological resources in mathematics teaching and learning (e.g., Teacherlink, Henri Piciotto’s Math Education Page, and MathTools).
Technology is used for classroom demonstrations and explorations, to stimulate group work, and to facilitate homework assignments. Because technology is interesting to many students, it can be used to motivate and engage the students, as well as to foster mathematical investigations and increase understanding.
Technology resources are increasingly accessible in secondary math classrooms. In 2009, the National Center for Education Statistics reported that 95% of all secondary public school teachers in the U.S. had computers in their classrooms for everyday use, and 94% had classroom Internet access (Gray, Thomas, & Lewis, 2010). Nevertheless, the same survey found that 16% of secondary teachers reported never using the computers during classroom instructional time and 23% reported using computers only rarely. Thus, though many educators have successfully integrated technology into classroom teaching, many teachers are still hesitant to do so. An important task for teacher preparation programs is to provide future teachers with the skills they need to incorporate technology effectively into mathematics instruction.
Integrating Objectives in Mathematics and Technology
The objectives of the capstone technology course are that the preservice teachers will learn to employ computer-based technologies for analyzing mathematical content from secondary school curricula; that they will design, present, and assess the effectiveness of technology-enhanced mathematical learning activities; and that they will analyze mathematical content from secondary school curricula to interrelate topics, construct critical concepts, discover why relationships exist, discover why certain algorithms work, and apply useful topics to address real-life problems. These goals are complementary–mathematical investigations motivate and provide a setting for learning to use technology, while the use of technology facilitates examination of mathematical topics at a deeper level than would otherwise be possible.
In one activity from the capstone-technology course, students use an applet (Measuring Error in a Linear Model from the electronic examples of the NCTM, 2000, Principles and Standards) to investigate methods of identifying a line of best fit for a set of bivariate data. In this activity, students propose and examine a variety of methods for identifying the best fit line such as eyeballing it, finding a balanced line, such that the same number of points are above and below it, minimizing the sum of the shortest distances from each point to the line, looking at the sum of the absolute vertical distances from the points to the line, and using the sum of the squared vertical distances.
The first two methods are accessible to algebra students learning to graph, interpret, and find equations for lines. However, there is not a natural way to quantify the error associated with the lines in these cases, nor is it obvious how one would develop a systematic procedure for finding the line. The shortest distance, absolute distance, and squared distance methods of identifying the best fit line can be used more easily to quantify error. Preservice teachers can draw on their higher level mathematics knowledge to investigate these three methods.
Using the applet, students manipulate a set of points and a summary line in a scatter plot (see Figure 1). The applet can display the error as the sum of the shortest distances, absolute distances, or squared distances from each point to the line. Students can investigate relationships between lines obtained using each of these methods of describing the error, they can examine how each of the methods is affected by individual data points, and they can look at the influence of extreme values.
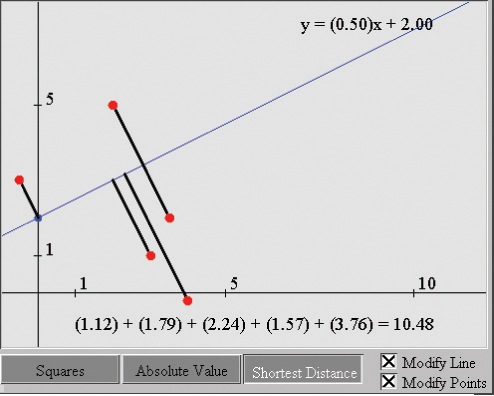 Figure 1. An applet for investigating methods of identifying a line of best fit.
Figure 1. An applet for investigating methods of identifying a line of best fit.
Based on these investigations, students choose a method of identifying the best fit line and develop an expression for the total error associated with the method. They then derive the slope and intercept parameters of the line. It quickly becomes apparent to students that the least squares line most easily yields the desired parameters. The activity gives insight into development of statistical methods and understanding of line of best fit. The applet facilitates visualization, manipulation of the data points and summary lines, and rapid computation. The user can then compare the methods of identifying a best fit line in a way that would not be possible with pencil and paper.
When technology is introduced to students in the context of a mathematical problem, the instructor can model appropriate use of technology and motivate subsequent lessons to teach specific skills with the tool. Students see how technology can foster comprehension and problem solving.
Adapting to Technological Innovation
In the Technology Position Statement of the Association of Mathematics Teacher Educators (AMTE, 2006), the authors contended that the use of technology “to improve the learning of mathematics at all levels, [will prepare students] to use technology appropriately, fluently, and efficiently to do mathematics in the techno-rich environments in which they will study and work in the future” (p. 1). However, the “techno-rich environments” of the future will likely include technologies very different from those now in use. The collection of available technologies for teaching and learning mathematics is constantly changing and expanding. Whereas graphing calculators first began appearing in high school mathematics classrooms only around 20 years ago, today the nearly ubiquitous calculators are complemented by personal computers, interactive whiteboards, the Internet, mobile data collection units, and educational software. Moreover, more tools and software packages are continually added to the mix.
A course aimed at training teachers in the use of current tools without also preparing them to take advantage of technological developments runs the risks of teaching information that may be obsolete in a matter of a few years and of failing to prepare teachers to make the most of technology that will impact student learning in the future. Since people have a tendency to stick with the familiar, preservice teachers must not only learn to value technology and to use it well, but also learn to identify and appropriately use new applications for mathematics teaching. Teacher-educators have the opportunity and the responsibility to prepare preservice teachers to adapt to the technological climate of rapid change and innovation and to take advantage of the ever-increasing array of resources available to them.
An understanding of how to use technology appropriately for teaching and learning mathematics is foundational to any effort to keep up with evolving technological resources. Describing the skills that teachers must possess to adapt to evolving technology, Thompson and Kersaint (2002) said, “Teachers must be prepared to make decisions about various technologies, must be taught new skills for working with them in classrooms, and must be able to address many of the pedagogical issues that arise when using technology” (p. 137).
Teachers must have an understanding of the role of technology in mathematics education, knowledge of how effectively to use technology to enhance learning of mathematics, skill in the use of specific tools and software, understanding of how students learn with technology (Lee & Hollebrands, 2008), positive experiences learning with technology, and experience planning and leading technology-enhanced lessons on their own account (Powers & Blubaugh, 2005). Furthermore, in order to take advantage of technological innovation, teachers must be aware of the vast array of tools available to them and they must be confident to seek out and learn to use technologies that will help them to meet their curricular goals.
Students become aware of the many ways in which technology can impact mathematics teaching and learning through exposure to diverse resources. These resources may include online math forums and journals, applets, dynamic geometry packages, graphing calculators, and tools for lesson preparation. Such exposure leads to an expanded view of the role of technology in mathematics teaching and learning, stimulates excitement for using technology, and primes future teachers to look for and take advantage of new resources. In our course, students create webpages in html, investigate online resources, work with graphing calculators and Geometer’s Sketchpad, manipulate spreadsheets, and learn to use GeoGebra. Students are exposed to other tools and technologies through class discussions and student presentations.
The breadth of resources addressed in the course necessarily limits the depth of what can be covered in class on any particular tool. Therefore, students are expected use the resources outside of class to increase their skill; at the same time they become more comfortable bringing themselves up to speed with new technology. With each application discussed in the capstone technology course, students create an original product such as an applet (in GeoGebra or Geometer’s Sketchpad), a program (with a graphing calculator), or a webpage. They are expected to refer to tutorials, help packages, and each other to move beyond the material covered explicitly in classroom instruction.
As students investigate these resources, their excitement about technology increases, they gain confidence in their ability to learn to use novel resources, and they are better prepared to adapt technology to address their instructional needs in the future. Webpages created by students from the capstone-technology course and containing links to recent student projects can be viewed at http://www.math.usu.edu/~schneit/Math5010/
In the capstone technology course, students are introduced to the software package GeoGebra through an investigation of conic sections and learn to use it to work with functions and basic constructions. They then use GeoGebra to create an original applet. Through this assignment, they become more familiar with the software’s capabilities. Figure 2 displays a student-created GeoGebra applet. The student moved well beyond the work she had done in class with functions to develop an applet intended to guide the user to identify patterns in Pascal’s Triangle (Bischoff, 2009).
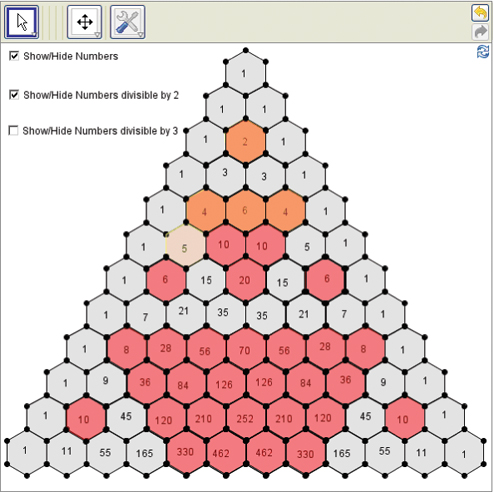
Figure 2. An Interactive Pascal’s Triangle constructed in GeoGebra.
The applet has a tool for highlighting hexagons that can be used to identify those that match a given criterion, such as divisible by 2 or by 3. Numbers divisible by 2 can also be automatically highlighted when the appropriate checkbox is marked. The user-highlighted numbers are displayed in yellow and the automatically highlighted numbers in red. Numbers that have been selected by the user and the applet are shown in orange.
In addition to conducting personal investigations into the technologies introduced in our course, students have opportunities to identify new resources. Students seek novel applications in a class of known technologies. For instance, having used an online applet in a mathematics learning activity and discussed general applet features and functionality, students search the Internet for applet resources that address a particular topic of their choosing. In this way, they become aware of the multiplicity of such resources available and are not limited by the strictly familiar. Students are also expected to seek out, to learn to use, and to present to the class an unfamiliar software or technology. In their presentations, they describe the use and functionality of the technology as well as how the tool could be used to increase the mathematical understanding of their students.
The experience gives them the opportunity independently to figure out how to use a new resource and increases their confidence to be able to do the same in the future. It requires them to apply their knowledge of appropriate pedagogy for teaching with technology to identify resources that would benefit the mathematical learning of their future students. Finding a novel utility fuels excitement for technology and fosters a tip of the iceberg mentality in which students begin to realize the vast array of technologies available to them.
Through this assignment, students have identified a variety of software packages and Internet resources. They have also become familiar with media such as podcasts and clickers. Recently, students presented the dynamic geometry package Cinderella and introduced many of the software features such as construction tools and animations. Figure 3 shows an applet, created by the students, in which they constructed a circle in various geometries. In addition to learning to do basic constructions, the students learned about Cinderella’s virtual laboratory capabilities, with which users can model forces and look at the behavior of springs.
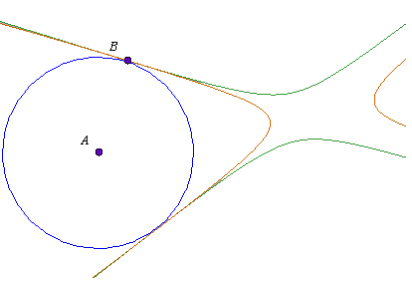 Figure 3. Circles in different geometries constructed in Cinderella.
Figure 3. Circles in different geometries constructed in Cinderella.
Just as technology has an essential role in secondary mathematics education, it has an essential role in secondary mathematics teacher education. The techno-rich environments of the future might look very different from the pc-driven world of today; nevertheless, preservice teachers can be prepared to excel and prosper with a broad knowledge base to draw from and opportunities to seek out and learn to use new resources.
Fostering Collaboration
The majority of our mathematics education majors take the capstone technology course in the second semester of their junior year or the first semester of their senior year, just prior to student teaching. At that point, they have completed numerous college-level courses in both mathematics and pedagogy. Many of them have worked as mathematics tutors or have led recitation sections of lower level mathematics classes; thus, the level of mathematical and pedagogical knowledge of the students is high. Our course structure capitalizes on the expertise of the students, fostering a highly collaborative learning environment. Students work together to investigate mathematical content. They teach each other features of various technologies, both formally through presentations and informally as they see the innovations of their peers and share ideas. Students discuss readings (in particular, see Garofalo, Drier, Harper, Timmerman, & Shockey, 2000; Kimmins and Bouldin, 1996) and use these to establish jointly a framework for development of technology-enhanced mathematics activities. They also work together to develop and engage their classmates in technology-enhanced learning activities.
Student-led mathematics lessons are an integral part of the course. In these activities, students employ technology in a mathematics lesson addressing topics from secondary mathematics in a way that guides their classmates to interconnect topics, construct concepts, discover relationships, or make sense of algorithms. Thus, though the content is from the secondary curriculum, the student presenters approach it in way that motivates an authentic learning experience for their peers and that can be adapted for use in their own future classrooms. Presentations have addressed topics such as inequalities, applications of matrices in cryptography, recursion functions, math in music, and connecting the unit circle to graphs of trigonometric functions.
In one presentation, students prepared an activity to introduce geometric representation of fraction multiplication. In this lesson the presenters led a discussion about common misconceptions and confusions about fractions and discussed how the geometric representation clarifies them. They introduced the pattern block applet from the National Library of Virtual Manipulatives and worked with their colleagues to examine how fraction multiplication might be represented geometrically (see Figure 4).
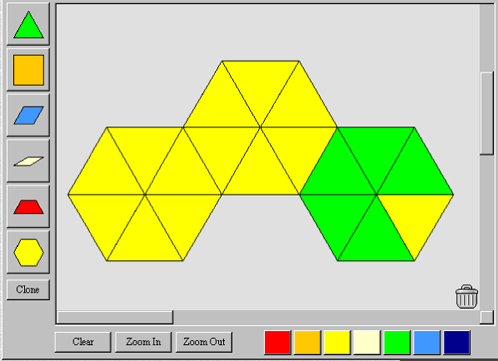
Figure 4. A geometric representation of the multiplication of 5/6 by 1/3.
The student-led presentations motivate both the presenters and their classmates to look at familiar concepts in unfamiliar ways and to make connections between topics and subjects. Students further develop their technology skills as they prepare appropriate resources for addressing the mathematical content of their lessons and as they work with the resources developed and identified by their peers.
These student-led presentations together with class discussions and engagement with technology in mathematical tasks comprise a program of experience, discussion, and practice that can help to develop students’ technology, pedagogy, and content knowledge (TPACK). According to Neiss (2005), TPACK (or technology pedagogical content knowledge, as she referred to it at that time)
is the integration of the development of knowledge of subject matter with the development of technology and of knowledge of teaching and learning. And it is this integration of the different domains that supports teachers in teaching their subject matter with technology. (p. 510)
When these domains are integrated in preservice teacher preparation so that teachers learn their subject matter with technology, they can refer to their own experience to reflect on the ways in which learning with technology influences mathematical thinking, to consider the ways in which technology supports student understanding, and to evaluate advantages and disadvantages of using technology in their teaching. These reflections, supplemented with technology lessons, readings, and classroom discussions can build understanding of principles of effective use of technology in mathematics teaching and learning.
When preservice teachers then practice these principles by designing and presenting lessons to their classmates, they synthesize what they have learned to make decisions about the role of technology in the lesson development and the ways in which it will influence student learning. These considerations contribute to the preservice teachers’ abilities to make decisions about the appropriate use of technology as they teach mathematics to their own students.
Implications for Teacher Education
A course or program that aims to prepare preservice teachers to adapt to and to take advantage of changing technology needs to be adaptable and dynamic itself. With this goal in mind, teaching a technology class that focuses solely on graphing calculators or Geometer’s Sketchpad is probably not sufficient, nor is teaching courses the same way year after year. Though calculators and dynamic geometry software continue to be important, innovation and adaptability can be emphasized by introducing new technologies and using them to access exciting mathematics in novel ways.
The preservice teachers themselves can help teacher educators keep course content on technology up to date; just as we can capitalize on their mathematics and pedagogical expertise to enrich the classroom learning experience, we can take advantage of their technological expertise to ensure that the course content moves forward. As students seek out new technological resources and present them to their classmates, we can incorporate the best of these into the course content of subsequent semesters. We can learn from our students and carry that knowledge to the next class. As we expand our own horizons, we model attitudes and behaviors that will help our students be successful users of technology in the future.
Conclusion
To obtain the greatest benefits from technology resources in mathematics teaching, preservice teachers need a deep understanding of secondary level mathematics content, they need to be able to integrate technology effectively into mathematics teaching, and they need to be able to adapt to evolving technology. Technology skills can be most effectively addressed in the context of collaborative mathematics learning experiences.
Learning to use technology while working with peers to investigate mathematics motivates technology use, stimulates interest, and gives students firsthand knowledge of the advantages of learning with technology. It enables the instructor to motivate and model appropriate technology use and allows students to benefit from the expertise of their classmates.
As students investigate technology capabilities individually and share their knowledge with their peers, they develop skills and attitudes needed to adapt to the evolving technology climate, they recognize the diversity of resources available to them, and they gain confidence in their ability to seek out new resources and learn to use them effectively. Most importantly, they are prepared to take best advantage of educational technologies for fostering the mathematical understanding of their students both now and in the future.
References
Association of Mathematics Teacher Educators. (2006). Preparing teachers to use technology to enhance the learning of mathematics: A position of the Association of Mathematics Teacher Educators. Retrieved from http://www.amte.net/sites/all/themes/amte/resources/AMTETechnologyPositionStatement.pdf
Auchincloss, C., & McIntyre, T. (2008). iPod “Teach”: Increased access to technological learning supports through the use of the iPod Touch. Journal of Special Education Technology, 23(2), 45-49.
Banister, S. (2010). Integrating the iPod Touch in K–12 education: Visions and vices. Computers in the Schools, 27(2), 121-131.
Bischoff, J. (2009). Pascal’s triangle and patterns. Retrieved from the Utah State University website: http://schneiter.math.usu.edu/math5010/Fall2009/JoAnna’sPage/Pascal/myapp.html
Boast, S. (2000). Mathematics activities for calculator based labs (CBLs) and TI-83 graphing calculators. Retrieved from the Fairmount Center for Science and Mathematics Education website: http://www.webs.wichita.edu/facsme/cbl/mathapp.htm
Conference Board of the Mathematical Sciences. (2001). The mathematics education of teachers. Washington, DC: American Mathematical Society & Mathematical Association of America.
Davis, M.R. (2010). Solving algebra on smartphones. Technology Counts, 29(26), 20-23.
Garofalo, J., Drier, H., Harper, S., Timmerman, M.A., & Shockey, T. (2000). Promoting appropriate uses of technology in mathematics teacher preparation. Contemporary Issues in Technology and Teacher Education, 1(1). Retrieved from https://citejournal.org/vol1/iss1/currentissues/mathematics/article1.htm
Gray, L., Thomas, N., & Lewis, L. (2010). Teachers’ use of educational technology in U.S. public schools: 2009 (NCES 2010-040). Washington, DC: National Center for Education Statistics, Institute of Education Sciences, U.S. Department of Education.
Kimmins, D., & Bouldin, E. (1996). Making mathematics come alive with technology. In Proceedings of the 11th annual Instructional Technology Conference, Murfreesboro, TN. Retrieved from http://frank.mtsu.edu/~itconf/papers96/kimmins.html
Kurz, T. L., Middleton, J. A., & Yanik, H. B. (2005). A taxonomy of software for mathematics instruction. Contemporary Issues in Technology and Teacher Education, 5(2). Retrieved from https://citejournal.org/vol5/iss2/mathematics/article1.cfm
Lee, H., & Hollebrands, K. (2008). Preparing to teach mathematics with technology: An integrated approach to developing technological pedagogical content knowledge. Contemporary Issues in Technology and Teacher Education, 8(4). Retrieved from https://citejournal.org/vol8/iss4/mathematics/article1.cfm
National Council of Teachers of Mathematics. (2000). Principles and standards for school mathematics. Reston, VA: Author.
National Council of Teachers of Mathematics. (2008). The role of technology in the teaching and learning of mathematics. Retrieved from http://www.nctm.org/about/content.aspx?id=14233
Niess, M. L. (2005). Preparing teachers to teach science and mathematics with technology: Developing a technology pedagogical content knowledge. Teaching and Teacher Education, 21(5),509-523.
Nord, G. D., Jabon, D., & Nord, J. (1997). The mathematics of the Global Positioning System. Mathmatics Teacher, 90, 455-462.
Powers, R., & Blubaugh, W. (2005). Technology in mathematics education: Preparing teachers for the future. Contemporary Issues in Technology and Teacher Education, 5(3/4). Retrieved from https://citejournal.org/vol5/iss3/mathematics/article1.cfm
Thompson, D., & Kersaint, G. (2002). Editorial: Continuing the dialogue on technology and mathematics teacher education. Contemporary Issues in Technology and Teacher Education, 2(2). Retrieved from https://citejournal.org/vol2/iss2/mathematics/article1.cfm
Valk J., Rashid, A.T., & Elder L. (2010). Using mobile phones to improve educational outcomes: An analysis of evidence from Asia. International Review of Research in Open and Distance Learning, 11(1), 117-140.
Author Notes:
Kady Schneiter
Utah State University
Email: [email protected]
Cinderella – http://www.cinderella.de/tiki-index.php
GeoGebra – http://www.geogebra.org/cms/
Henri Piciotto’s Math Education Page – http://www.mathedpage.org
Law of Averages – http://www.math.usu.edu/~schneit/CTIS/LawOfAverages
Lemonade Stand – http://coolmath-games.com/lemonade
MathTools – http://mathforum.org/mathtools
Measuring Error in a Linear Model – http://standards.nctm.org/document/eexamples/chap7/7.4
Multiplication Concentration – http://www.aplusmath.com/Games/Concentration/Multiplication_Concentration.html
Pascal’s Triangle – http://schneiter.math.usu.edu/math5010/Fall2009/JoAnna’sPage/Pascal/myapp.html
Pattern Block applet (National Library of Virtual Manipulatives) – http://nlvm.usu.edu/en/nav/frames_asid_169_g_1_t_2.html?open=activities&from=topic_t_2.html
Teacherlink – http://teacherlink.org
![]()