As we move into the teen years of the 21st century, advanced digital technologies are significantly changing the nature of the culture as well as the way mathematicians think about and do mathematics. Has this change shifted how students today are or should be learning mathematics? Are they using today’s technologies while learning mathematical ideas?
What about the mathematics teachers? Are they prepared to guide student learning of mathematics for the 2010’s and beyond? The National Council of Teachers of Mathematics (NCTM) established its Technology Principle in its Principles and Standards of School Mathematics, “The existence, versatility, and power of technology make it possible and necessary to reexamine what mathematics students should learn as well as how they can best learn it” (NCTM, 2000, p. 25).
Many digital technologies have proved useful for students learning mathematics: graphing calculators, applets of virtual manipulatives (such as those available through the National Library of Virtual Manipulatives at http://nlvm.usu.edu/en/nav/vLibrary.html), spreadsheets, computer algebra systems, and dynamic geometry tools (such as Geometer’s Sketchpad). Each of these technologies provides visual representations that enable students to explore mathematical ideas in more dynamic ways.
When asked to identify technologies for teaching mathematics, one mathematics teacher education professor proposed a technology that surprised many of the participants at his workshop: “iPods – because students have them, and we as teachers need to think of how this technology can be useful in teaching mathematics” (Garofalo, 2006). Many of today’s school-aged youth actively contribute to YouTube, where they upload, view, and share their video clips as a way of communicating their thinking and ideas. They use simple digital video tools such as cell phones, digital cameras with movie settings, and inexpensive camcorders to create and share video and to communicate with others far beyond their local communities. Garofalo’s challenge is, thus, for mathematics teachers to determine how these commonplace video tools can be used for learning mathematics.
Ten years into the 21st century, few teacher preparation programs have been revised so that mathematics teachers are prepared to incorporate digital tools as they facilitate students’ mathematics learning. Perhaps the vision of using digital videos for learning mathematics needs some direction. Our goal in this editorial is to provide that direction to mathematics teachers and teacher educators for using digital videos as mathematical learning tools.
With the capabilities of digital videos, students should no longer be expected to learn mathematical concepts and processes only by sitting and listening to long explanations. They can be more actively involved in constructing their knowledge with the aid of digital video as they explore the amazing world of mathematics. Mathematics teachers must consider how they can adapt their teaching and incorporate these technologies to help students learn mathematics in ways similar to ways students learn and communicate outside the classroom.
The perspective we present goes beyond the traditional view of students passively watching video. We suggest ways digital videos can be used to present challenging mathematical questions for students. Watching, analyzing, and creating are three types of active student engagement with videos we consider (Niess & Walker, in press). Many videos can also be layered with other dynamic, interactive media to engage students in thinking about mathematics in ways previously difficult to achieve.
Watching. Visualization is an important tool in problem solving, and students need multiple visualization opportunities if this tool is to be developed (NCTM, 2000). Through watching appropriate digital videos, students can expand their visualization skills. Watching is a strategy that requires gathering and integrating information from multiple sources, both audio and visual.
Video clips offer instructional possibilities for engaging students in the watching mode to explore mathematical ideas. Video clips (short excerpts from movies, television shows, professionally prepared educational videos, or personally created videos) can be used to introduce new mathematical concepts and processes, explore mathematics in nature, art, or other contexts in the real world, and engage students in expressing their mathematical understandings as they think about what has been said or displayed.
YouTube.com is a rich source for video clips or short movies that can be used for challenging students to watch carefully and engage in mathematical thinking. Consider, for example, Abbott and Costello’s 1941 movie, In the Navy (Video 1). This video has a scene where Lou Costello is explaining to Bud Abbott that he has 28 donuts to share among seven people. Lou decides that each person should get 13 donuts to share the donuts fairly and equally. Bud questions Lou’s mathematics, and the scene progresses until Bud gives up trying to convince Lou that his arithmetic is wrong.
Teachers can challenge students to explore Costello’s division method. Does this method work for all division problems? Students can work together in groups to develop explanations they might use to help Costello understand that his ways of doing the operations are incorrect. One group can consider Costello’s method of dividing and why it is incorrect. Another group can explain why the multiplication proof is incorrect, and another can explain why the repeated addition is incorrect.
By watching videos, students can engage in richer discussions that help them develop a more conceptual understanding of mathematics. Videos such as these provide a starting point for engaging students in mathematical thinking.
Analyzing. Reasoning is a fundamental part of mathematics, and reasoning mathematically is a habit of mind that must be developed through consistent use and in a variety of contexts (NCTM, 2000, p. 56). Analysis is a reasoning process described as a process “to divide a complex whole into its parts or elements” (Analyze, n.d.).
Analysis is at the heart of mathematical reasoning. In mathematics, people analyze situations and models to define functions that describe relationships. Students need experiences that guide them as they learn to reason mathematically. They need opportunities to challenge them to make conjectures based on their analyses and to communicate the thinking that directs them toward their conjectures. Engaging students in analysis of events in video clips is one way of providing these important experiences.
In grades 9-12, students can develop insights into mathematical abstraction and structure by exploring the behavior of nonlinear relationships for polynomial, exponential, rational, and periodic functions. Consider the following scenario:
Abby is riding her bike along the sidewalk and hears a clicking noise as the back wheel turns. Upon investigation she discovers that her tire has a rock lodged in it that is hitting the ground each time the tire rotates. What would the path of the rock look like if it were graphed on the x-y axis?
As students analyze the path of the rock embedded in a bicycle wheel, they are engaged in mathematical processes for identifying a specific functional notation for the motion. They develop tabular and graphical representations of a particular situation to aid in visualizing the mathematical situation.
An animated cycloid video from Jim Wilson, University of Georgia, simulates the path of a rock embedded in a tire (http://jwilson.coe.uga.edu/EMT668/EMT668.Student.Folders/BrombacherAarnout/EMT669/cycloids/cycloid.mov). Figure 1 describes a cycloid of the path of the rock as a function of time. Similar projects can be considered with other objects in motion, such as a seesaw, a Ferris wheel, or other kind of carnival ride. Students might even simulate the motion of these different objects using Geometer’s Sketchpad.
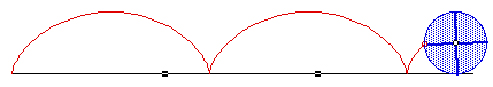
Figure 1. A graphical representation of the path of the rock on the tire as a function of time—a cycloid function.
Analyzing videos adds an often-overlooked dimension to the mathematics. Students need time and appropriate tools to reason through a problem situation, along with guidance from the teacher. Students can learn to analyze information and use the process in future problem solving.
Creating. Communication is an essential aspect of mathematics. According to NCTM’s Principles and Standards for School Mathematics, communication is a way of sharing ideas and clarifying understanding. Creating videos provides a different and perhaps more engaging experience for helping students communicate their mathematical understandings. Students can create a video of someone walking in front of a camera to describe the movement displayed from a particular function. For example, a student can move in front of the camera in the shape of the function y = abs(x). The student would start about 3 meters away from the camera, walk toward the camera at a constant rate, and then walk away from the camera at the same constant rate.
Alternatively, one student can be recorded moving in front of the camera, and other students can be challenged to determine the function for the path of that person. They can explore their solution either by graphing the movement or by defining it as a function, which they demonstrate by entering the data in their graphing calculators or spreadsheets and displaying both the tabular and graphical representations. They can include these visuals in their video creations to display the multiple representations of the person’s movement.
The valuable part about creating videos is that students also engage in watching and analyzing the videos. Thus, all three modes are used to develop an understanding of mathematics. Students can create several types of videos to enhance learning in the mathematics classroom. They might use digital video equipment to create a teaching video, in which they teach a mathematical concept or process. Small groups of students can create a video that shows each student completing problems as they explain what they learned in class that day. Students in each group can pick one problem from the homework set and show how to complete that problem in a video.
Another idea for working with students to create mathematics using digital video technology comes from choreography. Dance, gymnastics, skating, baton twirling, and cheerleading routines have a designed set of movements. One of the most complicated forms of choreography is that used by a band director for a marching band and its formations. A marching band not only performs musical compositions, but also entertains with steps and movements to create designs such as letters, logos, or even animated pictures. The members must mark time, change directions, change style of stepping, march forwards and backwards, all while playing the designated music with their instruments. Band directors must create a set of movements and formations for their members that coincide with the music, yet also look visually appealing to an audience watching both from the ground level and from above.
Creating a digital video of a choreographed piece that focuses on the mathematics of the movements, as with band formations, requires far more than simply designing the movements and gathering appropriate videos. Students need to be guided in thinking about the mathematics of the various formations. They need to watch videos of different formations where they are asked to identify potential mathematical functions for the formation being displayed in the video clips.
Students might even watch the videos at different speeds to examine more carefully the movement. They need experiences in mathematically analyzing the actions, where they must defend their analyses for the mathematics involved in the formation. These experiences are essential in their preparation for engaging in creating formations that evolved from various mathematical functions. They might even make another video in which they describe their mathematical reasoning used in developing a dynamic choreographed video solution.
When adding digital videos to the pedagogical mix, teachers are able to challenge students to reflect on what is said and done in ways that ultimately engage them in important mathematical processes—reasoning and problem solving. Incorporating digital videos in classroom instruction provides students with a different and often more engaging way for communicating what they know and understand. Communicating about mathematics is an essential ingredient for clarifying their reasoning and understanding. Creating videos provides ways to meet this goal. Integrating digital videos with additional dynamic media such as Geometer’s Sketchpad, spreadsheets, calculators, and virtual manipulatives affords students with opportunities to create, use, and make sense of multiple representations of mathematical ideas.
Using videos in teaching mathematics is certainly not consistent with traditional views of teaching mathematics. Typically, teachers have not experienced learning mathematics through all of these three modes—watching, analyzing, and creating—using videos. They, like their students, have “watched” teachers as they did the analysis. For sure, their teacher preparation programs have not guided them in thinking about teaching with videos as considered in these three modes.
The challenge is for mathematics teacher educators to restructure the preparation programs to consider preparing teachers to engage in this new vision—a vision that incorporates digital videos in ways that provide exciting, effective, and rigorous mathematics learning opportunities for K-12 students. This challenge calls for the development of a teacher knowledge that includes technology, pedagogy, and content knowledge, or TPACK (Mishra & Koehler, 2006; Niess, 2005). This dynamic framework invites teacher educators to redesign their programs toward developing the knowledge needed for responding to the challenges of integrating technologies such as videos as tools for learning mathematics.
Teacher educators must prepare teachers for guiding students in exploring mathematical concepts and processes in visual ways and use digital video as a tool for communicating their thinking. Teacher educators need to challenge preservice and in-service teachers’ beliefs and dispositions about what mathematics is important to learn and how that mathematics needs to be learned, specifically when thinking about digital technologies such as digital videos. Preservice and in-service teachers need opportunities to explore different ways of learning and developing mathematical ideas with digital videos. They need to be challenged to reconsider the mathematics curriculum with respect to what is important to know and be able to do in mathematics. They need to be engaged in using digital videos in exploring mathematics by watching, analyzing, and creating.
Ultimately, teacher educators need to redesign their programs to provide opportunities that help preservice and in-service teachers in envisioning and implementing videos as instructional items in light of the impact of the capabilities of this technology on mathematics as a discipline as well as a societal tool.
How will the teen years of the 21st century impact the teens in our classrooms learning mathematics? Teacher educators must seriously reconsider how they prepare teachers to teach mathematics. They need to incorporate ways that more accurately represent mathematics, how it is developed, and how it is implemented in society. They need to provide preservice and in-service teachers with experiences that engage them in thinking about the mathematics and how it can best be learned using more visual technologies in concert with other digital technologies.
References
Analyze. (n.d.). In Merriam-Webster online dictionary. Retrieved from http://www.merriam-webster.com/dictionary/analyze
Garofalo, J. (2006, January). Which technologies to incorporate into teacher preparation, including goals, rationales and relative emphases? Workshop presented at the annual meeting of the Association of Mathematics Teacher Educators, Tampa, FL.
Mishra, P., & Koehler, M.J. (2006). Technological pedagogical content knowledge: A framework for integrating technology in teacher knowledge. Teachers College Record, 108(6), 1017-1054.
National Council of Teachers of Mathematics. (2000). Principles and standards for school mathematics. Reston, VA: Author.
Niess, M. L. (2005). Preparing teachers to teach science and mathematics with technology: Developing a technology pedagogical content knowledge. Teaching and Teacher Education, 21(5), 509-523.
Niess, M. L. , & Walker, J. M. (in press). Digital video in mathematics education. In G. Bull & L. Bell (Eds.), Teaching with digital video: Watch, analyze, create. Eugene, OR: International Society for Technology in Education.
![]()