As equity in mathematics education has garnered more attention, multiple avenues have emerged for increasing learning opportunities for students historically marginalized in mathematics. In this project, we brought together two avenues for equity-minded mathematics teaching: developing mathematics teaching among prospective teachers (PTs) that incorporated both technology and funds of knowledge to foster mathematics learning toward supporting broader equity goals.
This study examined mathematics teaching that utilized digital technologies for increasing opportunities for mathematical reasoning and sense making and supporting positive dispositions toward mathematics (as recommended in Forgasz et al., 2010). Leveraging funds of knowledge means using the cultural, linguistic, and cognitive resources from home or community settings to promote learning the school mathematics curriculum (Aguirre et al., 2012; Harper et al., 2018).
This paper reports our study of the use of robotics in mathematics teaching, which are appropriate for working with students across grades preK-12 and for supporting culturally responsive teaching (Leonard et al., 2016, 2018; Newton et al., 2020; Sullivan & Bers, 2016; Xia & Zhong, 2008). We explored PTs’ development of mathematics teaching within an elementary mathematics methods course through a model that engaged them in learning about technological tools and about community-based mathematics practices. Our aim was to design and implement science, technology, engineering, and mathematics (STEM) activities that leveraged both robotics and funds of knowledge.
Background
Although this study centered PTs’ development, we grounded our work with PTs in separate lines of research. This research suggested that integrating digital technology and leveraging funds of knowledge in mathematics classrooms can positively impact the mathematics achievement of preK-12 students (e.g., Kisker et al., 2012; Li & Ma, 2010). Bringing these two pedagogical approaches together in a university-based elementary mathematics methods course was grounded in theories framing both mathematics and digital technologies as situated within cultural practices. This effective and equitable teaching had to address the different needs, positions, and identities of students as they engaged with mathematics through technology (Forgasz et al., 2010; Nasir, 2002).
Teaching mathematics with technology shows potential to support differentiated instruction and student-centered practices (Thomas & Edson, 2019), but without cultural considerations can exacerbate inequities (Forgasz et al., 2010). In particular, differences across gender, race/ethnicity, and socioeconomic groups in access to and uses of technology at both home and school may further limit mathematics learning opportunities for students from historically marginalized groups (Forgasz et al., 2010; Warschauer & Matuchniak, 2010; Wang & Moghadam, 2017).
For example, Black students are likely to use technology in school mathematics at least once a week; however, the use of that technology often prioritizes remedial computer-drill (Kitchen & Berk, 2016; Warschauer & Matuchniak, 2010), an instructional approach that is known to perpetuate inequitable mathematics outcomes for Black students (Berry et al., 2014; Martin, 2019). Thus, educators integrating technology into mathematics teaching toward equity goals must consider the broader sociocultural and sociopolitical conditions impacting mathematics learning opportunities for students from historically marginalized groups.
Accordingly, a funds of knowledge approach is a promising complement to teaching mathematics with technology because of the inherent emphasis on bridging cultural-, community-, and home-based practices with school practices. Leveraging funds of knowledge fosters a strengths-based approach by positioning students’ diverse knowledge bases, experiences, and resources as assets for mathematics learning (Aguirre et al., 2012; Moll et al., 1992). Adopting such a strength-based approach encourages teachers to emphasize what students know and can do with available resources and tools, which may mediate challenges in the differential access and use of technology.
This study aimed to integrate two considerations essential in the preparation of teachers of mathematics (Association of Mathematics Teacher Educators, 2017), using mathematical tools and technology and drawing on students’ mathematical strengths, usually considered separately. In this study, we asked the following research question: How do PTs develop mathematics teaching that uses the cultural, linguistic, and cognitive resources from home and community settings to promote learning school mathematics with robotics?
Learning to teach mathematics with technology requires a sophisticated and integrated knowledge of teaching, mathematics, and technology (Koehler & Mishra, 2009; Thomas & Edson, 2019). Likewise, learning to bridge funds of knowledge and the school mathematics curriculum requires deep knowledge of teaching, mathematics, and students’ community- and home-based experiences and resources (Aguirre et al., 2012; Harper et al., 2018). Thus, bringing together these two avenues for equity in mathematics education increases the complexity of learning to teach.
Our study aimed to open pathways for and identify challenges to preparing PTs for teaching mathematics by leveraging both technology, namely robotics, and funds of knowledge. The following sections contain a brief overview of the research on teaching and learning to teach mathematics with robotics and funds of knowledge.
Research on Robotics in STEM Education
Policymakers have called for integrated content frameworks to support preK-12 STEM education that incorporates critical thinking, fundamentals of coding, and use of digital technologies (e.g., Tennessee Department of Education, 2018a). Students historically marginalized in mathematics, however, also experience marginalization when learning coding and using digital technologies.
For example, by age six, stereotypes that boys are better than girls at robotics and computer programming lowers girls’ sense of belonging in STEM and limits their access to activities such as computer games and technological toys (Master et al., 2016). Research consistently shows that cultural stereotypes and, consequently, limited opportunities to engage with coding and digital technologies maintain gender inequities (Bian et al., 2017; Funke et al., 2017; Master et al., 2016, 2017), but considerations of access and participation among Black and Latinx children remain underexplored. In fact, the experiences of Black and Latinx children have been largely ignored (Newton et al., 2020).
Only a few studies have taken up cultural considerations (Leonard et al., 2016, 2018; Newton et al., 2020; Scott et al., 2015), but those studies show that robotics offers an authentic way for teachers and students to draw on cultural capital as they use digital technologies and engage with the fundamentals of coding. Further, robotics provides a highly engaging STEM strategy to reinforce mathematical concepts (e.g., solving equations; Grubbs, 2013).
In addition to individual motivation, Yuen et al. (2014) found that using robotics facilitated collaborative learning experiences that encouraged students to draw on multiple strengths in design and implementation, which aligns with equitable approaches to broadening participation in mathematics education (Esmonde, 2009). Additional research suggests that student use of robotics is an impactful instructional method for students with exceptional needs (e.g., autism spectrum disorder, emotional and behavioral disorders, Down syndrome, and medically fragile students; Benitti, 2012; Knight et al., 2019; Nickels & Cullen, 2017; Taylor, Vasquez et al., 2017).
Support for developing teachers’ STEM instructional skills and pedagogical use of robotics is an ongoing area of research. Leonard et al. (2018) focused on developing practicing teachers’ STEM skills in tandem with culturally responsive teaching. Findings from their study noted increased teacher efficacy, improved technical understanding, and development of equitable STEM practices for teachers.
Research indicates a continued “need for teacher [professional development] and ongoing support as teachers integrate robotics and computational thinking in their classrooms” (Chalmers, 2018, p. 99). This study contributes to the field’s emerging understandings of how teachers learn to integrate robotics into mathematics instruction.
Research on Funds of Knowledge in Mathematics Education
Guidance on preparing teachers of mathematics increasingly has emphasized the importance of drawing on students’ mathematical strengths, particularly regarding valuing diverse mathematical, cultural, and linguistic funds of knowledge (Association of Mathematics Teacher Educators, 2017). Funds of knowledge refer to the “historically accumulated and culturally developed bodies of knowledge and skills essential for household or individual functioning and well-being” (Moll et al., 1992, p. 133). Accordingly, mathematics instruction that leverages funds of knowledge uses the cultural, linguistic, and cognitive resources from home or community settings to promote learning the school mathematics curriculum (Aguirre et al., 2012; Harper et al., 2018).
Research has shown that culturally relevant learning environments that value funds of knowledge positively affect student effort and engagement (Howard, 2001; Ladson-Billings, 2009). In addition to increasing participation, incorporating children’s everyday mathematics practices into classroom instruction challenges students’ expectations about mathematics, broadening ideas about who can do mathematics and what mathematics is (Civil, 2002). Consequently, situating mathematics in its community- and home-based cultural context significantly increases student performance on traditional measures of mathematics achievement (Kisker et al., 2012).
Supporting teachers to utilize funds of knowledge for students’ learning of school mathematics is not straightforward (Civil, 2007). Given the commonly held misperception that mathematics is culturally neutral, the divide between home-based and school-based mathematics remains wide. Thus, identifying and leveraging everyday mathematics practices proves more challenging than in other disciplines such as language arts or social studies (González et al., 2001).
Research on PTs’ development of mathematics teaching that leverages funds of knowledge shows a tendency toward only superficial connections to out-of-school experiences (e.g., changing names of locations) or only procedural mathematics (e.g., calculations with money) rather than reasoning and sense making (Aguirre et al., 2012; Harper et al., 2018). These findings indicate a continued need for research in mathematics teacher education on bridging funds of knowledge and school mathematics in meaningful ways, and this study contributes to the field’s understandings of how teachers learn to leverage funds of knowledge in mathematics instruction.
Transdisciplinary Connections as Funds of Knowledge
In addition to home- and community-based funds of knowledge, we also included knowledge, experiences, and ways of knowing from other disciplines in our current framing of funds of knowledge. We chose to broaden the concept of funds of knowledge in this study for several reasons.
First, connecting mathematics to other disciplines shows promise for addressing inequities in mathematics (Jao & Radakovic, 2018). Transdisciplinary connections can enhance students’ ability to leverage their home- and community-based funds of knowledge in meaningful ways in mathematics (Harper 2017, 2019). Further, the focus on using robotics in mathematics is inherently transdisciplinary, bringing together computer science and mathematics. A conceptual model that expands STEM to STEAM, by promoting cross-curricular content integration through art-related fields (e.g., social studies, literature, and visual art), fosters teachers’ ability in creating authentic problem-based learning tasks (Quigley et al., 2017).
Finally, teachers can identify and leverage everyday practices from children’s lives more easily in other disciplines than in mathematics (González et al., 2001). Thus, we hoped that encouraging transdisciplinary connections might also enhance teachers’ ability to leverage children’s home- and community-based funds of knowledge in meaningful ways in mathematics instruction.
Research Questions
Overall, we aimed to explore how PTs develop mathematics teaching that uses the cultural, linguistic, and cognitive resources from home and community settings to promote learning school mathematics with robotics. More specifically, we addressed the following research questions:
- How do PTs connect mathematics learning and robotics?
- How do PTs who use robotics for mathematics instruction leverage community funds of knowledge and transdisciplinary connections?
Methods
Context and Participants
Research took place within initial teacher licensure programs at a public university in the southeastern United States. Across fall 2018, spring 2019, and fall 2019, groups of PTs from five sections of a master’s-level elementary mathematics methods course designed, planned for, and facilitated mathematics activities at informal STEM events (henceforth, family STEM nights) hosted afterschool by nearby public elementary schools and preschools. Table 1 provides a summary of PT enrollment across the five sections of elementary mathematics methods.
Table 1 Prospective Teacher Enrollment Across Five Sections of Mathematics Methods
| Semester | Section Focus | Total PTs | Enrollment by Licensure Area/Cohort |
|---|---|---|---|
| Fall 2018 | K-5 | 16 | 8 master’s candidates seeking initial licensure for K-5 6 master’s candidates seeking initial licensure for special ed 2 undergraduate (seniors) seeking initial licensure in deaf ed |
| Fall 2018 | PK-3 | 20 | 19 master’s candidates seeking initial licensure for PK-3 1 undergraduate (senior) seeking initial licensure for K-5 |
| Spring 2019 | K-5 | 21 | 8 master’s candidates seeking initial licensure for K-5 (urban) 9 master’s candidates seeking initial licensure for K-5 4 master’s candidates seeking initial licensure for special ed |
| Fall 2019 | K-5 | 22 | 11 master’s candidates seeking initial licensure for K-5 9 master’s candidates seeking initial licensure for special ed 2 undergraduate (seniors) seeking initial licensure in deaf ed |
| Fall 2019 | PK-3 | 24 | 24 master’s candidates seeking initial licensure for PK-3 |
| TOTAL | 103 | 37 seeking initial licensure for K-5 (28 master’s candidates; 1 undergraduate; 8 master’s – urban) 43 master’s candidates seeking initial licensure for PK-3 19 master’s candidates seeking initial licensure for special ed 4 undergraduate (seniors) seeking initial licensure in deaf ed |
We did not explicitly ask PTs to self-identify race and gender; therefore, these demographics are not reported in Table 1. Based on informal conversations with students across the courses, demographics seemed typical of teacher education programs nationally (i.e., mostly young white women from middle class backgrounds).
Harper was the instructor for all sections of the course, and Stumbo served as a teaching assistant for all sections in spring and fall 2019. The methods course is designed for master’s candidates seeking initial licensure while simultaneously completing a yearlong teaching internship; however, some undergraduate students take the course before their internship based on senior privilege. The course is a requirement for PTs across multiple licensure programs, including preK-3 licensure, K-5 licensure, K-5 licensure with a focus on urban, multicultural education, special education, and deaf education.
In preparation for facilitating activities at the family STEM nights, all PTs completed various activities and readings to learn about leveraging cultural and community funds of knowledge, making transdisciplinary connections, and using digital technology in mathematics teaching as part of the methods course (for more details about course readings, class and homework activities, etc., across various sections and semesters, see Harper, 2020).
For example, in fall 2018, several groups of PTs expressed interest in using available robotics but ultimately decided to use nondigital resources (e.g., snap cubes and geometric shape building sets) because they lacked experience using robotics. Consequently, beginning in spring 2019, we included in the methods course one 3-hour class session dedicated to using technology, transdisciplinary connections, and home- and community-based experiences in mathematics education.
Building on required course readings (e.g., Carpenter et al., 2017; Daml, 2017), this lesson introduced general principles for teaching mathematics with technology and making transdisciplinary and home-school connections. PTs engaged with three robotics tools and one nondigital STEM tool to identify ways of supporting mathematics and transdisciplinary learning using available resources and to identify possible home-school experiences to enrich this learning.
The nondigital STEM tool focused on magnetism (STEM Magnets Set by Learning Resources; https://www.learningresources.com/stem-magnets-activity-set). One robotics tool involved programming a pathway on a tablet using block coding (Dash by Wonder Workshop; https://www.makewonder.com/robots/dash/). Another robotics tool involved programming a pathway using directional arrows on the robot itself (Code and Go Robot Mouse by Learning Resources; https://www.learningresources.com/code-gor-robot-mouse-activity-set). Another involved connecting circuits to build a robot that could create artwork with one or two markers (Smart Art Circuit Cubes by Tenka; https://circuitcubes.com/collections/kits/products/circuit-cubes-smart-art-kit-lite).
The family STEM night project served as the major course project and was designed to integrate various ideas from across course activities and readings (not only the 3-hour lesson described previously). The project design was adapted from the Community Exploration Module developed by the TEACH Math project (Turner et al., 2015). The project was divided into four stages in fall 2018 and spring 2019 and five stages in fall 2019. PTs completed the project in small groups of two to four. For more details about the major project, including detailed assignment descriptions and rubrics, see Harper (2020). Stages were as follows:
1. Community Walk. PTs visited the community surrounding the school where the family STEM night was held to identify community-based experiences and mathematics and STEM practices they could leverage in their activity design. They reflected on what they learned about mathematics in the community and proposed an idea for the family STEM night activity. This stage supported PTs to (a) describe key mathematics concepts; (b) use tools, technology, and other resources effectively to support mathematics learning; (c) leverage community-based experiences to support mathematics learning; and (d) view all people as mathematically capable.
2. Lesson plan. PTs developed a lesson plan for their family STEM night activity and received feedback from the instructor or teaching assistants. Revisions were made as needed. This stage was designed to address the four goals from Stage and 1 was also to support PTs to anticipate children’s strategies and mathematical thinking and view students as capable of solving sophisticated, yet accessible, mathematics problems.
3. Lesson implementation. PTs implemented their lesson at a family STEM night.
4. Reflection. PTs answered eight reflection questions to demonstrate their ability to recognize children’s mathematical engagement and to describe what they learned about mathematics teaching and from the lesson implementation. This activity focused on the Stage 1 and 2 goals and encouraged PTs to relate children’s strategies to the mathematics concepts students are learning.
5. Revision and publication. Beginning in fall 2019, PTs revised their lesson plan based on their experience with implementation and their reflection. They provided a rationale for their revisions and published their final lessons to share with other teachers. This activity was added to encourage PTs to analyze and learn from their own teaching and contribute to a network of teachers who seek to improve their classroom practice.
Data Sources and Analysis
Data sources included reflections on the community walk and initial ideas for STEM lessons (one per group of PTs; Project Stage 1), initial and final lesson plans with instructor feedback (one per group of PTs; Project Stages 1 and 5), and written reflections following the implementation of the STEM lesson (one per PT; Project Stage 4). Analysis proceeded through various rounds, which were guided by a qualitative approach that involves iterative rounds of analysis to describe, condense, and display the data in ways that allow for researchers to identify themes (Miles et al., 2014) in relation to the research question.
Round 1
The first round of analysis sought to condense the data set by limiting it to those data relevant to the research questions (i.e., only those lessons that used robotics in mathematics instruction). Harper examined each lesson plan from all five sections of the course to identify whether planned activities incorporated robotics or other digital technologies. Only those groups who incorporated robotics were selected for the second round of analysis. Accordingly, only data from two groups in spring 2019 and two groups from fall 2019 were included in subsequent analyses.
Ten PTs participated as members of these four groups; all 10 PTs were master’s degree candidates completing their yearlong internship. In spring 2019, both groups – a group of four special education PTs (Adam, Claire, Peyton, and Whitney; all names are pseudonyms) and a pair of K-5 urban education PTs (Hollie and Idil) – implemented their lessons at Dozier Elementary (an urban Title I, preK-5 school; 42% Black, 17% Latinx student population). In fall 2019, a pair of K-5 PTs (Brittany and Lily) implemented their lesson at Mountainside Elementary (a rural Title I, K-5 school; 90% white student population). The other pair in fall 2019 (Dallas and Hope) implemented their lesson at Moses Smith Preschool (an urban Title I, public preschool; 90% Black student population).
Round 2
The goal of the next round of analysis was to develop a codebook to describe the contents of the data set relevant to the research questions. Harper began this process by analyzing written reflections by PTs in selected groups from spring and fall 2019. Using NVivo, analysis began with three broad themes: (a) mathematics, (b) technology, and (c) funds of knowledge. Through iterative cycles of open coding, subthemes were identified and data were coded at the sentence level. Additional themes were added until codes were exhaustive. Initial analysis focused on individual responses to three of eight reflection questions.
- How did you see children and families engaging in mathematics during your task?
- How did you see children and families connect your mathematics task to or align it with family or community knowledge or practices?
- How did you see children and families connect your mathematics task to other content areas (e.g., STEAM or literacy)?
These three questions were selected for codebook development because they elicited PTs’ ability to recognize and describe children’s engagement with mathematics, technology, and funds of knowledge, whereas other reflection questions asked about mathematics teaching and learning more broadly.
The appendix provides definitions for all themes and subthemes, with illustrative examples for each subtheme. Subthemes for mathematics focused on identifying the specific mathematics topics engaged during the STEM night (e.g., counting, distance, or multiplication).
Subthemes for funds of knowledge identified sources of home or community connections (e.g., familiar locations, family, or parental involvement). Subthemes for technology noted the specific robotics and supporting digital technologies used or how the tools were used (e.g., Code and Go Robot Mouse or Dash Robot).
One additional theme emerged, Access, which denoted PTs’ descriptions of how mathematics was accessible for or inclusive of every student. Subthemes in this category identified specific features of an activity that broadened access to mathematics (e.g., multiple entry points or student input). Stumbo and Kim reviewed the initial coded excerpts using the codebook to confirm that themes and subthemes were exhaustive for responses to the three reflection questions and helped refine definitions in the final version of the codebook.
Round 3
Harper used the established codebook (appendix) to analyze remaining data. Namely, the codebook was used to analyze data from the community walk (Project Stage 1), group lesson plans (Project Stages 2 and 5), and individual written responses to the remaining five reflection questions (Project Stage 4):
- What were your strengths at supporting students to grapple with a challenging mathematics task?
- What is an area of growth for you to support students to grapple with a challenging mathematics task?
- How did this experience help you see what diverse learners are capable of doing in mathematics (versus what they can’t do)?
- Based on your experience, what opportunities and challenges do you see for using these types of tasks in your future mathematics teaching?
- Anything else that you’d like to share about what you learned about mathematics teaching and/or learning? (Optional)
Harper applied codes at the topic level (i.e., coding phrases/sentences or groups of sentences related to the same topic), and only excerpts relevant to mathematics, funds of knowledge, and technology were coded. Access codes were applied only to excerpts that were otherwise coded. Finally, hierarchy charts and matrices were created using NVivo to identify the prevalence of subthemes through a visual data display showing nested relationships among codes and data sources.
We also created data displays in NVivo to show cooccurrence of codes to examine connections among mathematics, robotics (RQ1) and community funds of knowledge (RQ2). Excerpts coded for prevalent subthemes and for cooccurring themes were reviewed to generate the overarching findings and to identify illustrative excerpts.
Round 4
To address questions about the relationship between mathematics instruction using robotics and transdisciplinary connections (RQ2), Stumbo identified instances in the lesson plans that either explicitly named standards from disciplines outside of mathematics or suggested cross-curricular connections, using his familiarity with the content standards as a former elementary teacher and current elementary teacher educator. Excerpts were taken verbatim and analyzed using the state content standards for fine arts, science, computer science, and social studies. Five content areas emerged as relevant in the analysis. Within each content area, Stumbo identified the relevant strands within the state standards, as follows.
Computer Science. The relevant strand was coding and computer programming. Within this strand students “use analytical and innovative problem solving skills to decompose, identify patterns, generalize information, and formulate algorithmic processes to solve a problem or related set of problems with a variety of tools” (Tennessee Department of Education, 2018b, p. 5).
English Language Arts. The relevant strand was speaking and listening. Within this strand students “present information/ideas formally and informally in such a way that others can follow a line of reasoning” (Tennessee Department of Education, 2016a, p. 41).
Fine Arts. The relevant strand was the visual arts strand. This strand describes how “the course of instruction in all public schools for (K-8) shall include art … education to help each student foster creative thinking, spatial learning, discipline, craftsmanship and the intrinsic rewards of hard work” (Tennessee Department of Education, 2018c, p. 1).
Science. Two relevant strands were related to science: engineering design and physical science. Within the engineering strand students “integrate science, mathematics, technology, and engineering design to solve problems and guide everyday decisions” (Tennessee Department of Education, 2016b, p. 1). The physical science strands suggested, “Children form mental models of what science is at a young age” (Tennessee Department of Education, 2016b, p. 8).
Social Studies. Two strands were relevant: geography and culture. Within the geography strand, “students will use knowledge of geographic locations, patterns, and processes to show the interrelationship between the physical environment and human activity…” (Tennessee Department of Education, 2017, p. 10). Within the culture strand, “students will use culture and cultural diversity to understand how human beings create, learn, share, and adapt to culture and appreciate the role of culture in shaping their lives and society, as well the lives and societies of others” (Tennessee Department of Education, 2017, p. 10).
Finally, charts for the cooccurrence of codes were created in NVivo to show connections between mathematics instruction using robotics and transdisciplinary content (RQ2). Excerpts coded for prevalent and cooccurring themes were reviewed to generate the overarching findings and to identify illustrative excerpts.
Findings
Of six total groups, only two groups incorporated robotics into their STEM night activities in spring 2019, and of 20 total groups, only two groups incorporated robotics into their activities in fall 2019. Findings come from the lesson plans and individual reflections of PTs in those four groups.
In this section, first is an overview of the mathematics, funds of knowledge, and robotics planned for each activity based on the analysis of lesson plans. Then is an elaboration of what individual reflections and lesson plans suggested about PTs’ development of mathematics teaching that uses funds of knowledge to promote learning school mathematics with robotics toward broader equity goals.
Overview of Dash Robot Activities
In spring 2019, Adam, Claire, Peyton, and Whitney, planned for students at Dozier Elementary to navigate the Dash Robot to move between familiar community-based locations on a grid with 20-centimeter blocks (Figure 1). In the lesson plan, the group focused on mathematical ideas of sequencing as students gave “directions through up, down, left, or right to move the Dash Robot from a starting point to an ending point.” They planned that students would need to engage in mathematical practices such as making sense of problems and persevering to solve them as they calculated “distance between places by both measuring through 10 cm [or 20 cm] increments and/or blocks.”
Figure 1 Adam, Claire, Peyton and Whitney’s Lesson in Action

The group described technology integration in their lesson through the use of the Dash Robot and also noted that “once the distance is calculated the students will input the information to the iPad touchscreen tablet PC to control the Dash Robot.” Finally, in describing community funds of knowledge that students could leverage, the group focused on how the activity would be “engaging and accessible for students and families in the area because it allows them to make real world connections. The places on the map will provide a similar approximation to the actual locations of the places in the community.” This approximation of relative distances and locations was designed to allow students to leverage their knowledge of the community to navigate Dash on the grid.
In fall 2019, Brittany and Lily planned for students at Mountainside Elementary to navigate the Dash Robot to move from the local Corner Market to four regional distributors to collect ice, jam, milk, and peaches and to deliver the supplies to the Corner Market. In the lesson plan, the pair focused on the mathematics involved in counting by ones or tens. Both the grid and the iPad application for controlling the Dash Robot’smovement were based on 10-centimeter increments, allowing for one-to-one correspondence.
Brittany and Lily also emphasized the need for engaging in mathematical practices related to reasoning and problem solving because some of the items from distributors are perishable. Students needed to “find one efficient path for the Wonder Workshop Dash to take in order to pick up all the required items.”
The pair described technology integration in their lesson through the use of the Dash Robotand its accompanying application:
Students are required to utilize an application on a tablet to practice basic coding skills in order to make Dash move from location to location. They will be tasked with making Dash turn different directions and move forward across different distances.
In describing this technology integration, they highlighted computational thinking (i.e., algorithmic thinking, and sequencing) in coding the Dash Robot. Finally, in describing community funds of knowledge that students could leverage, the pair focused on how “Corner Market is unique to the school community. All the locations and foods should be familiar to the students who have been to Corner Market before.” Brittany and Lily had used products and distributors they identified when visiting the market and talking with the market employees.
Figure 2 Brittany and Lily’s Lesson in Action

Overview of Code and Go Robot Mouse Activities
In spring 2019, Hollie and Idil planned for students at Dozier Elementary to navigate the Code and Go Robot Mouse to move from the school or a student’s home to a familiar community location (Figure 3). In the lesson plan, the pair focused on the mathematics involved in “mapping and coding the pathway from Point A to Point B.” In particular, they named, visual/spatial reasoning by predicting movement in space to create sequences that determine “step-by-step, how to go from place to place in the community.”
Figure 3 Hollie and Idil’s Lesson in Action

They also noted a need to engage in mathematical practices related to making sense of problems and persevering in solving them. The pair described technology integration in their lesson through the use of the Code and Go Robot Mouse, which“moves on its own [through a predetermined pathway] once programmed by the participants.”
In describing technology use, they emphasized predicting the sequence to code the mouse to move through the pathway. Finally, in describing community funds of knowledge that students could leverage, the pair focused on how
students and families will use knowledge of places in the community to formulate a path between two…places that they frequently visit. This requires that students think about what they know about the location between two points as well as their experience walking, driving, biking, etc. from one to the other in order to code a pathway.
Hollie and Idil envisioned students connecting to their experiences traveling between two places as a basis for deciding which path the Code and Go Robot Mouse should take.
In fall 2019, Dallas and Hope planned for students at Moses Smith Preschool to identify a favorite location, place a moveable token on a grid to indicate the location, and navigate the Code and Go Robot Mouse to the location (Figure 4). In the lesson plan, the pair identified a range of mathematics concepts, including assigning “a number word to each square on the grid as they count them and then demonstrate cardinality by answering how many squares they counted or plan to move in total” and understanding “a unit is a consistent measurement of distance that the mouse moves.” They also emphasized the need for making sense of spatial reasoning to plan a sequence for the robot mouse’s movement.
Figure 4 Dallas and Hope’s Lesson in Action.
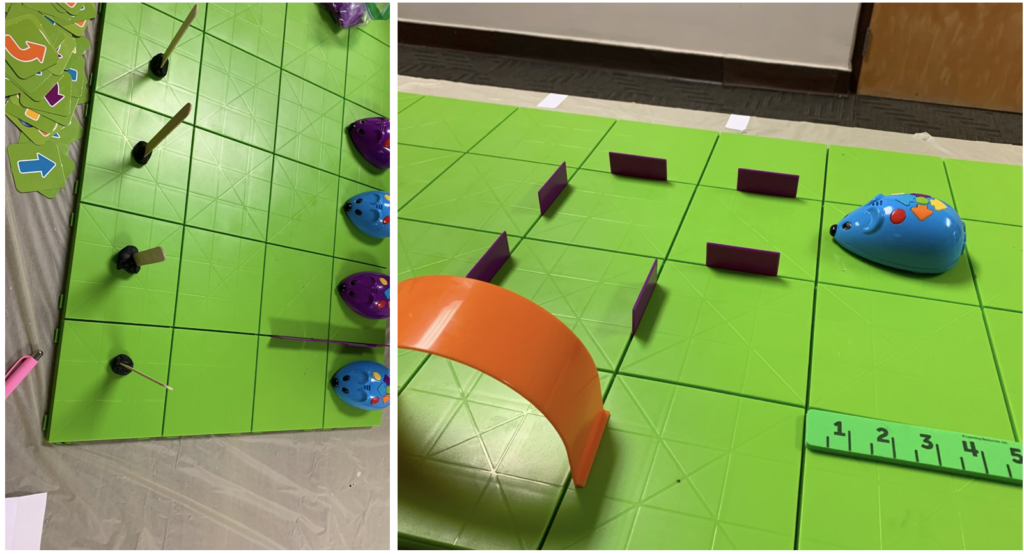
The pair described technology integration in their lesson through the use of the Code and Go Robot Mouse and related the mathematics content to coding: “The use of the programmable mouse engages children in coding. This requires them to translate their understanding of the units and patterns into a dictated code.”
Finally, in describing community funds of knowledge that students could leverage, Dallas and Hope imagined that using “landmarks found in the community are meaningful to the children, which will prompt children to make connections to real experiences.” They noted possibilities for making similar community connections without the Code and Go Robot Mouse: “The task of navigating between community landmarks is able to be replicated without the mouse at home/school through drawing and writing,” but they saw the novelty of a unique material not readily available for students to use in mathematics instruction as a way of promoting engagement.
Mathematics and Robotics
This section begins with a discussion of findings that showed how PTs connected mathematics and robotics (RQ1) following the implementation of their lessons. Both the Robot Mouse and the Dash Robot facilitated engagement with mathematics concepts related to counting, distance, and pattern recognition and sequencing (i.e., computational thinking). The features of each digital technology, however, led to opportunities for engaging with multiplicative reasoning through the Dash Robot activities and for emphasizing trial and error through the Robot Mouse activities.
The Robot Mouse emphasized mathematics concepts related to counting, as Dallas described: “Families and children alike would point to and count the squares, then press the buttons on the [Robot Mouse] for the same number of units, and then also count the movements of the mouse as it traveled.” Both pairs also noted that coding the Robot Mouse (i.e., preprogramming by selecting the appropriate direction buttons on the top of the mouse) engaged students with sequencing and persevering in problem solving.
For example, Idil described how “students had trouble understanding that the mouse turns on the same box instead of the next square,” which facilitated, as Hollie explained, students working to “discover the correct [sequence to navigate the] pathway through trial and error.” Likewise, Hope described a memorable instance when “I knew what [a student] had done wrong, but let him struggle so he could problem solve. This trial and error led him to understand what he was doing wrong and he fixed it on his own.”
Both groups who used the Dash Robot described a similar coding process, requiring sequencing and perseverance, by creating block codes on an iPad application to move Dash. For example, Brittany noted how she engaged students by asking questions such as, “Was there another route you could have taken that was just as efficient as your first route?” or “Why did you choose to get the ice before the milk?”
The application on the iPad tablet, however, required students to input distance beyond counting the number of squares, in addition to direction, for the robot’s movements. PTs described how this technological feature facilitated additional mathematical engagement. Accordingly, Brittany’s questions for students included, “How many boxes until you get to that location?” The features of the digital technology also required her to ask, “What do you notice about the measurements of the paper?”
In Adam, Claire, Peyton, and Whitney’s lesson, Dash needed to move through a map that was built from 20 cm blocks, and in the lesson plan, PTs identified the primary mathematical goal as calculating the distance. In reflections, however, PTs emphasized the multiplicative reasoning necessary to calculate distance as the primary mathematical work of the activity. As Peyton described, “Some students knew their multiplication [facts and could] figure out how far to program the robot to go by counting the blocks and multiplying by 20. Other students … used the multiplication chart or repetitive addition.”
Likewise, Brittany and Lily both emphasized that students easily counted by tens when moving Dash on their 10 cm grid. As Lily noted, “Once students realized that each box was 10 centimeters, they started counting by 10s to determine how far to make the robot move.”
Mathematics With Robotics and Funds of Knowledge
Next is a discussion of findings that showed how PTs leveraged cultural and community funds of knowledge in mathematics instruction that used robotics (RQ2). Groups emphasized different mathematics topics and integrated different digital technologies, but all groups leveraged similar community-based funds of knowledge, namely familiar community-based locations. All PTs agreed with Hollie that students “were able to use their knowledge of the community” to make sense of sequencing to travel between locations in an authentic way, which is important “in order to successfully connect students to the learning.”
PTs across groups also described how familiarity with community locations supported students in reasoning about distance. For example, Peyton described how students relied on familiarity with community locations to place locations on the map that reflected authentic distances as far or near:
When students drew their house or favorite place in their community, I asked the students if their house or favorite place was close to the school or far away from the school. The students then answered with time, blocks, or miles. After answering how far their house or favorite place was from the school, they would translate if that was close or far. This allowed the students to use their knowledge of the community and distance to determine if their house or favorite place would be considered close or far from the school.
Other groups, namely Hollie and Idil and Dallas and Hope, also asked students to place locations on the map, which created similar opportunities for reasoning authentically about distance.
In addition to leveraging knowledge about distances, students had some opportunity to draw on their funds of knowledge as they decided how to navigate the grids. For example, Whitney described how one student made choices based on his daily routine and the activities he engaged with in different locations:
[The student] started Dash at his house then moved Dash to school. He then coded Dash to visit the Dozier Grill for an after-school snack before heading back home for the evening. I thought it was neat to see this student making personal connections to the activity and getting excited about relating his daily routine to multiplicative thinking with distances.
Brittany and Lily’s lesson gave students a unique way to draw on funds of knowledge in deciding on how to navigate the grid. Although students did not have direct experience as a truck driver picking up supplies from regional distributors (or perhaps even awareness of Corner Market’s use of regional distributors), they had to use their knowledge of perishable food items to make decisions. As Lily noted, the task “set up the coding in a puzzle-like format, where participants would have to critically evaluate the way in which they want the robot to move, and then move and join the puzzle pieces together.” They also needed to consider, however, that ice might melt or milk might spoil during the trip.
The experience gave PTs an opportunity to see family members as home-based resources for mathematics learning. PTs described how students and family members collaboratively engaged in multiplicative reasoning, counting, sequencing, and making sense of distance. PTs agreed with Hope, who noted the importance of family members as resources: “Altogether, we were able to code the mouse to move to its destination.” For example, family members supported mathematics learning by “prompting the kids to look at the [multiplication] chart … or helping them count by 20s” (Adam), listening as students reasoned aloud, “If I go this way … then this will happen.” (Idil), or “helping them count … by pointing and reminding the child” (Hope).
Adult family members also leveraged their own experiences traveling between locations in the community to help students navigate the pathways. For example, Dallas noted how “one child picked [a city three hours away] and the family joked about it only being a few squares away.” Idil described how parents “would help the students with the directions” by reminding them of how they traveled between familiar locations in real life.
Transdisciplinary Connections
Findings are elaborated in this section that showed how PTs leveraged transdisciplinary connections, as other funds of knowledge, in mathematics instruction that used robotics (RQ2). Across all lesson plans, PTs implied connections to cross-curricular content, and their reflections indicated that they valued opportunities for such connections.
For example, Whitney noted that mathematics and robotics integration “is a great opportunity to engage students with cross-curricular learning with vocabulary and literacy,” but efforts to successfully identify explicit connections to disciplinary concepts outside of mathematics varied. All groups were able to identify relevant mathematics standards and practices for their tasks, but in spring 2019 only Hollie and Idil explicitly identified relevant science and English language arts concepts.
In fall 2019, both groups attempted to identify relevant disciplines outside of mathematics, namely computer science (both groups) and English language arts (Dallas and Hope), likely because we encouraged groups to try to identify disciplines outside of mathematics in fall 2019, based on preliminary analyses from spring 2019.
PTs struggled, however, to identify the most appropriate concepts outside of mathematics for their tasks. Across all groups, PTs identified correctly only two standards outside of mathematics for their lessons. Idil and Hollie identified a relevant science standard: “Describe objects accurately by drawing and/or labeling pictures.” This standard was appropriate because they asked students to draw and label familiar community-based locations (Tennessee Department of Education, 2016b, p. 20). Brittany and Lily identified an applicable computer science standard for their Dash Robot activity: “Use a block of code or script from a previous program, identify the control structures in the algorithm such as loops, and/or conditionals in the code” (Tennessee Department of Education, 2018b, p. 18).
Both pairs (Hollie and Idil; Dallas and Hope) who attempted to identify English language arts connections struggled to do so. For instance, Hollie and Idil focused on students’ “describing what would happen if the mouse is programmed in a specific order and they will be identifying cause and effect relationships by using ‘if this, then.’” The third grade standards related to cause and effect relationships, however, did not fit the ways in which students engaged these ideas in the lesson.
Similarly, Dallas and Hope recognized that asking students to create “word problems” that support “children to comprehend how the mathematical tasks fit into the bigger picture” connected to literacy concepts, but they did not name a specific standard. In both cases, Stumbo identified that the most appropriate English language arts standard for the lessons was “Describe people, places, things, and events with relevant details, expressing ideas and feelings clearly” (Tennessee Department of Education, 2016a, p. 48).
Because all groups’ tasks involved coding a robot to navigate a map, implied connections were made to both primary (grades K-2) and intermediate grades (3-5) for geographic concepts in social studies and robotics usage from the technology standards. For instance, Adam, Claire, Peyton, and Whitney included an extension task in which students would engage with the mathematical idea of scale and an actual map of the community to place locations on the grid. PTs, however, did not explicitly identify relevant social studies standards related to geographic concepts of mapping. Based on their knowledge of elementary standards, Stumbo identified relevant transdisciplinary connections across science, social studies, computer science, and fine arts present in the lessons but not named by PTs (Table 2).
Table 2 Transdisciplinary Connections Present in Lesson Plans but Not Identified by Prospective Teachers
| Related Standards | Spring 2019 | Fall 2019 | ||
|---|---|---|---|---|
| Dash | Mouse | Dash | Mouse | |
| Science (TN Department of Education, 2016b) | xx | |||
| Compare and contrast solutions to a design problem by using evidence to point out strengths and weaknesses of the design (p. 29). | xx | |||
| Children form mental models of what science is at a young age (p. 8). | ||||
| Social Studies (TN Department of Education, 2017) | ||||
| Determine the use of diverse types of maps based on the purpose (p. 15). | xx | xx | ||
| Use personal directions such as: up, down, near, far, left, right, in front of, and behind (p. 24). | xx | xx | ||
| Computer Science (TN Department of Education, 2018b) | ||||
| Collaboratively, students can build independence and sophistication using a simple design process to illustrate a program's sequence and outcomes (p. 15). | xx | |||
| Use a block of code or script from a previous program, identify the control structures in the algorithm such as loops, and/or conditionals in the code (p. 18). | xx | |||
| Fine Arts (TN Department of Education, 2018c) | ||||
| Create art that represents natural and constructed environments (p. 151). | xx | |||
Supporting Equitable Mathematics Engagement
Finally, this section describes findings that emerged from our analysis related to access and inclusion in mathematics instruction that leverages robotics, community funds of knowledge, and transdisciplinary connections. PTs emphasized the role of both robotics and funds knowledge in supporting inclusive mathematics engagement. Funds of knowledge provided “opportunities for students to engage with learning using their own experiences” (Whitney), which allowed for multiple entry points, diverse solution paths, and perseverance in solving challenging problems.
For example, Adam described creating multiple entry points by ensuring familiar community connections: “If the students did not recognize any places other than the school, we saw kids draw pictures of their house and add it to our map.” Regarding perseverance, Hollie noted the importance of making the task meaningful by including familiar locations, because “many students who did not correctly program the mouse the first time wanted to keep trying and learning.” They were personally invested. Brittany also shared how this experience helped her realize that children bring home-based experiences with digital technology that can be valuable in mathematics learning:
I went into this STEM Night thinking some students may not be able to figure out how to work the Dash and tablet right away. I was surprised to see how easily every child could work both of these! Through this observation, I was able to learn about students’ experiences. I realized just how much technology they engage with in their lives as they were all so comfortable with using the tablet.
PTs also described how drawing on authentic understandings of distance and relative locations of community places supported problem solving, as previously described.
Similarly, PTs described the important role that technology played in keeping students motivated (because “robots!”; Whitney) and allowing for multiple entry points and solution paths. In terms of initial hook, Lily said, “Since our task took up a large spot on the carpet, involved controlling robots, and had a [local] Corner Market’s sign in the center, our task was quite popular since it was so engaging.” Brittany described how the features of the digital technology allowed for multiple entry points:
Every student was able to get onto the tablet and make the Dash move towards a location of their choosing, the floor task, while some students were immediately able to begin coding and making Dash move to the locations in an order that made the most sense.
Claire agreed that having “several applications on the iPad to move [Dash]” allowed her to adapt the activity “if a student was struggling with one of the apps.” Hollie also emphasized how the technology supported increasing mathematical challenge: “Depending on how quickly students seemed to understand the task, we [could] allow them to create more difficult paths and add obstacles.”
Whitney described the experience as “eye-opening and exciting” because it helped her realize the possibilities for accommodating diverse mathematics backgrounds in a single activity by leveraging both funds of knowledge and technology. Adam described how robotics allowed for diverse solution paths and mathematical engagement:
Some students were able to use mathematical concepts to explain, some students were able to use directional concepts, and some students were able to show you what to do by pointing/telling. It helped me to realize that no matter the task, diverse learners can complete the task, but in their own way.
Overall, PT responses indicated that mathematics lessons integrating both robotics and funds of knowledge make mathematics learning more accessible and engaging for students.
Discussion and Implications
Designing and enacting robotics tasks supported PTs to refine their understanding of the mathematical work inherent in tasks; to recognize community knowledge and family members as resources for mathematics learning; and to use digital technology to differentiate mathematics instruction. This study points to areas of promise and continued refinement for creating a model for mathematics teacher education that moves beyond equipping PTs only with knowledge of community or technological resources. Instead, findings suggest that the STEM night development, implementation, and reflection experience facilitated PTs’ use of such knowledge to put equitable mathematics teaching and learning into practice, at least for those PTs who chose to use robotics.
Integrating both funds of knowledge and technology played a key role in PTs’ development of equitable mathematics teaching. We identified several themes in how PTs developed mathematics teaching that used cultural, linguistic, and cognitive resources from home and community settings to promote learning school mathematics with robotics:
- The choice to integrate robotics supported more meaningful connections to funds of knowledge.
- Sense of place provided an accessible entry point for teaching mathematics with funds of knowledge and technology.
- Inclusion of other content areas helped strengthen connections.
Using Robotics to Support Meaningful Connections
Past research (e.g., Aguirre et al., 2012, Harper et al., 2018) has shown that when PTs attempt to integrate funds of knowledge and children’s mathematical thinking the resulting lessons tend to reflect only limited attention to both. More specifically, these lessons typically include only emergent connections – tasks that resemble traditional school-based tasks, vaguely connected to the community and requiring only low cognitive demand work (e.g., using procedures without meaning; Aguirre et al., 2012).
We observed a similar prevalence of emergent connections across all groups who developed lessons in 2018-19. The four groups who chose to leverage robotics, however, demonstrated more transitional connections – “notable attempts to connect to both [children’s mathematical thinking and community funds of knowledge] in more than superficial ways” (Aguirre et al., 2012, p. 185) but still lacking consistent attention to both. PTs attempted to elicit children’s experiences with traveling between locations in the community or region to emphasize higher cognitive demand work in parts of the task (e.g., sequencing; persevering in problem solving; trial and error; and reasoning about relative distance) while continuing to focus on procedures without connection to meaning or community in other parts (e.g., using multiplication facts).
All PTs promoted at least intermediate levels of sequence learning for students at the family STEM nights as they emphasized that “different sets of instructions can produce the same outcome” (Rich et al., 2017, p. 187) while at other times focusing exclusively on correct or incorrect answers produced through counting or calculations. Although we acknowledge several missed opportunities for more meaningful connections, the choice to use robotics helped push PTs from emergent to transitional connections.
Because school-based lessons rarely use robotics, PTs had no preexisting model for using robotics in a mathematics lesson. Thus, PTs were encouraged to design tasks more similar to those they learned about in the methods course (i.e., open to multiple strategies and focused on reasoning and problem solving), rather than tasks that resembled traditional school-based tasks.
Mathematics teacher educators should encourage PTs to use innovative tools not typically seen in classroom mathematics lessons when learning to leverage funds of knowledge in mathematics. Robotics provides one such example, but other such tools might include nondigital STEM materials (e.g., STEM Magnets Set by Learning Resources; https://www.learningresources.com/stem-magnets-activity-set) or children’s literature, as we saw among other groups in 2018-19. Future research might explore the impact of these types of tools on PTs’ capacity to integrate funds of knowledge into mathematics lessons.
Teachers interested in leveraging robotics and funds of knowledge for mathematics instruction can begin in low-risk spaces where the traditional mathematics routine can be more easily disrupted. For example, several PTs identified stations or workshops – common elementary school routines, in which small groups of students rotate through self-guided mathematics activities with the teacher leading one activity in the rotation – as opportunities to use robotics in their school-based mathematics instruction.
Using Sense of Place as an Entry Point
Research shows how high school and middle school students effectively draw on the capital that sense of place, or place attachment and identity that is contextually dependent, affords them as they engage in doing mathematics with technology (robotics and game design – Leonard et al., 2016; GIS – Rubel et al., 2017). “Spatial tools enable a locally focused curriculum, which addresses the need to contextualize mathematics in relevant questions and representations, especially for low-income youth of color” (Rubel et al., 2017, p. 649).
Using technology to explore place through mathematics shows promise, but the digital technologies used in previous studies are inappropriate for younger students, especially preschool and early elementary students. PTs in this study noted a similar personal investment among preK-5 students because of both the community connections and robotics. Although research shows the accessibility and benefits of using robotics in early childhood and elementary education (Sullivan & Bers, 2016; Xia & Zhong, 2008), little is known about how preK-5 children leverage community connections in learning mathematics with robotics. Robotics may be able to provide an accessible entry point for both elementary teachers and students (based on observations noted in PTs’ reflections) to develop a sense of place that contextualizes mathematics in relevant and meaningful ways.
Future research and teacher education efforts might consider ways to expand the use of robotics as a spatial tool in preK-5 mathematics. Mathematics teacher educators and teachers interested in integrating mathematics, robotics, and sense of place may find Adam, Claire, Peyton, and Whitney, Hollie and Idil, and Dallas and Hope’s approaches especially accessible. Asking students to identify familiar places and navigate robots between community-based places provides a good starting point.
Brittany and Lily’s lesson, however, offers an example of how teachers might continue to expand the ways we use robotics and mathematics to develop students’ sense of place beyond what is already familiar (such as regional distributors used by a local market). Future research might look at the impact of focusing on mathematics and sense of place through robotics on both teacher and student development.
Using Connections to Other Content Areas
One way of expanding the use of robotics as a spatial tool is to emphasize and support connections to other content areas, where leveraging funds of knowledge is more natural for teachers (González et al., 2001). Given the inherently interdisciplinary nature of spatial thinking (Rubel et al., 2017), as evidenced by the implied cross-curricular connections identified here, robotics may help facilitate more meaningful community connections.
Integrating STEAM problem-solving and content disciplines promotes deeper student understanding, multiple ways to approach problems, and increased teacher expertise to navigate multiple content areas (Quigley et al., 2017). Thus, we recommend integrating STEAM problem-based learning with other content areas to ensure more authentic connections. Doing so may be especially important as mathematics teacher educators and teachers strive to transition mathematics with robotics and funds of knowledge from the margins of mathematics instruction to play a role in primary mathematics lessons.
Concluding Thoughts
Guiding PTs to design, plan for, and enact activities at the STEM night required diverse stakeholders to collaborate, including university researchers and teacher educators who supported PTs, principals and teachers at rural and urban schools who planned and hosted the events, community members who discussed mathematics practices with PTs, and families and students who engaged with activities at the STEM night. Future research and teacher education efforts might explore possibilities for stronger collaboration among various stakeholders. Such collaborations might increase opportunities for both PTs and teachers to teach mathematics with robotics and funds of knowledge in traditional classroom spaces. Despite the challenges that come with such an endeavor, this study highlighted the power and possibilities that emerge when various stakeholders collaborate for mathematics teacher education.
Acknowledgements
This research was funded in part by a grant from the Office of Community Engagement and Outreach at the University of Tennessee, Knoxville.
The authors would like to thank Lynn Hodge, the East Tennessee STEM Hub, and the teachers and staff at each elementary school and preschool for organizing and hosting the family STEM nights that created valuable opportunities for PTs’ learning and made this research possible. We also appreciate Joshua M. Rosenberg for providing feedback on earlier versions of this manuscript.
References
Aguirre, J. M., Turner, E. E., Bartell, T. G., Kalinec-Craig, C., Foote, M. Q., McDuffie, A. R., & Drake, C. (2012). Making connections in practice: How prospective elementary teachers connect to children’s mathematical thinking and community funds of knowledge in mathematics instruction. Journal of Teacher Education, 64, 178–192. https://doi.org/10.1177/0022487112466900
Association of Mathematics Teacher Educators. (2017). Standards for preparing teachers of mathematics. amte.net/standards
Benitti, F. B. V. (2012). Exploring the educational potential of robotics in schools: A systematic review. Computers and Education, 58(3), 978–988. https://doi.org/10.1016/j.compedu.2011.10.006
Berry III, R. Q., Ellis, M., & Hughes, S. (2014). Examining a history of failed reforms and recent stories of success: Mathematics education and Black learners of mathematics in the United States. Race Ethnicity and Education, 17(4), 540–568. https://doi.org/10.1080/13613324.2013.818534
Bers, M. U., & Sullivan, A. (2019). Computer science education in early childhood: The case for Scratch Jr. Journal of Information Technology Education: Innovations in Practice, 18, 113–138.
Bian, L., Leslie, S. J., & Cimpian, A. (2017). Gender stereotypes about intellectual ability emerge early and influence children’s interests. Science, 355(6323), 389–391. https://doi.org/10.1126/science.aah6524
Carpenter, T. P., Franke, M. L., Johnson, N. C., Turrou, A. C., & Wager, A. A. (2017). Young children’s mathematics: Cognitively guided instruction in early childhood education. Heinemann.
Chalmers, C. (2018). Robotics and computational thinking in primary school. International Journal of Child-Computer Interaction, 17, 93–100. https://doi.org/10.1016/j.ijcci.2018.06.005
Civil, M. (2002). Everyday mathematics, mathematicians’ mathematics, and school mathematics: Can we bring them together? Journal for Research in Mathematics Education. Monograph, 11, 40–62.
Civil, M. (2007). Building on community knowledge: An avenue to equity in mathematics education. In N. S. Nasir & P. Cobb (Eds.), Improving access to mathematics: Diversity and equity in the classroom (pp. 105–117). Teachers College Press.
Daml, M. (2017). Using community events to enliven STEM education. Teaching Children Mathematics, 23(6), 376–379. https://doi.org/10.5951/teacchilmath.23.6.0376
Esmonde, I. (2009). Mathematics learning in groups: Analyzing equity in two cooperative activity structures. Journal of the Learning Sciences, 18, 247–284. https://doi.org/10.1080/10508400902797958
Forgasz, H. J., Vale, C., & Ursini, S. (2010). Technology for mathematics education: Equity, access and agency. In C. Hoyles & J. B. Lagrange (Eds.), Mathematics education and technology: Rethinking the terrain (pp. 385–404). Springer.
Funke, A., Geldreich, K., & Hubwieser, P. (2017, April). Analysis of scratch projects of an introductory programming course for primary school students. IEEE Global Engineering Education Conference, EDUCON, 1229–1236. https://doi.org/10.1109/EDUCON.2017.7943005
González, N., Andrade, R., Civil, M., & Moll, L. (2001). Bridging funds of distributed knowledge: Creating zones of practices in mathematics. Journal of Education for Students Placed at Risk, 6(1–2), 115–132. https://doi.org/10.1207/S15327671ESPR0601-2
Grubbs, M. (2013). Robotics intrigue middle school students and build STEM skills. Technology and Engineering Teacher, 72(6), 12–16.
Harper, F. K. (2020). Major project. MEDU 530 K-5: Teaching math in the elementary school. https://sites.google.com/utk.edu/medu530k-5/assignments/summative-assignments/major-project
Harper, F. K. (2019). A qualitative metasynthesis of teaching mathematics for social justice in action: Pitfalls and promises of practice. Journal for Research in Mathematics Education, 50(3), 268-310. https://doi.org/10.5951/MTLT.2019.0192
Harper, F. K., Drake, C, Bartell, T. G., & Najarro, E. (2018). “How I want to teach the lesson”: Framing children’s multiple mathematical knowledge bases in the analysis and adaptation of existing curriculum materials. In T. G. Bartell (Ed.), Toward equity and social justice in mathematics education (pp. 241-262). Springer.
Harper, F.K. (2017). Coming to understand the big issues: Remaking meaning of social justice through mathematics across the school year. In A. Chronaki (Ed.), Proceedings of the Ninth International Mathematics Education and Society Conference (pp. 513-521). University of Thessaly Press. https://www.mescommunity.info/mes9b.pdf
Howard, T. C. (2001). Telling their side of the story: African-American students’ perceptions of culturally relevant teaching. The Urban Review, 33(2), 131–149.
Jao, L., & Radakovic, N. (2018). Transdisciplinarity in mathematics education: Blurring disciplinary boundaries. Springer.
Kisker, E. E., Lipka, J., Adams, B. L., Rickard, A., Andrew-Ihrke, D., Yanez, E. E., & Millard, A. (2012). The potential of a culturally based supplemental mathematics curriculum to improve the mathematics performance of Alaska native and other students. Journal for Research in Mathematics Education, 43(1), 75–113. https://doi.org/10.5951/jresematheduc.43.1.0075
Kitchen, R., & Berk, S. (2016). Educational technology: An equity challenge to the Common Core. Journal for Research in Mathematics Education, 47(1), 3-16.
Knight, V. F., Wright, J., & DeFreese, A. (2019). Teaching robotics coding to a student with ASD and severe problem behavior. Journal of Autism and Developmental Disorders, 49(6), 2632–2636. https://doi.org/10.1007/s10803-019-03888-3
Koehler, M. J., & Mishra, P. (2009). What is technological pedagogical content knowledge? Contemporary Issues in Technology and Teacher Education, 9(1), 60–70. https://citejournal.org/volume-9/issue-1-09/general/what-is-technological-pedagogicalcontent-knowledge
Ladson-Billings, G. (2009). The dreamkeepers: Successful teachers of African American children. John Wiley & Sons.
Leonard, J., Buss, A., Gamboa, R., Mitchell, M., Fashola, O. S., Hubert, T., & Almughyirah, S. (2016). Using robotics and game design to enhance children’s self-Efficacy, STEM attitudes, and computational thinking skills. Journal of Science Education and Technology, 25(6), 860–876. https://doi.org/10.1007/s10956-016-9628-2
Leonard, J., Mitchell, M., Barnes-Johnson, J., Unertl, A., Outka-Hill, J., Robinson, R., & Hester-Croff, C. (2018). Preparing teachers to engage rural students in computational thinking through robotics, game design, and culturally responsive teaching. Journal of Teacher Education, 69(4), 386–407. https://doi.org/10.1177/0022487117732317
Li, Q., & Ma, X. (2010). A meta-analysis of the effects of computer technology on school students’ mathematics learning. Educational Psychology Review, 22(3), 215–243. https://doi.org/10.1007/s10648-010-9125-8
Master, A., Cheryan, S., & Meltzoff, A. N. (2016). Computing whether she belongs: Stereotypes undermine girls’ interest and sense of belonging in computer science. Journal of Educational Psychology, 108(3), 424–437.
Master, A., Cheryan, S., Moscatelli, A., & Meltzoff, A. N. (2017). Programming experience promotes higher STEM motivation among first-grade girls. Journal of Experimental Child Psychology, 160, 92–106. https://doi.org/10.1016/j.jecp.2017.03.013
Martin, D. B. (2019). Equity, inclusion, and antiblackness in mathematics education. Race Ethnicity and Education, 22(4), 459-478.
Miles, M. B., Huberman, A. M., & Saldaña, J. (2014). Qualitative data analysis: A methods sourcebook (3rd ed.). Sage.
Moll, L. C., Amanti, C., Neff, D., & Gonzalez, N. (1992). Funds of knowledge for teaching: Using a qualitative approach to connect homes and classrooms. Theory Into Practice, 31, 132–141. https://doi.org/10.1080/00405849209543534
Nasir, N. S. (2002). Identity, goals, and learning: Mathematics in cultural practice. Mathematical Thinking and Learning, 4(2–3), 213–247. https://doi.org/10.1207/S15327833MTL04023
Newton, K. J., Leonard, J., Buss, A., Wright, C. G., & Barnes-Johnson, J. (2020). Informal STEM: Learning with robotics and game design in an urban context. Journal of Research on Technology in Education. https://doi.org/10.1080/15391523.2020.1713263
Nickels, M., & Cullen, C. J. (2017). Mathematical thinking and learning through robotics play for children with critical illness: The case of Amelia. Journal for Research in Mathematics Education, 48(1), 22–77.
Quigley, C. F., Herro, D., & Jamil, F. M. (2017). Developing a conceptual model of STEAM teaching practices. School Science and Mathematics, 117(1–2), 1–12. https://doi.org/10.1111/ssm.12201
Rich, K. M., Strickland, C., Binkowski, T. A., Moran, C., & Franklin, D. (2017). K–8 learning trajectories derived from research literature: Sequence, repition, conditionals. ICER ’17, 182–190. https://doi.org/10.1145/3183508
Rubel, L. H., Hall-Wieckert, M., & Lim, V. Y. (2017). Making space for place: Mapping tools and practices to teach for spatial justice. Journal of the Learning Sciences, 26(4), 643–687. https://doi.org/10.1080/10508406.2017.1336440
Scott, K. A., Sheridan, K. M., & Clark, K. (2015). Culturally responsive computing: A theory revisited. Learning, Media and Technology, 40(4), 412–436. https://doi.org/10.1080/17439884.2014.924966
Sullivan, A., & Bers, M. U. (2016). Robotics in the early childhood classroom: learning outcomes from an 8-week robotics curriculum in pre-kindergarten through second grade. International Journal of Technology and Design Education, 26(1), 3-20.
Taylor, M. S., Vasquez, E., & Donehower, C. (2017). Computer programming with early elementary students with down syndrome. Journal of Special Education Technology, 32(3), 149–159. https://doi.org/10.1177/0162643417704439
Thomas, A., & Edson, A. J. (2019). A framework for mathematics teachers’ evaluation of digital instructional materials: Integrating mathematics teaching practices with technology use in K-8 classrooms. Contemporary Issues in Technology and Teacher Education, 19(3), 351–372. https://citejournal.org/volume-19/issue-3-19/mathematics/a-framework-for-teachers-evaluation-of-digital-instructional-materials-integrating-mathematics-teaching-practices-with-technology-use-in-k-8-classrooms
Tennessee Department of Education. (2016a). Tennessee English language arts standards. https://www.tn.gov/content/dam/tn/stateboardofeducation/documents/massivemeetingsfolder/meetingfiles4/10-20-17_III_J_Non-Substantive_Changes_to_Math_ELA__Science_Standards_Attachment_2_-_ELA.pdf
Tennessee Department of Education. (2016b). Tennessee academic standards for science. https://www.tn.gov/content/dam/tn/stateboardofeducation/documents/massivemeetingsfolder/meetingfiles4/10-20-17_III_J_Non-Substantive_Changes_to_Math_ELA__Science_Standards_Attachment_3_-_Science.pdf
Tennessee Department of Education. (2017). Tennessee social studies standards. https://www.tn.gov/content/dam/tn/education/standards/ss/Social_Studies_Standards.pdf
Tennessee Department of Education. (2018a). STEM strategic plan: An integrated K-12 STEM proposal for Tennessee. https://www.tn.gov/content/dam/tn/education/ccte/ccte_stem_strategic_plan.pdf
Tennessee Department of Education. (2018b). Digital readiness: K-8 computer science standards. https://www.tn.gov/content/dam/tn/stateboardofeducation/documents/2018_sbe_meetings/june-27,-2018-sbe-meeting/7-27-18%20III%20I%20K-8%20Computer%20Science%20Standards%20Clean%20Copy%202.pdf
Tennessee Department of Education. (2018c). Tennessee academic standards for fine arts. https://www.tn.gov/content/dam/tn/education/standards/art/Fine_Arts_Standards.pdf
Turner, E., Aguirre, J., Drake, C., Bartell, T. G., Roth McDuffie, A., & Foote, M. Q. (2015). Community mathematics exploration module. In C. Drake, J. M. Aguirre, T. G. Bartell, M. Q., Foote, A. Roth McDuffie, & E. E. Turner (Eds.), TeachMath learning modules for K-8 mathematics methods courses (Teachers Empowered to Advance Change in Mathematics Project). Retrieved from: http://www.teachmath.info
Wang, J., & Moghadam, S. H. (2017). Diversity barriers in K-12 computer science education: Structural and social. In SIGCSE 2017 – Proceedings of the 48th ACM Technical Symposium on Computing Science Education (pp. 615–620). Association for Computing Machinery
Warschauer, M., & Matuchniak, T. (2010). New technology and digital worlds: Analyzing evidence of equity in access, use, and outcomes. Review of Research in Education, 34(1), 179–225. https://doi.org/10.3102/0091732X09349791
Yuen, T. T., Boecking, M., Tiger, E. P., Gomez, A., Guillen, A., Arreguin, A., & Stone, J. (2014). Group tasks, activities, dynamics, and interactions in collaborative robotics projects with elementary and middle school children. Journal of STEM Education: Innovations and Research, 15(1), 39–46.
Xia, L., & Zhong, B. (2018). A systematic review on teaching and learning robotics content knowledge in K-12. Computers and Education, 127(122), 267–282. https://doi.org/10.1016/j.compedu.2018.09.007
Appendix
![]()