In Making Reform Work, Robert Zemsky described educational technology as an unfulfilled promise. He noted that the 1990s brought fanfare of a forthcoming educational revolution, spurred by new technologies, which would fundamentally change how teachers teach and how students learn. The anticipated sea change never came, and “the first decade of the 21st century has proved to be one of lowered expectations” (Zemsky, 2009, p. 145). He proposed that a major reason for educational technology’s disappointing impact is related to its “supply side” production.
Technology creators are typically detached from the sites of teaching and learning and develop products envisioned as being generally useful to teachers. These products, though, are not tailored to specific purposes in particular places, nor are they typically developed in response to the needs identified by educators on the ground. Zemsky suggested that a move toward creating technology that genuinely improves teaching and learning “will require faculty who seek new ways to solve old riddles and technologists who understand that their business is using technology to help people solve their own problems” (p. 154).
During the past 3 academic years we have been collaboratively engaging with the puzzle of how to help kindergarteners make sense of numbers, and we have developed software as one tool in this effort. Our collaborators include dozens of undergraduate preservice teachers enrolled in our elementary education program and two kindergarten teachers at a partnering elementary school. While the primary goals of our kindergarten Number Sense Project (NSP) have been to bolster the children’s number readiness and to improve the quality of our teacher education program, our development of software as an instructional tool in the project has given us some insight into Zemsky’s vision of demand side technology production: the creation of software built for a specific purpose in a particular school and developed in response to needs identified by local teachers.
This paper focuses on the role our software has played in the project and the manner in which it has been developed with both preservice and in-service teachers. Details about the design and rationale of the software, the collaborative development process, indications about its impact on teachers’ practice, and discussion about the potential of demand-side production of educational technology is described at length in this paper.
The Kindergarten NSP
The NSP is one aspect of an intensive partnership between the Augustana College Education Department and Longfellow Elementary School in Rock Island, Illinois. Augustana is a liberal arts college serving undergraduate students exclusively. Longfellow is a public elementary school located a few blocks from the College. Longfellow has a diverse student body with approximately 45% of students classified as Hispanic, 31% White, 14% Black, and 8% multiracial (Illinois State Board of Education, 2011). Approximately 85% of Longfellow’s students are considered to come from families with low income. The mutually beneficial partnership between Augustana and Longfellow began in earnest during the 2009-2010 school year. All junior elementary education majors from the college conduct their clinical work at Longfellow, observing classrooms, assisting teachers, and implementing some complete lessons at the school. In addition to their clinical hours, many collegiate education courses require students to plan, implement, and assess lessons with Longfellow students, thus enabling preservice teachers to apply their knowledge of teaching methods in real classrooms as part of their coursework.
The NSP represents an effort to optimize the benefits of this partnership in the area of mathematics education. The project has two main goals: (a) to enrich the teacher education experience at Augustana and (b) to impact mathematics learning positively among the elementary students at Longfellow. We address the first goal by providing our preservice teachers numerous opportunities to engage in the teaching cycle (i.e., assessing student knowledge, planning appropriate learning experiences for students, implementing instruction, reflecting on teaching and learning) with real students.
These teaching experiences begin during Augustana’s fall trimester (late August-early November). During that term the college students enrolled in the course, Teaching Mathematics in the Elementary School, are matched with Longfellow’s kindergarteners such that pairs of undergraduates work with groups of four to five children. Under the supervision of their college instructor (one of the authors) and the kindergarten teachers, the preservice teachers assess the numerical knowledge of the children individually and implement weekly learning experiences tailored to the children’s needs.
The College provides a fund of $2,000 per student that enables us to hire interested undergraduates drawn from the teaching methods course to continue working closely with the kindergarten for the remainder of the school year. These students spend 4 to 5 hours each week in the classroom, working closely with the kindergarten teachers in planning and implementing small-group and individual instruction for the children.
In addition to the supplemental teaching experience these students gain from their participation, they are also expected to provide feedback on the usefulness of the project software with children, suggest new software ideas, conduct a research project related to their work, and publicly present the results of their research at a professional conference. The NSP affords preservice teachers opportunities to engage in both teaching and research beyond the baseline requirements of clinical work.
Focusing on kindergarten numeracy is a promising strategy for meeting the project’s second goal of bolstering mathematics learning at Longfellow. A substantial body of research suggests that kindergarten is a pivotal year for children to develop foundational ideas about whole numbers. In their synthesis of early childhood mathematics research, Clements and Sarama (2007) concluded that the numerical competencies children develop before first grade are better predictors of subsequent mathematics achievement than other abilities “such as visual attention, metacognitive knowledge, and listening comprehension” (p. 478). Similarly, Jordan, Kaplan, Ramineni, and Locuniak (2009) tracked approximately 200 children from kindergarten through third grade and found that “higher levels of kindergarten number competence predicted statistically significant and substantively meaningful performance in composite mathematics achievement at the end of third grade” (p. 861).
Although kindergarten numeracy is vital for subsequent growth, children enter kindergarten with a wide range of background knowledge about numbers. Crafting a learning program appropriate for all (or even most) learners of this age is impossible: “A neat linear description of developmental stages will always be complicated by the exigencies of individual differences in cognition and experience” (Verschaffel, Greer, & De Corte, 2007, p. 591). Differences are particularly problematic in a setting such as Longfellow, where the majority of children come from low income families and relatively few children enter kindergarten with a formal preschool experience (National Research Council, 2009).
Thus, kindergarten numeracy became the focus of the extra teacher personnel the Augustana/Longfellow partnership would provide for the school. The presence of additional preservice teachers in the kindergarten has enabled the children to receive individualized attention in an area of instruction that has been shown to be highly predictive of future academic success (Jordan, Kaplan, Olah, & Locuniak, 2006; Jordan et al., 2009).
Collaboratively Developed Software as a Tool for Promoting Early Numeracy
The undergraduate participants supported the efforts of the regular kindergarten teachers in providing helpful numerical learning experiences for the children. These experiences include a variety of age-appropriate instructional approaches, such as the use of manipulatives, movement-based activities, mathematical children’s stories, and songs. An additional teaching tool that is utilized extensively during the individualized learning sessions is the collaboratively developed computer software built specifically for the project.
Although educational software has been readily available for the past 30 years, the early childhood education community initially resisted the use of computers for teaching young children. Many educators, influenced by Piaget’s construct of the early childhood concrete operations developmental stage, assumed that young children require physical play and the ability to manipulate concrete objects in order to learn abstract concepts. However, by the mid-1990s researchers found that the virtual manipulations facilitated by computer software could be analogous to physical manipulations and, thus, could effectively support children’s mathematical development (Clements, 1999, 2000; Yelland, 1998).
Today, the use of mathematical technology in elementary classrooms is expected. It is included as one of eight Standards for Mathematical Practice in the Common Core State Standards for Mathematics (Common Core State Standards Initiative, 2010, p. 7). The National Council of Teachers of Mathematics (NCTM, 2000) also encouraged the use of computer applications in elementary instruction, but emphasized that teachers play an essential role in ensuring that the technology is used effectively: “As with any teaching tool, [technology] can be used well or poorly. Teachers should use technology to enhance their students’ learning opportunities by selecting or creating mathematical tasks that take advantage of what technology can do efficiently and well” (p. 26).
Although the mathematics education community agrees that computer technology has the potential to promote rich mathematical thinking that would not be available without technology, research suggests that software is not being harnessed in this manner on a large scale. For instance,Yelland (2005) reviewed the literature on computer use in early childhood numeracy and found that
most examples of the use of computers in literacy and numeracy have tended to be with computer assisted learning (CAI) software and the drill and practice genre which reinforce “old learning” and emphasize the acquisition of skills in a vacuum with no attempt to relate them to authentic activity. (pp. 207-208)
Not only does this kind of mass-produced software fall short of promoting the kinds of higher order thinking suggested by the Common Core Standards for Practice and the NCTM Process Standards, but evidence also exists that school districts that purchase and use this software are seeing no real gains in test scores (Viadero, 2009). Zemsky (2009) and others (e.g., Watters, 2011) have suggested that a more effective model for software development would involve the inclusion of on-the-ground teachers in the design process so that they can help customize the power of computers to meet the needs of their own students.
The writing of Koehler and Mishra (2005; Koehler, Mishra, Hershey, & Peruski, 2004) in the area of technological pedagogical content knowledge supported the notion that the educative potential of technology can be optimized when teachers are involved in design. Focusing on the efforts of university teachers designing web-based blended learning environments, Koehler et al. (2004) argued that participation in design enriches teachers’ sense of how technology can enhance the learning of content. As Koehler and Mishra (2005) noted, “Most significantly, by participating in design, teachers build something that is sensitive to the subject matter (instead of learning the technology in general) and the specific instructional goals (instead of general ones)” (p. 135). The collaborative design work with kindergarten teachers likewise orients toward the specific content needs and instructional goals that surface as local kindergarteners make sense of numbers.
The Collaborative Development Process
The goal of the software development piece is twofold. First, we want to model the development and use of small, focused software programs designed to meet the needs of kindergarteners in a particular classroom. Second, we want teachers to see themselves as not just software users, but as software designers. In short, we want the teachers to develop ideas without requiring them to learn how to write computer programs. Prior to the beginning of the 2009-2010 school year we created a set of highly focused software applications developed specifically to complement the goals of our project and illustrate for our preservice teachers and the kindergarten teachers what was possible. After the initial packages were made, the teachers themselves became the primary generators of content-related ideas for the applications.
All of our software was built with LiveCode® from Runtime Revolution® (http://www.runrev.com), a rapid development environment that allows users to build applications quickly for multiple platforms. Hence, when a kindergarten teacher or preservice teacher suggests an idea for a piece of software, we are typically able to produce it within a week. During the 2009-2010 school year our software was built for Macintosh® and Windows® platforms, and these versions are available for free download at http://www.augustana.edu/numbersense. Since the 2010-2011 year, we have focused our development on mobile versions for Apple® devices that can be downloaded through iTunes® and our website. The kindergarten classrooms have the hardware to run these applications. Each classroom is equipped with Macintosh computers, our College has provided six iPod Touches® for the children to use, and our undergraduate teaching assistants have iPads® that they use with the children.
Our software design process has had three main components: (a) research and development of the initial programs prior to implementation; (b) modification of existing programs during the school year in order to better meet the unfolding needs of the children; and (c) creation of new applications in response to teacher requests.
Initial Applications
We began developing our Number Sense software in winter 2009, several months prior to its implementation in the Longfellow kindergarten. We set out to design electronic learning experiences that would be reasonably related to mandated kindergarten learning targets (NCTM, 2006, Curricular Focal Points prior to fall 2011 and Common Core standards beginning in the fall of 2011) and also to age-appropriate learning goals discussed in literature on early childhood mathematics. The work of Fuson, Grandau, and Sugiyama (2001) and Van de Walle (2004) proved particularly useful as we designed programs meeting these goals. These authors have developed frameworks describing the numerical concepts children should know in kindergarten, what they can know, and what their general learning trajectory will be as they move toward what they will know. These concepts include the ability to relate words, numerals and physical referents; the ability to recognize the cardinality of a set; the development of a spatial recognition of numbers; the ability to partition numbers, and the ability to connect numbers to important benchmarks such as 5 and 10.
The learning activities we developed provided opportunities for different students to work with concepts most appropriate for them. The kindergarten NSP was integrated into the fall Math Methods course that isa course requirement for elementary education majors. Many of the initial software titles corresponded to suggested learning activities described in the Van de Walle (2004) text used in the methods course. Table 1 summarizes some of the main learning goals identified in Fuson et al. (2001) and Van de Walle (2004), the software we developed to address these learning goals, and related Common Core Standards.
Table 1
Kindergarten Learning Goals With Software-Based Learning Activities
| Learning Goal[a] | Software Activity | Related Common Core Standards |
| Be Able to “Break Apart Numbers” Up to 10 (e.g., recognize that a collection of 9 can be broken down into a collection of 5 and a collection of 4; or different smaller collections such as “2,” 3” and “4”…) | Count Sort Ah Chute Pattern Sets Balance Math What’s Hiding? | K.OA.3: Decompose numbers less than or equal to 10 into pairs in more than one way, e.g., by using objects or drawings, and record each decomposition by a drawing or equation. |
| View Teen Numbers as One Group of Ten and Some Loose Ones | Pattern Sets
| K.NBT.1: Compose and decompose numbers from 11 to 19 into ten ones and some further ones. |
| Count a Disorganized Collection of Objects to 32 | Count Sort | K.CC.5: Count to answer “how many?” questions about as many as 20 things. |
| Begin a Basic “Counting On” Strategy for Addition | Word Problems | K.CC.2: Count forward beginning from a given number within the known sequence (instead of having to begin at 1). |
| Efficiently Recognize Numbers That are One More/Less or Two More/Less Than Another | Pattern Sets | K.CC.4c: Understand that each successive number name refers to a quantity that is one larger. |
| Master Number “Families” (especially 5 and 10) (e.g., know all of the 5 families, 0+5, 1+4, 2+3; all the 10 families; recognize facts such as “3 is 2 less than 5,” “7 is 2 more than 5”; “7 is also 3 less than 10.” | Count Sort Ah Chute Balance Math What’s Hiding? Pattern Sets | K.OA.4: For any number from 1 to 9, find the number that makes 10 when added to the given number. K.OA.5: Fluently add and subtract within 5. |
| [a] Gleaned from Fuson et al. (2001) and Van de Walle (2004) | ||
Though the initial applications were influenced primarily by our own experience with and research of early childhood numeracy, we received feedback from both of Longfellow’s kindergarten teachers and from kindergarten-aged children throughout this period that enabled us to refine the software in time for the new school year. We shared our progress on the software with the teachers during our spring 2009 planning meetings, seeking their input on the potential usefulness of the programs. As we built drafts of the software in the early months of 2009, we informally tested each package with the kindergarten-aged daughter of one of the authors in a preliminary effort to verify that the programs would be appropriate for children. We did more extensive field-testing over the summer with a group of five 4- to 6-year-old children. The teachers’ comments about and the children’s reactions to the software influenced the final versions, which were used in the kindergarten during the 2009-2010 school year. The final versions are described briefly in the appendix.
Modifications During the School Year
Both the preservice teachers and the kindergarten teachers began using these applications as part of their mathematical work in the kindergarten beginning in the 2009-2010 academic year. As the teachers witnessed children’s reactions to the software, and also as they began to recognize new learning needs for their students, they were able to share ideas with us about how the software should be modified to better meet the needs of the classroom.
For example, Count Sort was originally built as a simple program for fostering early counting. It provided white circles which turned blue when clicked (in order to help children recognize the one-to-one nature of counting, so that counted objects could be set apart from uncounted objects) and also enabled children to begin forming basic number families (e.g., 3, 2, and 5 are an additive “family”). As our preservice teachers worked with the kindergarteners, they found that the children also needed help in recognizing the meaning of numerals and in being able to estimate a quantity of objects by sight. The preservice teachers suggested that Count Sort could be modified to incorporate these other skills. Consequently, we added two new options to the existing package. The first was a numeral recognition option in which a menu of two to four numeral options would appear after the child had counted the last circle; the child would then be prompted to choose which numeral represented the amount of circles just counted (see Figure 1). The second new option related to estimation in that the menu of numeral choices appeared before the child began counting the circles, thus prompting the child to estimate the quantity prior to counting (See Figure 2).
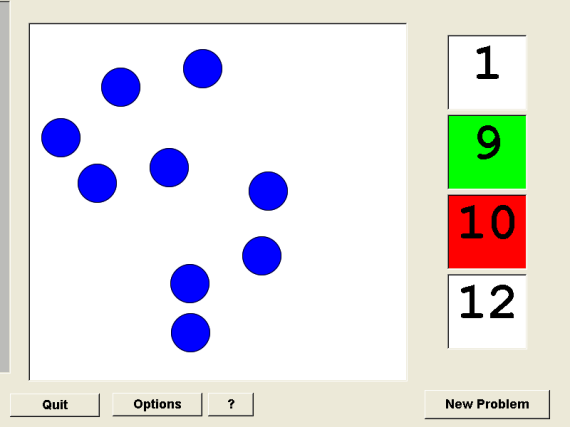
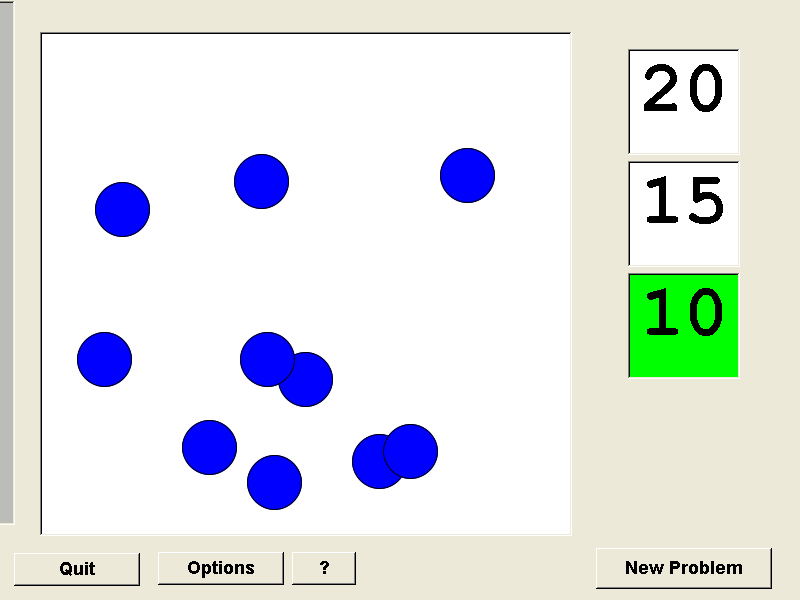
Figure 1. After the child counts the chips, number choices appear as another representation of the amount counted.
Figure 2. In the estimation option, the number choices appear before the child begins to count.
Another example of the modification process can be seen with Number Line Math. That program was originally developed for a different project. However, it was integrated into the NSP project when several of the preservice teachers indicated that some of the kindergarten students were ready to work on addition problems in an “x + y = ?” format. Focused on facts 1-10, the original version (Figure 3) made use of the number line and had options for addition and subtraction. It also required the user to click on a “?” to indicate readiness to answer the problem and then type the answer. The suggestions from the preservice teachers were to have the student click on the number line to indicate the answer, to show only addition or subtraction buttons rather than both at once, and to have the option to focus on a smaller range of facts. The new version (Figure 4) has options for 1-5, 6-10, and 1-10.

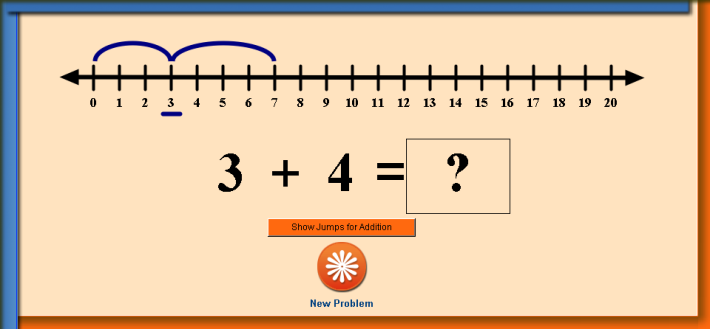 Figure 3. The original version of Number Line Math. Figure 4. The modified version based on undergraduate assistants’ feedback.
Figure 3. The original version of Number Line Math. Figure 4. The modified version based on undergraduate assistants’ feedback.
Teacher Requested Software
The kindergarten teachers began suggesting original ideas for software during the second year of the program, 2010-2011. By this time we had been present in the teachers’ classrooms for several months, our preservice teachers had shared ideas for software modifications, and the in-service teachers had been holding regular planning sessions with the undergraduates who worked at the school throughout the year. Conversations during those sessions regularly included discussions as to the purposeful use of our small-scale programs in supporting their children’s learning. In short, the teachers began to move toward our goal of helping them see themselves as software designers: individuals who could use their knowledge of students’ learning needs as a basis for proposing new applications for the computer.
One teacher-generated idea, 10-Frame Fill (Figure 5), was specifically meant to help the kindergarten students use a 10-frame to build their knowledge about 10 families (e.g., pairs of whole numbers whose sum is 10). The Longfellow mathematics curriculum emphasizes the use of 10 frames as a strategy for meeting Common Core Standard K.OA.4: “For any number from 1 to 9, find the number that makes 10 when added to the given number.” The first version of the software we designed to address this learning goal simply displayed a 10-frame with a random number of chips placed in the frame. There was no number entry mechanism, so the kindergarten student would tell the preservice teacher the answer. Subsequent suggestions from the preservice teachers included the use of a number pad to enter the answer and allowing users to drag different colored discs into the 10-frame.
A second software program that emerged from the in-service teachers was Line ‘em Up (Figure 6). One of the teachers regularly implemented an activity with the children in which she would shuffle a deck of cards showing the numerals 1-20, and then ask the children to organize the cards by laying them down face-up in numerical order. Many of her students had difficulty completing this task. Our Line ‘em Up game is simply an electronic representation of this game with the added advantage of facilitating practice and repetition with less need for intensive teacher oversight. As our preservice teachers began to use this tool with the children, they began to suggest ways of improving it further.
For example, the original version highlighted the correct point on the number line when the user dragged the number card to the correct place. Our college students indicated that the kindergarteners began looking for the highlight rather than using the numbers already placed to determine where to put a number card. The program was then changed to display the highlight only after the student “dropped” the number card into place. Another undergraduate-initiated option was added to the default one-card-at-a-time view (shown in the illustration) to include an option to view all cards at once randomly placed and scattered below the number line.

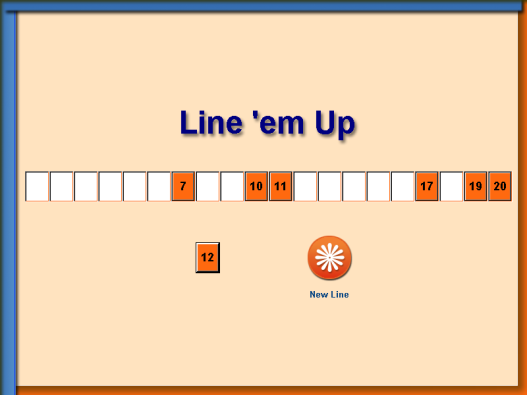
Figure 5. 10-Frame Fill
Figure 6. Line ‘em Up
The third year of our partnership, 2011-2012, marked the first year that Longfellow’s teachers would be held accountable for addressing the Common Core Standards over the year. Thus, this was the first year in which the children would be expected to represent sums and differences with symbolic equations as indicated by the Kindergarten Operations and Algebraic Thinking standards. The teachers were aware of existing software applications designed to enable older elementary students to practice their arithmetic facts, but these applications seemed too advanced for kindergarteners as they lacked age-appropriate visual supports. They proposed yet another software idea to us, one that would prompt kindergarteners to solve only simple addition and subtraction problems and also provide support via visuals and virtual manipulatives. This idea resulted in the Add Sub K-1 iPad app, a program that enables teachers to determine the addends and subtrahends that will be used (and hence control the complexity of the equations) and also provides children with supports for modeling equations. These supports include dragable shapes (Figure 7) and a writing board for making tally marks (Figure 8).
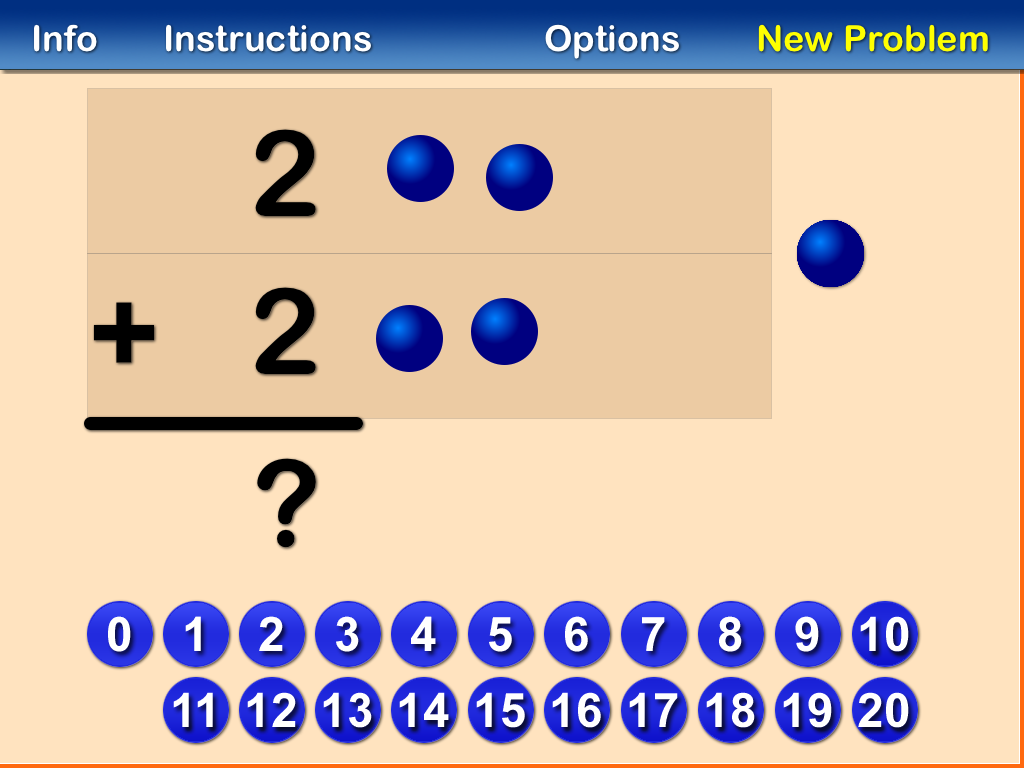
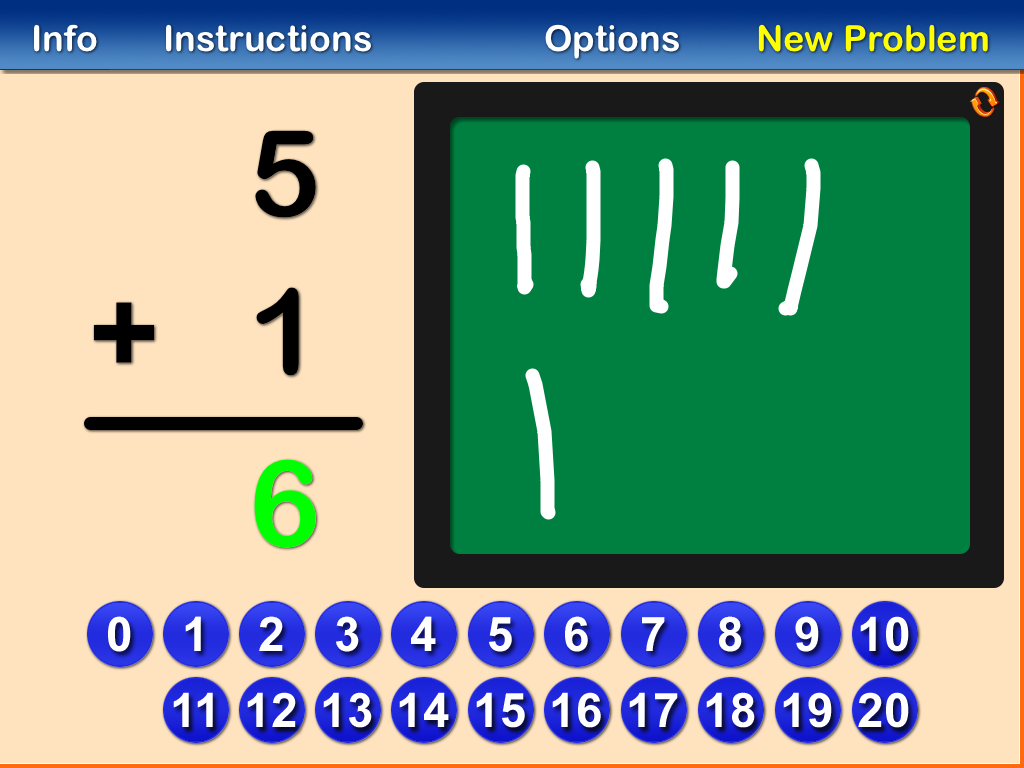
Figure 7. Dragging shapes option in Add Sub K-1
Figure 8. Tally option in Add Sub K-1
Teachers as Software Developers
These software applications represent one instructional tool among many used in the kindergarten NSP efforts to provide individualized support of children’s learning and rich experiential opportunities for preservice teachers. Of course, the use of software as a teaching tool is widespread. Our work is distinctive in that local teachers and preservice teachers have been active members of the design team. These educators have been able to propose ways that the computer or tablet might address particular learning struggles encountered in their own classrooms and, thus, have been able to help create the applications they feel they need rather than having to shop around for some piece of software that might help them. This aspect of our collaboration was highlighted by one of our teacher-collaborators:
In the past, if my students or I had a specific need, I then searched for the most appropriate software program, online service or piece of hardware that could best meet those needs. I also frequently attended local and state technology workshops and conferences to ask others for their suggestions/successes….What I’ve never experienced is being able to ask for a specific software program or game to custom-fit that need! That was amazing. When we thought our students needed more practice and reinforcement in counting skills, for example – you created fun and motivating programs to do that.
Our description of the collaborative design process presented earlier in this paper provides some evidence of the in-service and preservice teachers’ levels of engagement with this endeavor. This experience has been positive for the teachers involved and has opened up for them a new and potentially powerful approach for supporting their students’ learning.
I thought it was great when we could specifically identify which skills the children needed extra support and practice by using the observational assessments each month with [our curriculum]. We could then share those students’ weak areas and determine a computer game for extra practice. It also worked for creating challenge activities and games for those students with math skills exceeding the classroom norms.
Although our primary mission is to serve our local elementary school, our move toward developing mobile apps and distributing them through iTunes® has broadened the scope of our collaborative community. iTunes enables app users around the world to provide feedback to developers about apps and suggest changes. We actively invite users to communicate with us in detail via email, as well. The following message from a teacher in Ohio suggests that practitioners beyond our local community are eager to participate in the individualized software design process:
Do you take app “requests”? I teach in the Mason City School District in Ohio. We are a top-rated district in our state with high expectations and high achievement. As we transition to iPads with our staff and students, I know we are going to think, “We wish there was an app for…” or “We wish there was an app that…” (I know after having my iPad for 2 months and researching teacher productivity apps and student learning apps I already have a big list of “I wish…” for apps! (J. Davis, personal communication, January 7, 2012)
Demand-Side Technology Production: Final Considerations
Readers who peruse our Number Sense software will quickly note that its features and functions are simple. Graphics consist largely of basic shapes and colors, animation is minimal, and most of our packages were built without sound effects in order to minimize classroom distractions. In brief, our software would never hold broad-based commercial appeal, but that is the whole point. Our goal is to address immediate learning needs in two local classrooms. One team member has described our applications as just-in-time software, or software built soon after the moment when a teacher identifies a learning need of her students and wonders if an application can be built to address that need.
In the vital area of kindergarten numeracy, the immediate learning needs of children can be difficult for adults to anticipate (Verschaffel et al., 2007); hence, ongoing production of simple applications to address basic learning goals holds potential as an avenue toward fostering children’s number sense. Members of our team are often surprised by the seemingly simple conceptual hurdles young children encounter as their knowledge of numbers develops, yet these hurdles can often be cleared with the assistance of simple technological interventions.
For example, our preservice teachers recently noticed that many kindergarteners are in the habit of counting up from 1 every time they are attempting to place a particular number card in its proper place as they play the Line ‘em Up game. Of course, this is an acceptable and understandable strategy for this age group: As with the alphabet, most novice counters need to recite the entire sequence before knowing a given number’s position relative to other numbers. Still, our preservice teachers recognized that an important developmental step for these children will be to recognize instantly, for example, that 9 follows 8 and precedes to 10 without having to count up from 1.
As a means of helping the children develop such automaticity, our preservice teachers requested that we modify Line ‘em Up to include an option whereby the sequence of visible number cards on the screen begins with a number other than 1, thus prompting the user to consider another strategy when ordering numbers. This is just one instance of our project imagining and acting on ideas for meeting children’s often-unanticipated learning needs via technology as they surface.
Although we have found the production and implementation of small-scale, locally focused applications helpful at our site, the question of how realistic or effective this would be on a broader scale remains unanswered. Most classrooms will not have access to a programmer who can tailor applications to teacher requests; thus, the supply-side model of creating and providing applications of general use might seem like the only practical approach for distributing worthwhile technology across thousands of classrooms. Such technology has certainly proven to be worthwhile. Numerous mass-produced applications are valuable in educational settings. However, the teaching and learning process can be enhanced through targeted applications responding to local needs, an approach that might be fostered on a large scale. For example, one could imagine a generic application that could enable teachers to produce independently their own simple applications for their own students. A meta-application of this type might provide teachers with intuitive menus and visual prompts, thus sidestepping the need for familiarity with programming languages.
Teachers’ products would not be flashy, but they could help individual teachers meet very specific learning goals that may not be adequately addressed by commercial applications. Indeed, teachers are accustomed to tailoring learning experiences outside of the realm of technology. While the main textbook may provide the majority of learning materials for a classroom, most teachers will find ways to supplement the commercial textbook with homemade materials built to meet a specific learning objective. Educational technologists would do well to facilitate such efforts in the realm of technological applications as well.
References
Clements, D.H. (1999). ‘Concrete manipulatives,’ concrete ideas. Contemporary Issues in Early Childhood, 1(1), 45-60.
Clements, D.H. (2000). From exercises and tasks to problems and projects: Unique contributions of computers to innovative mathematics education. Journal of Mathematical Behavior, 19, 9-47.
Clements, D. H., & Sarama, J. (2007). Early childhood mathematics learning. In F. K. Lester (Ed.), Second handbook of research on mathematics learning and teaching (pp. 461-555). Charlotte, NC: Information Age Publishing.
Common Core State Standards Initiative. (2010). Common core state standards for mathematics. Retrieved from http://www.corestandards.org/assets/CCSSI_Math%20Standards.pdf
Fuson, K. C., Grandau, L., & Sugiyama, P. A. (2001). Achievable numerical understandings for all young children. Teaching Children Mathematics, 7, 522-526.
Illinois State Board of Education. (2011). Illinois school report card: Longfellow Liberal Arts Elementary School. Retrieved from http://webprod.isbe.net/ereportcard/publicsite/getReport.aspx?year=2011&code=4908104102015_e.pdf
Jordan, N., Kaplan, D., Olah, N., & Locuniak, M. (2006). Number sense growth in kindergarten: A longitudinal investigation of children at risk for mathematical difficulties. Child Development, 77(1), 153-175.
Jordan, N.C., Kaplan, D., Ramineni, C., & Locuniak, M.N. (2009). Early math matters: Kindergarten number competence and later mathematics outcomes. Developmental Psychology, 45(3), 850-867.
Koehler, M.J., & Mishra, P. (2005). What happens when teachers design educational technology? The development of technological pedagogical content knowledge. Journal of Educational Computing Research, 32(2), 131-152.
Koehler, M.J., Mishra, P., Hershey, K., & Peruski, L. (2004). With a little help from your students: A new model for faculty development and online course design. Journal of Technology and Teacher Education, 12(1), 25-55.
National Council of Teachers of Mathematics. (2000). Principles and standards for school mathematics. Reston, VA: Author.
National Council of Teachers of Mathematics. (2006). Curricular focal points for prekindergarten through grade 8 mathematics. Reston, VA: Author.
National Research Council. (2009). Mathematics learning in early childhood: Paths toward excellence and equity. Washington, DC: The National Academies Press.
Van de Walle, J. A. (2004). Elementary and middle school mathematics: Teaching developmentally (5th ed.). Boston, MA: Pearson Education, Inc.
Verschaffel, L., Greer, B., & De Corte, E. (2007). Whole number concepts and operations. In F. K. Lester (Ed.), Second handbook of research on mathematics learning and teaching (pp. 557-628). Charlotte, NC: Information Age Publishing.
Viadero, D. (2009). Reading, math software found to have little effect on scores. Education Week, 28(25), 8.
Watters, A. (2011, December 6). As the ed-tech marke grows, what voice do educators have? [Blog]. Retrieved from the Edutopia website: http://www.edutopia.org/blog/educator-voice-in-edtech-diy-audrey-watters
Yelland, N.J. (1998). Empowerment and control with technology for young children. Educational Theory and Practice, 20(2), 45-55.
Zemsky, R. (2009). Making reform work: The case for transforming American higher education. New Brunswick, NJ: Rutgers University Press.
Author Notes
Mike Egan
Augustana College
Email: [email protected]
Randy Hengst
Augustana College
Email: [email protected]
Appendix
Descriptions of Initial Software Packages
| Initially Developed Software: Titles and Descriptions | Screen Shots |
| Ah Chute This “fill the chutes” game was designed to promote basic counting, number recognition, help children begin to organize numbers into groups of 5, and recognize “5 families” (e.g. 1 & 4, 2 & 3, 1 & 2 & 2, etc.). It was modeled after the example on page 117 of the Van de Walle (2004) text.
| 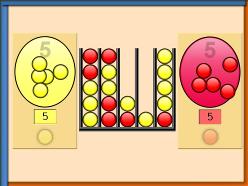 |
| Balance Math Using proportional rectangles modeled after Cuisenaire® rods, this game helps children build various number “families” and introduces an early concept of equations and inequalities. Options include using values 1-5 or 1-10 and creating situations where the user balances using addition or subtraction.
| 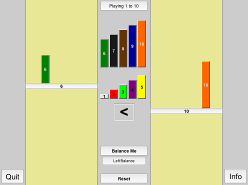 |
| Count Sort A very basic game for children just beginning to learn about numbers, Count Sort helps children recognize that counting must be done in a one-to-one fashion and also develops early recognition of number “families.” The program randomly generates a quantity of white circles which change to blue when clicked, hence the program facilitates the one-to-one skill of recognizing what has been counted and what has not. Children can also build number families by sorting the circles into subgroups. In the image shown here, 8 circles have been sorted into a “5, 3, 8” family. | 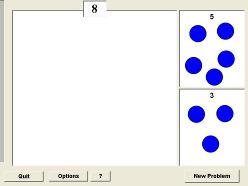
|
| Pattern Sets This software title was modeled after the information on pattern set recognition (Van de Walle, 2004, pp. 120-121). We added 10-frame patterns to the pattern designs suggested in the text (see the “Ten-Frame Flash” activity p. 123 of Van de Walle, 2004). Intended for more advanced kindergartners, Pattern Sets requires children to recognize visual representations of numbers quickly without having to count. This is an advanced application of number family recognition. Using the screenshot at right as one example, children will need to “know” that one group of 2 and one group of 3 is 5. Patterns are shown and then hidden in adjustable time increments from .1 to 2.5 seconds. |  |
| What’s Hiding? Designed for children who are ready to start applying their counting and number family recognition skills in the areas of basic addition and subtraction, this provides visual support as children attempt to identify missing addends. The program begins by revealing a particular number of white circles which turn blue as the child clicks/counts them. Once all circles have been counted, the numeral representing the amount counted is revealed and the circles are hidden under a rectangle. Some of the circles then slide out of the “hiding area,” and the child must determine how many of the circles are still hidden. | 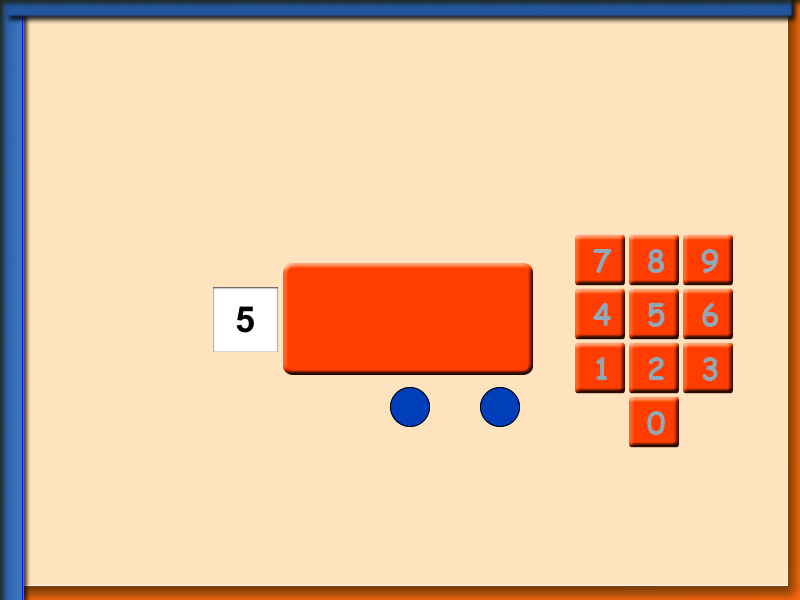 |
| Word Problems The Word Problems program randomly generates basic addition and subtraction “story problems” utilizing the various structures such problems can take (such as “Joining Result Unknown,” “Separating Start Unknown,” etc.). |  |
![]()